Confusion about parallel transport

[emily1986]

I am studying parallel transport in order to understand Berry curvature, but I know this topic is most commonly used in GR so I'm posting my question here. I do not know differential geometry. I am looking for a general explanation of what it means to parallel transport a vector.

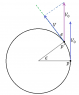
Mostly I am confused as to what is being held constant as you parallel transport a vector. I initially thought that the components of your transported vector were to be held constant with respect to a Cartesian coordinate system held at the surface of the curve. This coordinate system would always have the x-y axis forming a tangent plane on the surface, and the z axis always directed normal to the surface. But this couldn't be right, because the tangent plane is independent of path as long as the surface is smooth and differentiable. Then in this case, we would never get a different vector direction if we transported our vector around a closed path, which is obviously not true.

In other words, I would like to know what is wrong with my reasoning here: Parallel transport of a vector holds the components of the vector constant with respect to a plane tangent to the surface of the curved surface (and also to the axis normal to that surface). The plane tangent to a curved surface at a point will be the same regardless of the path you took to get to that point. Therefore any vector fixed with respect to this plane will also be the same. (which is incorrect)

Thank you for your help.

 

[Matterwave]

Are you working specifically with parallel transport of a vector along a 2-D surface embedded in 3-D Euclidean space?

 

[emily1986]

Yes, although I wouldn't mind understanding the general definition as well.

 

[Matterwave]

For a 2-D surface embedded in 3-D Euclidean space, the concept of parallel transport is much easier to visualize. Basically you have a vector on your 2-D surface, and since this vector lives in the tangent space of the 2-D surface, it also lives in the tangent space of the 3-D Euclidean space (which is itself). What you can do to parallel transport this vector around (this would be the Levi-Civita connection for your 2-D embedded surface) is to just have it move parallel to itself (in infinitesimal steps) in the 3-D Euclidean space (which is really simple since in Euclidean space parallel transport is trivial), and then simply project the resulting vector back onto the tangent space to the 2-D surface at the end of each infinitesimal step. This is probably the easiest case to visualize.

 

[emily1986]

When you say parallel transport keeps the vector parallel to itself, do you mean parallel in the traditional sense? In other words, are the components of the vector fixed in the 3-D Euclidian space? If this is the case, what would cause the vector to point in a different direction from which it started?

 

[A.T.]

When you say parallel transport keeps the vector parallel to itself, do you mean parallel in the traditional sense? In other words, are the components of the vector fixed in the 3-D Euclidian space? If this is the case, what would cause the vector to point in a different direction from which it started?

It stays parallel to itself locally over infinitesimally small steps along the path. Consider a sphere surface, which locally (in an infinitesimally small region) can be approximated by a plane. The vector’s projections on those local tangent planes stay parallel during infinitesimal steps. But along a global path they can accumulate a non-infinitesimal offset.

 

[emily1986]

So let's say that the vector we are transporting lies within the plane of the tangent plane. If we transported it along a closed path, would the vector remain inside the tangent plane or eventually pierce outside the plane?

 

[pervect]

When you say parallel transport keeps the vector parallel to itself, do you mean parallel in the traditional sense? In other words, are the components of the vector fixed in the 3-D Euclidian space? If this is the case, what would cause the vector to point in a different direction from which it started?

Yes, in the Euclidean geometry of the three-space, you just use the traditional parallel postulate. Or you can as you suggest use a cartesian coordinate system, and keep the components in the Cartesian coordinate system fixed.

What causes the vector to rotate is projecting it onto the 2d surface.

There's another way to define parallelism that works if you do not have torsion. I'll assume for the rest of the post that you don't have torsion. Matterweaves technique also assumed no torsion as this defines the Leva-Civita connection, and GR assumes no torsion. Your case as I understand it won't have torsion either.

This idea is a geometrical construction called Schild's ladder, see the wiki at http://en.wikipedia.org/w/index.php?title=Schild's_ladder&oldid=586460884

To use Schild's ladder for parallel transport you need to be able to draw curves that are the shortest curves between two (nearby) points on your curved surface, which are called (given the assumptions already made) geodesics.

You also need to be able to find the midpoint of a geodesic, and extend a geodesic (see the wiki article).

You can view Schild's ladder as a way to construct infinitesimal quadrilaterals whose opposite sides must by congruence have equal length, which makes them parallel.

 

[A.T.]

Is it correct to explain parallel transport the following way?

As long as the path follows a geodesic, the angle of the transported vector and the path's tangent is constant. When the path deviates from a geodesic in one direction, the transported vector changes it's angle to the path's tangent in the opposite direction by the same amount.

 

[Matterwave]

So let's say that the vector we are transporting lies within the plane of the tangent plane. If we transported it along a closed path, would the vector remain inside the tangent plane or eventually pierce outside the plane?

All vectors on the 2-D surface must live in the tangent plane. That's why, in the construction I showed you, you must project the vector down to the tangent space at each individual infinitesimal step. When you make a step, generally the vector won't remain in the tangent space anymore if you require that the vector is parallel to it self in the 3-D Euclidean space sense (i.e. yes, for when the Cartesian components of the vector remain constant), so you must project this resulting vector onto the tangent plane at the new location. It is this projection step that "changes the direction" of the vector as viewed from the 3-D Euclidean space. But because you MUST stay in the tangent space of the 2-D surface in order for the vector to be a valid vector, this is the best that you can do given the constraints. So we call this "parallel transport".

 

[emily1986]

It is this projection step that "changes the direction" of the vector as viewed from the 3-D Euclidean space.

Alright thank you for being patient with me, I think I'm starting to understand. From your explanation, it seems that the relative rotation of the tangent plane in 3-D space and the initial direction of the vector would be important. For instance, if we were to travel around the circumference of a sphere with our vector pointed in the direction of motion, and we projected our vector onto the tangent space after each step, since the tangent plane is only rotating in one direction (around the z axis if we are traveling along a line of latitude) then our traveling vector's projection would always stay directed towards the direction of motion, but its length would decrease to zero as the tangent plane neared 90 degrees with the vector's original orientation. What would the next step be? If we have a zero vector, then would there then be no projection after that point? Or do we normalize the vector after each step to hold its length constant?

 

[Matterwave]

Alright thank you for being patient with me, I think I'm starting to understand. From your explanation, it seems that the relative rotation of the tangent plane in 3-D space and the initial direction of the vector would be important. For instance, if we were to travel around the circumference of a sphere with our vector pointed in the direction of motion, and we projected our vector onto the tangent space after each step, since the tangent plane is only rotating in one direction (around the z axis if we are traveling along a line of latitude) then our traveling vector's projection would always stay directed towards the direction of motion, but its length would decrease to zero as the tangent plane neared 90 degrees with the vector's original orientation. What would the next step be? If we have a zero vector, then would there then be no projection after that point? Or do we normalize the vector after each step to hold its length constant?

The length wouldn't decrease to 0, it stays constant as you move it around the circumference. Remember that each step is infinitesimal. If you move the vector around the whole circumference, you will get the vector itself back. This is because you are moving the tangent vector to a geodesic along the same geodesic, and geodesics parallel transport their own tangent vectors.

 

[emily1986]

The length wouldn't decrease to 0, it stays constant as you move it around the circumference.

Wouldn't you have to normalize your vector at some point? If you keep projecting a vector on some surface that isn't in the plane of the vector, the projection length will be shorter than the original vector, correct?

 

[Matterwave]

Wouldn't you have to normalize your vector at some point? If you keep projecting a vector on some surface that isn't in the plane of the vector, the projection length will be shorter than the original vector, correct?

The way I've always thought about it was that since each step is infinitesimal, you aren't really making any difference to the length of the vector by projecting it. But I haven't thought about that too much, so maybe someone more knowledgeable can answer. :)

 

[A.T.]

The way I've always thought about it was that since each step is infinitesimal, you aren't really making any difference to the length of the vector by projecting it.

Parallel transport is usually defined to preserve magnitude.
http://www2.warwick.ac.uk/fac/sci/physics/current/teach/module_home/px436/notes/lecture10.pdf
http://physicspages.com/tag/parallel-transport/

I'm not sure if makes a difference whether you achieve this by projection in infinitesimal steps, or re-normalizing the vector after each step.

 

[Matterwave]

Parallel transport is usually defined to preserve magnitude.
http://www2.warwick.ac.uk/fac/sci/physics/current/teach/module_home/px436/notes/lecture10.pdf
http://physicspages.com/tag/parallel-transport/

I'm not sure if makes a difference whether you achieve this by projection in infinitesimal steps, or re-normalizing the vector after each step.

Ah, I recall now a discussion I read in Wald. He was talking about how a geodesic is defined such that $\nabla_\vec{u}\vec{u}=0$ and not $\nabla_\vec{u}\vec{u}=k\vec{u}$...i.e. how a geodesic is defined such that its tangent vector is parallel transported, with the magnitude staying the same, along itself and not with the vector's magnitude changing. I recall he mentioned that a re-parameterization of the geodesics will take us from the second case to the first.

I can't recall much more details than that. That's about all I can recall as far as the magnitude of parallel transported vectors are concerned.

 

[Nugatory]

Parallel transport is usually defined to preserve magnitude...
I'm not sure if makes a difference whether you achieve this by projection in infinitesimal steps, or re-normalizing the vector after each step.

I believe that you have to do it in infinitesimal steps. When you project a vector onto a plane, the components of the projection change by amounts proportional to the sine of the angle between the vector and the plane; the magnitude of the projection is proportional to the cosine.

$cos\theta = 1-\theta^2+...$ while $sin\theta=\theta+...$. An infinitesimal step in which we can drop all the terms in $\theta^2$ and higher preserves the magnitude while changing the coordinates appropriately.

 

[stevendaryl]

Mostly I am confused as to what is being held constant as you parallel transport a vector. I initially thought that the components of your transported vector were to be held constant with respect to a Cartesian coordinate system held at the surface of the curve. This coordinate system would always have the x-y axis forming a tangent plane on the surface, and the z axis always directed normal to the surface. But this couldn't be right, because the tangent plane is independent of path as long as the surface is smooth and differentiable. Then in this case, we would never get a different vector direction if we transported our vector around a closed path, which is obviously not true.

Actually, I think that insight is exactly right, except that the notion of a "Cartesian coordinate system" is only valid in an infinitesimal region.

Let's do it in 2D to make it easier to visualize. Suppose you're trying to do parallel transport on the surface of a sphere. What you can imagine doing is first approximating the sphere by a polygonal surface--a "Geodesic Dome" shape made up of lots of little triangles. Within each triangle, you can set up a Cartesian coordinate system, and parallel transport becomes trivial: You're just keeping the components of the vector constant as you move around. But now, if you parallel-transport a vector around a path that is big enough to include several triangles, then you have to worry about how you transport across a boundary of neighboring triangles. That's actually not that hard, either. You can imagine taking the two little triangles and flattening them to make a flat quadrilateral. You can come up with a Cartesian coordinate system for the quadrilateral, and use that to define parallel-transport across the boundary. So you know how to transport within a triangle and across the boundaries. So you can transport a vector along any path.

But here's the "catch": Parallel transport this way is only defined for a small collection of triangles. There is a limit to how many can be "flattened out" at once. So if you have two different paths to connect triangle A with triangle B, and those paths involve different triangles, then there is no guarantee that you will get the same answer from parallel-transport along the two paths.

 

[pervect]

I could look up the page number in Wald where he discusses why the length of a vector stays the same when parallel transported along a geodesic, but my recollection matches Matterweave's, and I suspect that people with the book can find it fairly easily, and people without the book won't find it that useful, (lacking both the book and the background in differential geometry that's needed to understand Wald's mathematics), so I don't currently see the point in looking it up. But if someone is really curious I'll do it.

I think it's sufficient to say at this point to just stress the end result, that parallel transport along a geodesic does not change the length of a vector. Also that Matterweave's scheme, when understood correctly, does not change the length of a vector.

I suppose at this point my main concern is that the OP has seen how to correctly understand Matterweave's scheme and why vectors don't change length when parallel transported.

A secondary concern is that the OP understands that the parallel postulate only applies to Euclidean geometries, so that we don't even expect Playfair's axiom (that there is only one line through an arbitrary given point parallel to a reference line) to work when we have non-Euclidean geometries.

[add]
Finally, if for some reason Matterweave's scheme isn't appealing, I'd like to point out again that in the absence of torsion (and I'm afraid I have no idea if Berry's phase has torsion or not :( ), there is a simple geometrical construction that one can use to parallel transport vectors known as "Schild's ladder".

 

[stevendaryl]

I could look up the page number in Wald where he discusses why the length of a vector stays the same when parallel transported along a geodesic, but my recollection matches Matterweave's, and I suspect that people with the book can find it fairly easily, and people without the book won't find it that useful, (lacking both the book and the background in differential geometry that's needed to understand Wald's mathematics), so I don't currently see the point in looking it up. But if someone is really curious I'll do it.

I have a little quibble about the claim that parallel transport preserves lengths. For one thing, parallel transport can be defined for manifolds that don't have a notion of "length" of a vector. For another, preserving lengths is not quite general enough, because there can be null vectors. So what, exactly, is preserved when you parallel-transport null vectors?

 

[emily1986]

This is because you are moving the tangent vector to a geodesic along the same geodesic, and geodesics parallel transport their own tangent vectors.

Just to make sure I understand, this means that we will never get a difference in phase as long as we travel along a geodesic?

cosθ=1−θ 2 +... cos\theta = 1-\theta^2+... while sinθ=θ+... sin\theta=\theta+... An infinitesimal step in which we can drop all the terms in θ 2 \theta^2 and higher preserves the magnitude while changing the coordinates appropriately.

If this is the correct way of thinking about this, then the change in phase of our vector is merely an accumulation of error? We ignore higher order terms, but over large distances they add up.

But here's the "catch": Parallel transport this way is only defined for a small collection of triangles. There is a limit to how many can be "flattened out" at once. So if you have two different paths to connect triangle A A with triangle B B, and those paths involve different triangles, then there is no guarantee that you will get the same answer from parallel-transport along the two paths.

Could you explain this further? What is the source of the difference between the two answers?

I suppose at this point my main concern is that the OP has seen how to correctly understand Matterweave's scheme and why vectors don't change length when parallel transported.

It seems similar to the adiabatic evolution of state vectors in quantum mechanics. The state vector will never change length, but acquires a phase. I'm not sure how to understand this geometrically though.

A secondary concern is that the OP understands that the parallel postulate only applies to Euclidean geometries, so that we don't even expect Playfair's axiom (that there is only one line through an arbitrary given point parallel to a reference line) to work when we have non-Euclidean geometries.

I do know this superficially, but I am still unclear about how 'parallel' is defined on a curved surface.

 

[Nugatory]

the change in phase of our vector is merely an accumulation of error?

I'd prefer to call it an accumulation of curvature effects.

 

[A.T.]

@emily1986
As long as you transport along a geodesic, the angle of the transported vector to the path tangent doesn't change. But of course a geodesic can intersect itself at any angle in general curved spaces, so a puerly geodesic parallel transport still can detect that curvature.

If your path on a trangulated surface crosses so many triangles, that you cannot flatten that crossed subset at once, then that subset has intrinsic curvature, which will be detected by parallel transport. To do the parallel transport you flaten just pairs of triangles, as you reach the edge between them.

 

[Matterwave]

I could look up the page number in Wald where he discusses why the length of a vector stays the same when parallel transported along a geodesic, but my recollection matches Matterweave's, and I suspect that people with the book can find it fairly easily, and people without the book won't find it that useful, (lacking both the book and the background in differential geometry that's needed to understand Wald's mathematics), so I don't currently see the point in looking it up. But if someone is really curious I'll do it.

I think it's sufficient to say at this point to just stress the end result, that parallel transport along a geodesic does not change the length of a vector. Also that Matterweave's scheme, when understood correctly, does not change the length of a vector.

I suppose at this point my main concern is that the OP has seen how to correctly understand Matterweave's scheme and why vectors don't change length when parallel transported.

A secondary concern is that the OP understands that the parallel postulate only applies to Euclidean geometries, so that we don't even expect Playfair's axiom (that there is only one line through an arbitrary given point parallel to a reference line) to work when we have non-Euclidean geometries.

[add]
Finally, if for some reason Matterweave's scheme isn't appealing, I'd like to point out again that in the absence of torsion (and I'm afraid I have no idea if Berry's phase has torsion or not :( ), there is a simple geometrical construction that one can use to parallel transport vectors known as "Schild's ladder".

Did you just call me Matterweave 4 times in one post? Matterwave yo...Matterwave. I don't wear a weave! :L

I also realized, after thinking for a bit, that this point by Wald was more about geodesics parallel transporting their own tangents (i.e. appropriately parameterized geodesics will parallel transport their own tangents, rather than simply "parallel transport + length manipulation" their own metrics) rather than about how parallel transport "preserves lengths". I think that given a metric connection, the "length" of a parallel transported vector will be preserved simply because the metric is connection-compatible. In other words we have $\nabla_\vec{u} \tilde{g}=0$ for all paths, so that the inner product between vectors is preserved by the connection, and therefore the length of vectors is preserved by the connection!

Sorry @emily1986, I got off on a tangent by mistake!

 

[Matterwave]

I have a little quibble about the claim that parallel transport preserves lengths. For one thing, parallel transport can be defined for manifolds that don't have a notion of "length" of a vector. For another, preserving lengths is not quite general enough, because there can be null vectors. So what, exactly, is preserved when you parallel-transport null vectors?

For null vectors, they have to remain null. See my post #19 with regards to the requirement of preserving lengths. Essentially, when a metric is present on a manifold, the connection must be metric-compatible, which means not just length preservation, but also angle preservation between two vectors as are they are parallel transported simultaneously along the same path.

 

[pervect]

Did you just call me Matterweave 4 times in one post? Matterwave yo...Matterwave. I don't wear a weave! :L

I did, Sorry about that. At least I'm consistent with my typos.

 

[stevendaryl]

For null vectors, they have to remain null.

That doesn't uniquely determine a null vector. If you have a vector A^\mu which is null, then any other vector of the form \lambda A^\mu is also going to be a null vector. So that criterion doesn't determine \lambda.

 

[Matterwave]

That doesn't uniquely determine a null vector. If you have a vector A^\mu which is null, then any other vector of the form \lambda A^\mu is also going to be a null vector. So that criterion doesn't determine \lambda.

Certainly. The requirement "preserves length" certainly does not uniquely determine the connection. I don' think...anyone tried to say that?

But the fundamental theorem of Riemann geometry (applies to pseudo-Riemannian manifolds as well) guarantees that the Levi-Civita connection is THE unique torsion-free connection which preserves the metric (not just lengths, but angles as well, as I mentioned).

http://en.wikipedia.org/wiki/Fundamental_theorem_of_Riemannian_geometry

 

[pervect]

I've been doing some skimming on "Berry phase" and related search terms. http://www.mpi-halle.mpg.de/mpi/publi/pdf/6325_05.pdf looks interesting. I'm not sure what to make of it yet, but I suspect that maybe the focus on lengths was confusing for a good reason. The closest I can come to a summary at this point is that the concern is related to transporting vectors representing a quantum system (I imagine the spin of a fermion for concreteness) rather than something classical. Anyway the Berry connection isn't the metric compatible one we are used to from GR.

 

[Nugatory]

I did, Sorry about that. At least I'm consistent with my typos.

You are an honest man. I'd be tempted to try blaming that sort of consistency on my spellchecker.