Outer measure .... Axler, Result 2.14 .... Another Question ....

[Math Amateur]

I am reading Sheldon Axler's book: Measure, Integration & Real Analysis ... and I am focused on Chapter 2: Measures ...

I need further help with the proof of Result 2.14 ...

Result 2.14 and its proof read as follows

In the above proof by Axler we read the following:

" ... ... To get started with the induction, note that 2.15 clearly implies 2.16 if \(\displaystyle n = 1\) ... "Can someone please demonstrate rigorously that 2.15 clearly implies 2.16 if \(\displaystyle n = 1\) ...

... in other words, demonstrate rigorously that \(\displaystyle [a, b] \subset I_1 \Longrightarrow l(I_1) \geq b - a\) ...
My thoughts ... we should be able to use \(\displaystyle (a, b) \subset [a, b]\) and the fact that if \(\displaystyle A \subset B\) then \(\displaystyle \mid A \mid \leq \mid B \mid\) ... ... ... ... but we may have to prove rigorously that \(\displaystyle \mid (a, b) \mid = b - a\) but how do we express this proof ...
Help will be much appreciated ... ...

Peter=============================================================================================================

Readers of the above post may be assisted by access to Axler's definition of the length of an open interval and his definition of outer measure ... so I am providing access to the relevant text ... as follows:

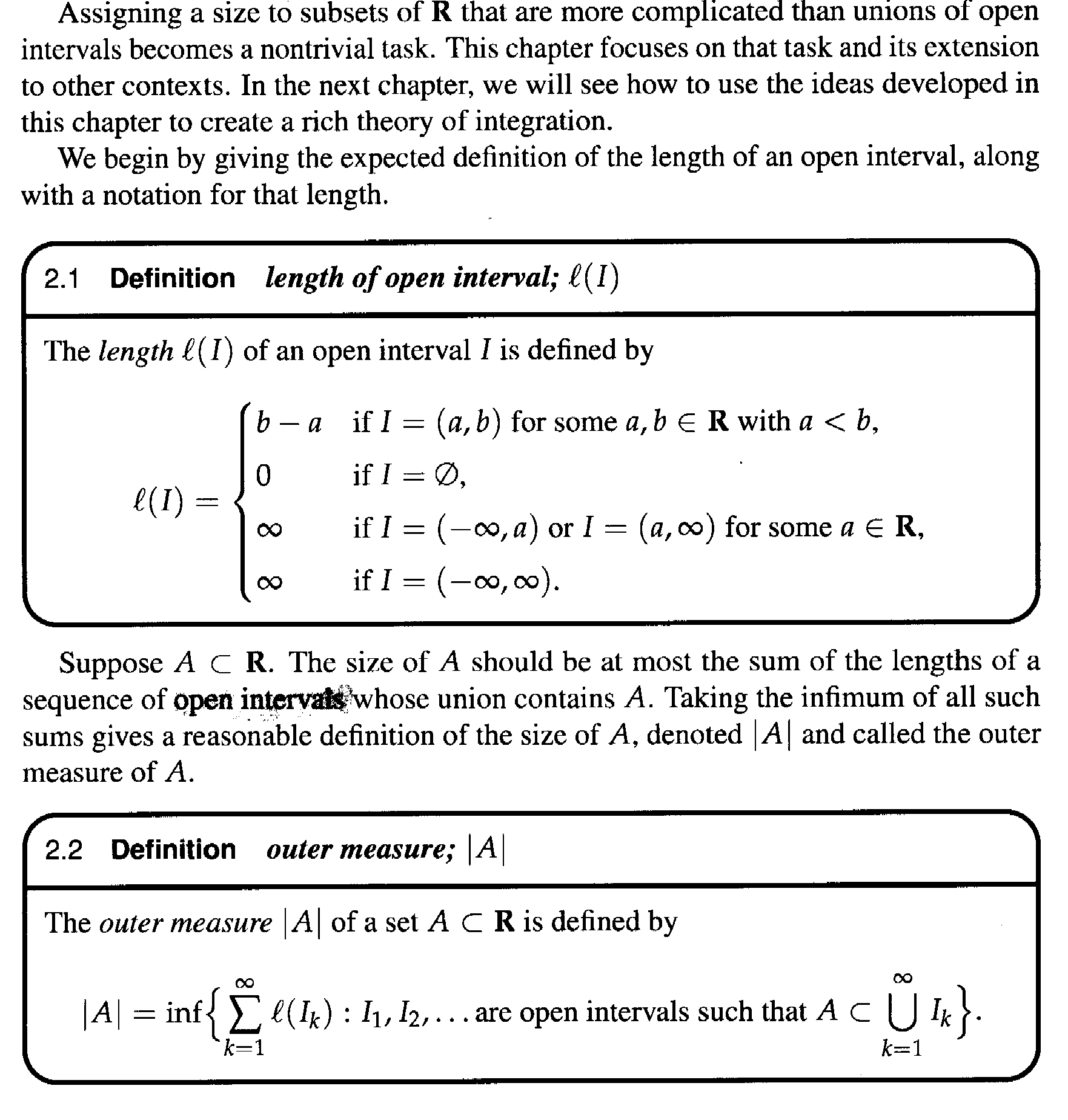
Hope that helps ...

Peter

 

[GJA]

Hi Peter,

Since $I_{1}$ is an open interval containing $[a,b]$, its right endpoint, say $c$, must be larger than $b$ (with infinity being allowed). Similarly, its left endpoint, say $d$, must be less than $a$ (with negative infinity being allowed). Can you proceed from here?

 

[Math Amateur]

Hi Peter,

Since $I_{1}$ is an open interval containing $[a,b]$, its right endpoint, say $c$, must be larger than $b$ (with infinity being allowed). Similarly, its left endpoint, say $d$, must be less than $a$ (with negative infinity being allowed). Can you proceed from here?

Thanks for the help GJA ... appreciate it ... I believe I can now proceed ...

Now ... we wish to show that \(\displaystyle [a, b] \subset I_1 \Longrightarrow l(I_1) \geq b - a\) ...

... so ... proceed as follows ...

Let \(\displaystyle I_1 = (c, d)\) where \(\displaystyle c \leq a \lt b \leq d\) ...

Then \(\displaystyle l(I_1) = d - c \geq b - a\) ...

Is that correct?

Peter

 

[GJA]

Looks good. Nicely done!