SHM r/R Ratio Question: Solving for Simple Harmonic Motion in a Fixed Trough"

[Saitama]

Homework Statement

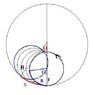
(see attachment for figure)
A small object is mounted to the perimeter of a hoop of radius r. The mass of the object and the hoop is same. The hoop is placed into a fixed semi-cylinder shaped rough trough of radius R, such that small mass is at top. Find the least r/R ratio such that the object performs simple harmonic motion.

Homework Equations

The Attempt at a Solution

I honestly have no idea on how should I begin to solve this problem, this is very different from the SHM problems I have done.

Any help is appreciated. Thanks!

 

[mfb]

After a rotation by an angle of θ, can you calculate the position of the mass and the circle? Can you calculate the potential energy?

 

[Saitama]

After a rotation by an angle of θ, can you calculate the position of the mass and the circle? Can you calculate the potential energy?

But then, how will I find the angle rotated by the small mass?

 

[mfb]

What do you mean with "the angle rotated by the small mass"?
Rotate the hoop by an angle θ in your trough, find the new positions of hoop and attached mass with geometry.

 

[Saitama]

What do you mean with "the angle rotated by the small mass"?
Rotate the hoop by an angle θ in your trough, find the new positions of hoop and attached mass with geometry.

(see attachment)
I am having trouble finding the new position of the attached mass. Is angle α=θ?

 

[mfb]

α=θ would imply that the mass always stays on top. In reality, the hoop rotates quicker.
Hmm, you use a different θ.

I'll switch to your angles:
After rolling a distance of d on the track, the contact position (on the hoop) changed by α=d/r. As seen from the center of the track, the hoop moved by an angle θ=d/R. Putting this together, the point mass is an angle of α-θ = d/r - d/R away from the top of the hoop now.

 

[Saitama]

α=θ would imply that the mass always stays on top. In reality, the hoop rotates quicker.
Hmm, you use a different θ.

I'll switch to your angles:
After rolling a distance of d on the track, the contact position (on the hoop) changed by α=d/r. As seen from the center of the track, the hoop moved by an angle θ=d/R. Putting this together, the point mass is an angle of α-θ = d/r - d/R away from the top of the hoop now.

Nice explanation mfb, thanks!

I still cannot find the potential energy of the system. (see attachment)
The blue dot represents the CM of hoop and the black dot represents the attached mass. How can I find h?

 

[mfb]

The blue dot has a fixed distance from the center of the track (which?), and you can use θ...

 

[Saitama]

The blue dot has a fixed distance from the center of the track (which?), and you can use θ...

R-r?

I worked on it to find h, is h=r+rcosθ?

 

[haruspex]

R-r?

I worked on it to find h, is h=r+rcosθ?

No, not quite.
It's easier to think in terms of how far the blue dot is below the centre of the hoop. It starts R-r below.

 

[Saitama]

No, not quite.
It's easier to think in terms of how far the blue dot is below the centre of the hoop. It starts R-r below.

I gave it a try again. This time, I got h=R(1-cosθ)+rcosθ. Is this right?

 

[haruspex]

I gave it a try again. This time, I got h=R(1-cosθ)+rcosθ. Is this right?

Yes.

 

[Saitama]

Yes.

What should be my next step?

 

[Saitama]

Can I get some more help?

 

[mfb]

Your next step should be to think about the problem. In addition, see post 2:

can you calculate the position of the mass and the circle? Can you calculate the potential energy?

 

[Saitama]

Your next step should be to think about the problem. In addition, see post 2:

Potential energy or change in potential energy?

 

[mfb]

Potential energy (as function of some angle), as I wrote.

An expression for the kinetical energy can be interesting, too, but I think it is not required for the answer.

 

[Saitama]

Potential energy (as function of some angle), as I wrote.

An expression for the kinetical energy can be interesting, too, but I think it is not required for the answer.

Potential energy assuming it is zero at the bottom of the trough is
U=mgh+mg(h+r\sin(\alpha-\theta))
U=2mgR(1-\cos \theta)+2mgr\cos \theta+mgr\cos(\alpha-\theta)
m is the mass of the hoop and the attached mass.

 

[haruspex]

Potential energy assuming it is zero at the bottom of the trough is
U=mgh+mg(h+r\sin(\alpha-\theta))
U=2mgR(1-\cos \theta)+2mgr\cos \theta+mgr\sin(\alpha-\theta)
m is the mass of the hoop and the attached mass.

Wouldn't r.sin(α-θ) represent a horizontal displacement?

 

[Saitama]

Wouldn't r.sin(α-θ) represent a horizontal displacement?

Oops, sorry about that.

What should I do next?

 

[ehild]

First express alpha with theta.

The potential function should have minimum at equilibrium. What is the condition that your U(θ) function has minimum at θ=0?

ehild

 

[Saitama]

First express alpha with theta.

How?

Do I need to use the relation posted by mfb: α-θ = d/r - d/R ?

 

[ehild]

What is d?

Think what rolling means. The point where the ball and cylinder touch each other covers equal lengths of arc both of the cylinder and ball. That gives a relation between alpha and theta.

Draw a big circle and cut out a small one, and try.

ehild

 

[Saitama]

What is d?

Rθ?

Think what rolling means. The point where the ball and cylinder touch each other covers equal lengths of arc both of the cylinder and ball. That gives a relation between alpha and theta.

Draw a big circle and cut out a small one, and try.

ehild

I am still clueless.

 

[haruspex]

Rθ?


I am still clueless.

If a ball radius r rolls along the ground through an angle theta about its own axis, how far has the point of contact with the ground travelled? If now that ground is itself curved up with some radius R>r, what does that distance along the circumference tell you about the angle the point of contact has traveled around the ground's centre of curvature?

 

[ehild]

Imagine that you paint the inside of a tube with a roller painter. You turn the roller once, till the same part touches the wall again. How long is the painted strip with respect to the circumference of the roller? And how long is it when you roll it only half .. or 3/4?

ehild

 

[Saitama]

Imagine that you paint the inside of a tube with a roller painter. You turn the roller once, till the same part touches the wall again. How long is the painted strip with respect to the circumference of the roller?

It is equal to the circumference of roller.

 

[ehild]

Yes. so if you turn the roller by angle alpha = 2pi the strip is s=(2pi) r long.
If you turn only halfway alpha =1/2*(2pi) the strip is s=pi*r long. If you turn by alpha the strip is s=... long.

But the length of the strip is also s=Rθ, so how are alpha and theta related?

ehild

 

[Saitama]

Yes. so if you turn the roller by angle alpha = 2pi the strip is s=(2pi) r long.
If you turn only halfway alpha =1/2*(2pi) the strip is s=pi*r long. If you turn by alpha the strip is s=... long.

But the length of the strip is also s=Rθ, so how are alpha and theta related?

ehild

s=αr, therefore α=Rθ/r. Should I plug this in the equation I wrote for Potential energy?

 

[ehild]

s=αr, therefore α=Rθ/r. Should I plug this in the equation I wrote for Potential energy?

Yes :)

ehild