Angular velocity Definition and 841 Threads
-

B What is physical significance of the direction of angular velocity?
This question has been bugging me for quite a while, That what do we mean by direction of angular velocity or torque. As we know that the direction of angular velocity or torque even is determined by right hand thumb rule, and it come out to be perpendicular to the rotational plane. So my... -
A
System of two wheels of different sizes with an axle through their centers
If I understand correctly : The angular velocity vector has two components: one along ##\text{-ve z-axis}## and one along ##\text{-ve x-axis} ## So the motion can be considered to be two rotations:(some animation might help) Rotation about ##\text{z-axis}## with angular speed...- Aurelius120
- Thread
- Angular momemtum Angular velocity Rigid body dynamics Rotational mecahnics Wheels
- Replies: 67
- Forum: Introductory Physics Homework Help
-
M
Horizontal component of the Coriolis force
The coriolis force that acts on the object moving on the Earth is: $$F_{cor}=2m(\vec v \times \vec \omega)$$##F_{cor}## is the Coriolis force, ##m## is the mass of the object, ## \vec{v}## is the velocity of the object in the Earth frame, and ## \vec{\omega}## is the angular velocity of the...- MatinSAR
- Thread
- Angular velocity Coriolis force
- Replies: 2
- Forum: Introductory Physics Homework Help
-
A
Comparison between two Tippe tops
I think that the second tippe top will spin on its stem first, and the first tippe top will stop spinning first due to its greater mass and lower angular velocity. Here are my ideas: They are given the same initial energy. By the conservation of energy principle, an object with greater mass...- abrahamabraham
- Thread
- Angular velocity Energy
- Replies: 1
- Forum: Introductory Physics Homework Help
-
D
Rotation matrix of three intrinsic rotations
I have three frames. The first is the fixed global frame. the second rotates an angle PHIZ with respect to the first. And the third first rotates a PHIX angle with respect to the x axis of the second frame, and then rotates a PHIY angle with respect to the last y axis. That is, there are a total...- dbeckam
- Thread
- Angular velocity Rotation matrix
- Replies: 3
- Forum: Mechanical Engineering
-
I
Investigation regarding the rotor ride
So far some of the topics I can think of are Investigating how angular velocity affects the centripetal force experienced by riders on the rotor or the coefficient of friction required to pin the rider to the wall.- Inaaya
- Thread
- Angular velocity Centripetal force Coefficient of friction
- Replies: 8
- Forum: Introductory Physics Homework Help
-
J
I Speed of Light Could be Reached and Indeed even Exceeded
If I mount a fast spinning motor - let's say a 250k rpm, inside a cylinder, and use this cylinder as the armature of the second cylinder that sits round the first one and has the same rpm with the angular velocity in the same direction as the first one, and continue building these layers of fast...- James1355
- Thread
- Angular velocity Speed of light
- Replies: 21
- Forum: Special and General Relativity
-

Brownian Ratchet (exercise framed by Feynman's Lectures, l. 46)
1. (d) Both clockwise and counterclockwise rotation, with no net direction. Because the pawl, which is also at the same temperature as the paddle and the ratchet, will undergo Brownian motion and bounce up and down randomly. Sometimes, it will fail to catch the ratchet teeth and allow the...- Hak
- Thread
- Angular velocity
- Replies: 32
- Forum: Introductory Physics Homework Help
-

Angular Velocity of a Large Pendulum on Earth as seen from the stars
I don't understand the question. how am I supposed to find the magnitudes and directions of the velocity from the figure?- Asem
- Thread
- Angular Angular velocity Earth Pendulum Stars Velocity
- Replies: 11
- Forum: Introductory Physics Homework Help
-

I Angular velocity of a rod and what formula to use while solving.
The question is: A uniform rod of length ##L## stands vertically upright on a smooth floor in a position of unstable equilibrium. The rod is then given a small displacement at the top and tips over. What is the rod's angular velocity when it makes an angle of 30 degrees with the floor, assuming...- brochesspro
- Thread
- Angular Angular velocity Formula Rod Rotaional kinematics Rotating bodies Velocity
- Replies: 15
- Forum: Mechanics
-
A
Question involving clockwise angular movement
v=1.00*8=>v=8 rad/s ar=>100*8^2=>ar=64 rad/s^2 at=1.00*4=>at=4 rad/s^2 The only question I have is ar=-64 rad/s^2, not 64 rad/s^2 as I calculated. I believe this is because the wheel is accelerating in a clockwise direction. However this is not indicated by the mathematical equation. How do I...- as2528
- Thread
- Angular Angular acceleration Angular velocity Movement
- Replies: 9
- Forum: Introductory Physics Homework Help
-
A
Question involving angular acceleration of a spinning wheel
wfinal=98.0 rad/s, dt=3.00s w=(37 revs/3)=>w=(37 revs*(2*pi/1))/3=>w=77.493 a=(98-77.493)/3=>a=6.8357 My answer is exactly half of the correct answer. Where did I go wrong?- as2528
- Thread
- Acceleration Angular Angular acceleration Angular speed Angular velocity Spinning Wheel
- Replies: 12
- Forum: Introductory Physics Homework Help
-

Change in Angular Velocity While Orbiting With No Torque
The planet is faster when it is closer to the planet because when it is closer to the planet it has less rotational inertia, and rotational momentum is conserved in this system, so less rotational inertia means a greater angular velocity. This explains why it is slower when it is farther away...- uSee2
- Thread
- Angular Angular velocity Ap physics 1 Change Torque Velocity
- Replies: 30
- Forum: Introductory Physics Homework Help
-
Z
Angular velocity of an airplane
Speed = 900km/hour tan(α)=900t/10000 α=arctan(900t/10000) Derivative is 900/(10000+81 t^2)- Zoubayr
- Thread
- Airplane Angular Angular velocity Velocity
- Replies: 18
- Forum: Introductory Physics Homework Help
-
D
I Can a Satellite Maintain its Angular Velocity with Continuous Low Thrust?
Suppose two satellites are in a circular heliocentric orbit with radius R and with angular velocity O'. Satellite 2 then undergoes a low continuous thrust. Can Satellite 2 (the one that undergoes the continuous low thrust) maintain the same angular velocity O' about the sun? It seems that...- dansmith170
- Thread
- Angular Angular velocity Continuous Orbital mechanics Velocity
- Replies: 2
- Forum: Astronomy and Astrophysics
-
A
Angular Velocity from KE, radius, and mass
I tried using the equation w^2 = (4*K)/(mr^2) but I don't think this is right... I got my answer to be 3.2243 and that's not correct- aivilo775
- Thread
- Angular Angular velocity Mass Radius Velocity
- Replies: 4
- Forum: Introductory Physics Homework Help
-
R
I Changes to a spinning skater's angular velocity
Can we describe what is happening as the skater's angular velocity increases/decreases using F=ma rather than invoking L = constant?- Rosenthal
- Thread
- Angular Angular velocity Spinning Velocity
- Replies: 4
- Forum: Classical Physics
-

Angular Velocity in the Rotating systems
Summary: Consider a body which is rotating with constant angular velocity ω about some axis passing through the origin. Assume the origin is fixed, and that we are sitting in a fixed coordinate system ##O_{xyz}## If ##\rho## is a vector of constant magnitude and constant direction in the...- WMDhamnekar
- Thread
- Angular Angular velocity Rotating Systems Vector calculus Velocity
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-

Calculating final rotational speed from angular velocity
I have no idea how to go about this. Any help would be appreciated thanks :) Edit: I converted the 1.5 rev/s to rad/s = 9.4 rad/s- Anmol Dubey
- Thread
- Angular Angular velocity Final Momentum No idea Physics Rotational rotational speed Speed Thanks Velocity
- Replies: 6
- Forum: Introductory Physics Homework Help
-
D
I Solving for Angular Velocity After a Disc Collision
Hello to everyone, first of all shame on me I has to ask and can not figure out it by myself... The problem is I am trying to code game where two homogenous discs with same mass and same diameter, no fricition due to gravitational forces, can collide. I can figure out the speed and direction...- daavid
- Thread
- Angular Angular velocity Collision Collison Disc Velocity
- Replies: 13
- Forum: Classical Physics
-

I Angular Velocity: Vector or Not?
I understand that angular velocity is technically not a vector so does that mean the cross product of the radius vector and the angular velocity vector, the tangential vector, is also not a vector?- e2m2a
- Thread
- Angular Angular velocity Vector Velocity
- Replies: 5
- Forum: Classical Physics
-
D
Is the Angular Velocity of Pinned Rods Calculated Correctly?
My line of thinking is as follows: \omega_{PQ} = \frac{v_{\perp}}{\ell} = \frac v\ell \frac{\sqrt3}{2} Similarly for rod ##QR## \omega_{QR} = \frac{v_{\perp}}{\ell} = \frac v\ell \frac{\sqrt3}{2} Is my reasoning correct?- DrNG
- Thread
- Angular Angular velocity Rod Rotational kinematics Velocity
- Replies: 5
- Forum: Introductory Physics Homework Help
-
L
Calculate the angular velocity for each case
First case, descends with the wheel: mgh = .5(I)(w^2) ———- GPE converted to wheel energy w = .1095. ———- rotation result is .1095 Second case, allow to free fall and impulse: mgh = .5(m)(v^2). ———- GPE converted to kinetic energy v = 7.746 ———-...- leafy
- Thread
- Angular Angular velocity Velocity
- Replies: 5
- Forum: Introductory Physics Homework Help
-
P
Angular velocity of rod and bug
I(i)w(i)= I(f)w(f) I(i)= 1.08 x 10-3 kg·m2 w(i)= 0.221 rad/s I(f)= mr^2 + I(i) = (5 x 10^-3)(.138)^2 + (1.08 x 10^-3) (1.08 x 10-3)(.221) = ((1.08 x 10^-3)+9.22 x 10^-5))w(f) w(f) = (2.3868 x 10^-4)/(0.00117522) w(f)= 0.203094 rad/s This is my attempt; however, I cannot seem to get it...- p37
- Thread
- Angular Angular velocity Bug Rod Velocity
- Replies: 6
- Forum: Introductory Physics Homework Help
-
T
Angular Velocity: Pulley and belt system
So far I have: The velocity of the belt will be the same for pully A and D, so we can calculate the angular velocity of pulley D: ## V_A = V_B ## ## \omega_A r_A = \omega_D r_D ## ## ((20*3)+40)(0.075) = \omega_D (0.025) ## ## \omega_D = 300 Rad/s ## My next step was to determine the angular...- thatstheguy9
- Thread
- Angular Angular velocity Belt Pulley System Velocity
- Replies: 11
- Forum: Introductory Physics Homework Help
-
N
Engineering Find the velocity and angular velocity of the rod and point
Hi everyone :)! I resolve this problem with components method and trigonometry method. My results with components method its okay, but i can´t obtain the correct VE velocity. Im sure that the problem its in the angles, but i don't know how to fix it. The correct answers: -Angular velocity...- Noob of the Maths
- Thread
- Angular Angular velocity Point Rod Velocity
- Replies: 10
- Forum: Engineering and Comp Sci Homework Help
-
N
Find the angular velocity of this linkage
Hi! everyone! ;) I have a problem with the development of this problem. I need to resolve it with 2 procedures: trigonometry and instant centers. My advance can be see in the next image: The instant centers procediment its (1) up and trigonometry procediment its (2) down. I know that the...- Noob of the Maths
- Thread
- Angular Angular velocity Linkage Velocity
- Replies: 8
- Forum: Precalculus Mathematics Homework Help
-

I Apparent angular velocity (inclined orbit)?
Here's the problem setup, my student and I are stuck. A disk is rotating at constant angular velocity ω, and we are watching a point on the rim, parameterized by the angular position θ, move. Because we are observing the motion from an inclination angle Ψ, we do not always observe the...- Andy Resnick
- Thread
- Angular Angular velocity Orbit Velocity
- Replies: 11
- Forum: General Math
-
B
Existential dilemma on angular velocity of a complex rigid body
I assumed the angular velocity of the center of mass of the two discs about z axis to be w1 note that angular velocity of center of mass of both discs and center of anyone disc about z axis is same, you can verify that if you want, me after verifying it will use it to decrease the length of the...- bhupesh
- Thread
- Angular Angular velocity Body Complex Rigid body Velocity
- Replies: 25
- Forum: Introductory Physics Homework Help
-
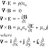
Measure angular velocity and acceleration from missing tooth wheel
Hi, I need to come up with a math model for a digital ignition system. I've been thinking about it and I think that I need to measure 2 things to be able to calculate when I have to start charging the coil. They are the angular velocity and the acceleration but how can I do it? the idea is to... -

Terminal angular velocity of Disc in magnetic field
Torque appiled by smaller disc = mga emf of disc due to B = Bwr2/2 Current I = Bwr2/2R force = IBr = Bwr3/2r torque = rF = Bwr4/2r mga = Bwr4/2r- Advay
- Thread
- Angular Angular velocity Disc Field Magnetic Magnetic field Velocity
- Replies: 1
- Forum: Introductory Physics Homework Help
-
V
How to determine angular velocity about a certain axis?
If the crawling insect were stationary at a certain instant of time, then it would have the same angular velocity as that of disk, which is w in a clockwise direction. But now it's velocity at any instant is the vector sum of velocity due to rotation and the velocity it crawls at. My attempt is...- vcsharp2003
- Thread
- Angular Angular velocity Axis Velocity
- Replies: 10
- Forum: Introductory Physics Homework Help
-

Finding Angular Velocity in Rotational Motion Problems
53 rpm equals 5.55 rad/sec multiply 5.55 by 2pi to get angular velocity of 34.8717 Is the answer 34.8717? What should I have done to more accurately solve the problem with a better understanding? What other steps should I take when solving similar problems? and lastly, Is the mass relevant...- momoneedsphysicshelp
- Thread
- Angular Angular velocity Intro physics Motion Physics Rotational Rotational motion Velocity
- Replies: 5
- Forum: Introductory Physics Homework Help
-

Engineering Solving for Angular Velocity at Point A - Confused!
So for this question I got the right angular velocity. But I don’t get the same velocity for point A. I don’t understand why it’s cos30, problem asked for V_a when theta = 45 so I used cos45. I have my work below.- Pipsqueakalchemist
- Thread
- Angular Angular velocity Confused Point Velocity
- Replies: 1
- Forum: Engineering and Comp Sci Homework Help
-

Direction of Angular velocity and Angular momentum?
I am very confused when textbooks say the direction of Angular velocity is perpendicular ot radius and theta for that matter direction is in perpendicular direction. I know this comes from cross product rule but what is the meaning of Angular velocity and Angular momentum directing in upward...- akashpandey
- Thread
- Angular Angular momentum Angular velocity Direction Momentum Velocity
- Replies: 9
- Forum: Mechanics
-

I What is the angular velocity of a satellite?
Angular velocity ω is by definition the runned angle dθ per time dt elapsed: ω=dθ/dt. If the time elapsed in the center of the Earth is dt, the dilated time elapsed on satellite is dt′. What is the satellite's angular velocity? Is it dθ/dt or dθ/dt′?- binis
- Thread
- Angular Angular velocity Satellite Velocity
- Replies: 132
- Forum: Special and General Relativity
-
H
What is the final angular velocity of the system after the collision?
I calculated as attached and got it right. However, I just wonder why we can't use conservation of energy as the question has already specified 'frictionless', meaning no energy loss and energy distributed to the rotation only.- hidemi
- Thread
- Angular Angular velocity Collision Final System Velocity
- Replies: 4
- Forum: Introductory Physics Homework Help
-

Angular velocity of a weighted rod left free to rotate around a pivot
Hi guys, I don't really know how to solve this problem. The point is finding ##\omega## when ##m_2## passes from ##m_1##'s original position. Ideally, I'm thinking about some conservation of energy/momentum to apply here, but I'm quite confused. Any hint?- greg_rack
- Thread
- Angular Angular velocity Pivot Rod Rotate Velocity
- Replies: 6
- Forum: Introductory Physics Homework Help
-

Getting the angular velocity using the angular acceleration graph
The answer here is A What i did is getting the area as follows, 2×4×1/2 +3×-6×1/2 +4×-6 = -29 and then use this Δω=ωf-ωi -29=ωf-5 ωf=24 but there is no such choice.- dahoom102
- Thread
- Acceleration Angels Angular Angular acceleration Angular velocity Graph Rotaional kinematics Velocity
- Replies: 3
- Forum: Introductory Physics Homework Help
-
A
Engineering How to find the angular velocity of a rotating drum?
Good day here is the exerciceThe only velocity I do have is the velocity v os the center of pulley 5, I tried to find the center of instantaneous velocity to find the angular velocity of pulley 5 but I couldn't, any hint would be highly appreciated! Best regards!- Amaelle
- Thread
- Angular Angular velocity Drum Rotating Velocity
- Replies: 7
- Forum: Engineering and Comp Sci Homework Help
-

Sum of oscillating frequency and angular velocity
The "egg" initially spun around axis 1 with at ##\omega_s##. After being disturbed, it has started to possesses angular velocities along 2 and 3. The question is to find the rotational speed of ##\vec \omega=\vec\omega_1+\vec\omega_2+\vec\omega_3## to a fixed observer. It is calculated that...- Leo Liu
- Thread
- Angular Angular velocity Frequency Oscillating Sum Velocity
- Replies: 11
- Forum: Introductory Physics Homework Help
-
M
Help with Angular Velocity True-False Question
1)Starting at rest, he brings the weights into his chest. His angular velocity increases. 2)A friend throws a third weight so that the student catches it in one of his outstretched hands. No matter what the direction of the throw, the student's angular velocity decreases. 3) Starting with...- MattAstros
- Thread
- Angular Angular velocity Velocity
- Replies: 12
- Forum: Introductory Physics Homework Help
-
A
Angular velocity and angular acceleration of a turbine
Okay so what I've done; I've put the diammter d = 1m as r = 1m The time interval of 4s is t = 4s and the angular velocitys as; ω1 = 20 rad/s ω2 = 40 rad/s Now to get the accelaration. Angular acceleration can be split into two parts tangetial acceleration and radial acceleration What I...- arhzz
- Thread
- Acceleration Angular Angular acceleration Angular velocity Turbine Velocity
- Replies: 17
- Forum: Introductory Physics Homework Help
-
A
Angular velocity in terms of Euler angles
In Chapter 4, derivation 15 of Goldstein reads: "Show that the components of the angular velocity along the space set of axes are given in terms of the Euler angles by $$\omega_x = \dot{\theta} \cos \phi + \dot{\psi} \sin \theta \sin \phi, \omega_y = \dot{\theta} \sin \phi - \dot{\psi} \sin... -
E
Orbital and spin angular velocity?
The Wikipedia page for angular velocity makes a big fuss over "spin" and "orbital" angular velocities, but I have checked through Gregory and Morin's textbooks on classical mechanics and haven't found any reference to them at all. They just work with a single quantity, the angular velocity...- etotheipi
- Thread
- Angular Angular velocity Orbital Spin Velocity
- Replies: 17
- Forum: Mechanics
-
G
What is a "Torsional Constant"?
The question was: I will also include the solution: So, what is the justification of the first formula [ω=√(C/I)]? I know how to derive simple harmonic equations, this one as I guess is probably similar? But I cannot connect as to how C is used exactly. And the second formula [ω'=ωβ], I...- Gourab_chill
- Thread
- Angular velocity Constant Moment of inertia Rotational motion
- Replies: 11
- Forum: Introductory Physics Homework Help
-

Require help with angular velocity and people flying off the planet
My solutions (attempts) : a> w=v/r | r=6.35x10^6m | therefore V=7.04x10^-5 m/s b> speed of rotation is faster at the equator than the pole as w=v/r. As w remains constant, as r increases towards the pole V has to decrease. c> F = W - R d> Stuck here. I presume that I have to use the equation...- RemotePhysics
- Thread
- Angular Angular velocity Circular motion Earth Gravity Planet Velocity
- Replies: 26
- Forum: Introductory Physics Homework Help
-

How Does Resolving Angular Velocity into Components Affect Its Magnitude?
Angular velocity is the degrees by which something rotates over a time period. If I have an angular velocity in one direction and I resolve it into its components, its components would obviously be of lesser value. Here's what I don't get. When I imagine this scenario, I see that the thing...- aspodkfpo
- Thread
- Angular Angular velocity Confused Velocity
- Replies: 27
- Forum: Introductory Physics Homework Help
-
G
Mechanics: Angular Velocity Vector Questions
Answers are the following : (i) v=(2cost)i - (2sint)j -(1/2)k (ii)2.06m/s (iii)2m/s^2 horizontally towards the vertical axis, making an angle of pi/4 with both the I and j axes.- girlwhoneedsmathhelp
- Thread
- Angular Angular velocity Mechanics Vector Velocity Velocity vector
- Replies: 6
- Forum: Introductory Physics Homework Help
-

Vector potential ##\vec A## in terms of magnetic field ##\vec B##
My solution is making an analogy of the ##\text{Relevant equations}## as shown above, starting from the equation ##\vec \omega = \frac{1}{2} \vec \nabla \times \vec v##. We have ##\vec B = \vec \nabla \times \vec A = \frac{1}{2} \vec \nabla \times 2\vec A \Rightarrow 2\vec A = \vec B \times...- brotherbobby
- Thread
- Angular velocity Curl Field Magnetic Magnetic field Magnetic vector potential Position vector Potential Terms Vector Vector potential
- Replies: 26
- Forum: Advanced Physics Homework Help