Brian Tsai
- 1
- 1
- TL;DR Summary
- Why the surface integral is 0 even the J itself extends to infinity (as in the case of an infinite straight wire).
I am recently reading "Introduction to Electrodynamics, Forth Edition, David J. Griffiths " and have a problem with the derive of the curl of a magnetic field from Biot-Savart law. The images of pages (p.232~p233) are in the following:

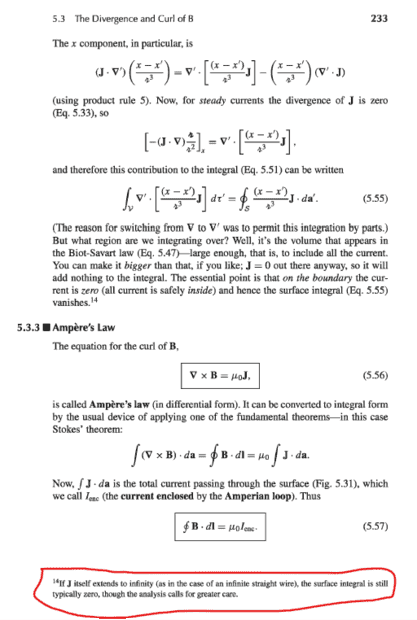
The second term in 5.55(page 233) is 0. I had known the reason in case of that the current density declined to 0 on the surface. My question is how to prove the surface integral will also be 0 when J extends to infinite(red block).
P.S. : This is my first time asking a question in English, and I had done my best to decrease the improper use of English. I sincerely hope that anyone who notices my post can answer my confusion and don't be mad at my terrible use in English
The second term in 5.55(page 233) is 0. I had known the reason in case of that the current density declined to 0 on the surface. My question is how to prove the surface integral will also be 0 when J extends to infinite(red block).
P.S. : This is my first time asking a question in English, and I had done my best to decrease the improper use of English. I sincerely hope that anyone who notices my post can answer my confusion and don't be mad at my terrible use in English