A. P. French "Matter and Radiation: The Inertia of Energy"

[Math Amateur]

I am reading A. P. French's book: "Special Relativity". Currently I am focused on the section: "Matter and Radiation: The Inertia of Energy."

Under the heading: "Matter and Radiation: The Inertia of Energy", French writes the following:

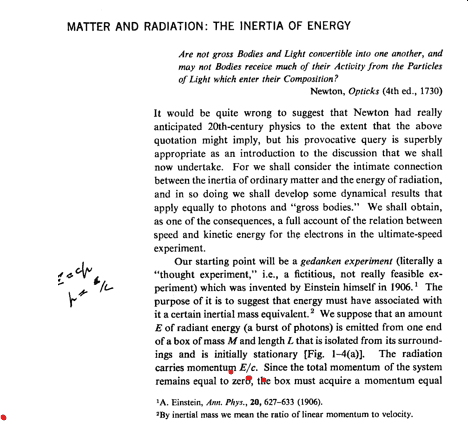
In the above text by Young we read the following:

" ... ... But this being an isolated system, we are reluctant to believe that the center of mass in the box plus its contents have moved. We therefore postulate that the radiation has carried with it the equivalent of a mass m , such that

mL + M(delta)x = 0 ... ... ... 1-7

... ... "


Can someone please explain how Young formulates equation 1-7 ... how does he arrive at this equation?

Peter

 

[PeroK]

What specifically is not clear? Once you get the idea that light has momentum and that the centre of mass should not move, the kinematics are quite straightforward, are they not?

 

[Ibix]

1-7 is just requiring that the center of mass doesn't move. A mass $m$ has moved one distance and a mass $M$ has moved another, but $\sum m_ix_i$ has not changed.

Note that this argument is slightly handwaving because the light pulse moves $L-\Delta x$, so he's quietly neglected a term like $m\Delta x$ as being very small.

 

[Math Amateur]

What specifically is not clear? Once you get the idea that light has momentum and that the centre of mass should not move, the kinematics are quite straightforward, are they not?

Well, I was having some difficulty proving 1-7 ... BUT ... I note that Young writes that 1-7 is a postulate or assumption ... so we do not have to prove it ... and ... if you assume 1-7 to be true then 1-8 follows by simple algebra ...

Peter

 

[Math Amateur]

1-7 is just requiring that the center of mass doesn't move. A mass $m$ has moved one distance and a mass $M$ has moved another, but $\sum m_ix_i$ has not changed.

Note that this argument is slightly handwaving because the light pulse moves $L-\Delta x$, so he's quietly neglected a term like $m\Delta x$ as being very small.

I note that Young writes that 1-7 is a postulate or assumption ... so we do not have to prove it ... and ... if you assume 1-7 to be true then 1-8 follows by simple algebra ...

Thanks again for your help ...

Peter