B Algebraic Operations on Energy-Momentum Relationships

[alan123hk]

This is just basic algebra for the energy-momentum relationship, but the calculations confuse me. May I ask what is wrong with my concept or calculation causing the following problem.

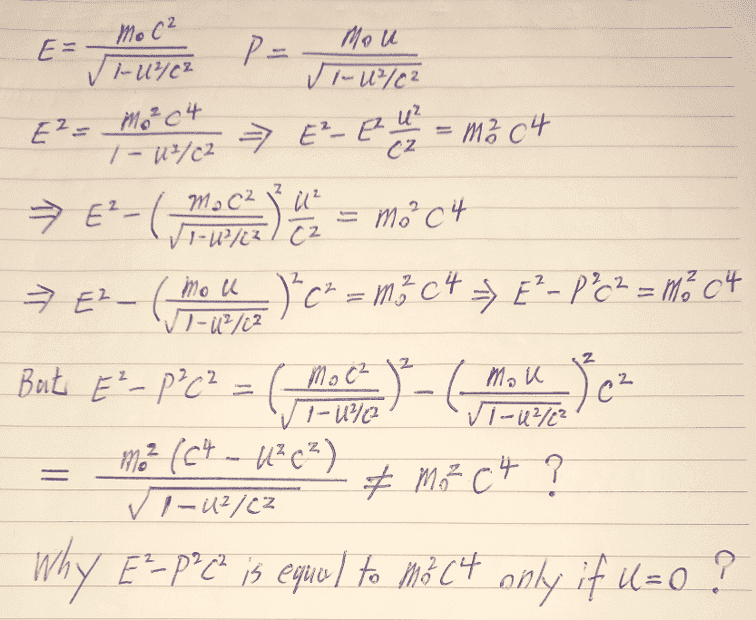
Maybe it's because I'm getting older, my ability to think and calculate has declined...

 

[weirdoguy]

Second line counting from the bottom - the square root should not be there since you raised it to the second power in the third line from the bottom.

 

[alan123hk]

Third line counting from the bottom - the square root should not be there since you raised it to the second power.

My goodness!
got it, thanks.

 

[PeroK]

Simpler is
$$E^2 - p^2 c^2 = \gamma^2m^2c^4(1 - \frac{v^2}{c^2})$$And the result follows immediately as the expression in brackets equals $\frac 1 {\gamma^2}$