- #1
Fjolvar
- 156
- 0
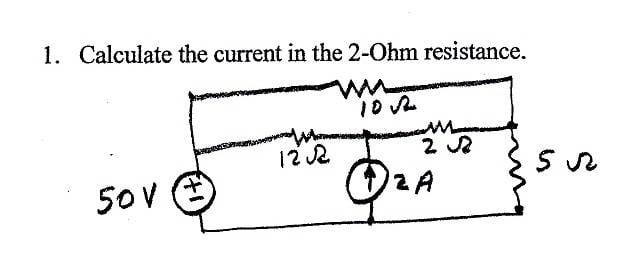
I've only taken a basic circuits course and that was years ago. Can anyone help me get this one started?
1. Homework Statement
KCL
[/B]
I'm not sure if I should use KCL right away or use Thevenin.
Since I need to find the current through the 2 ohm resistor, I can't combine the 10 ohm resistor with the 12 & 2 ohm resistor.
Also I'm not sure how the 2A current source would distribute through the circuit. Would the full 2A would flow through the 2 ohm resistor?
Any help would be much appreciated.
1. Homework Statement
Homework Equations
KCL
The Attempt at a Solution
[/B]
I'm not sure if I should use KCL right away or use Thevenin.
Since I need to find the current through the 2 ohm resistor, I can't combine the 10 ohm resistor with the 12 & 2 ohm resistor.
Also I'm not sure how the 2A current source would distribute through the circuit. Would the full 2A would flow through the 2 ohm resistor?
Any help would be much appreciated.