Approximating Pi to Different Digits

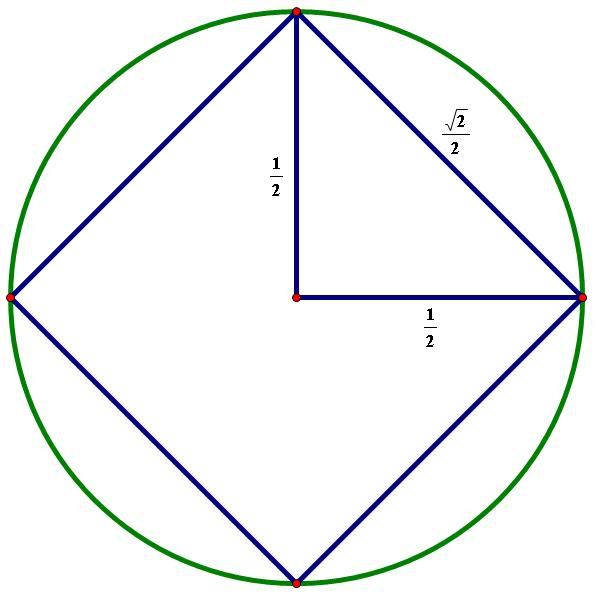
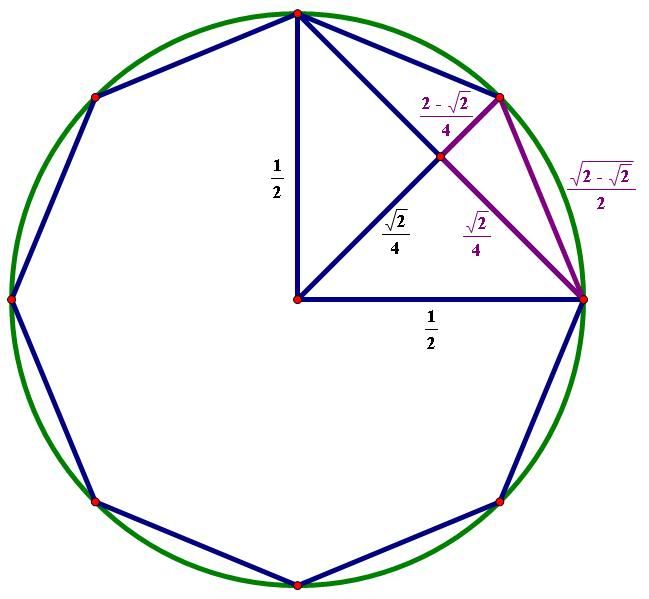
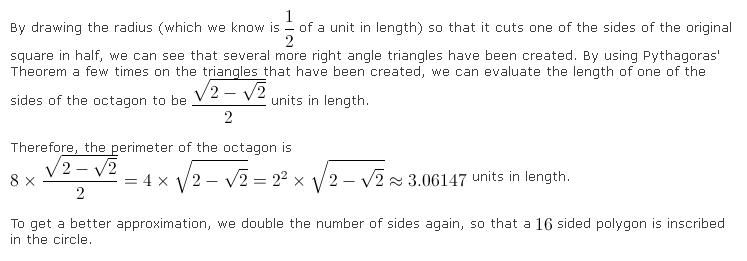
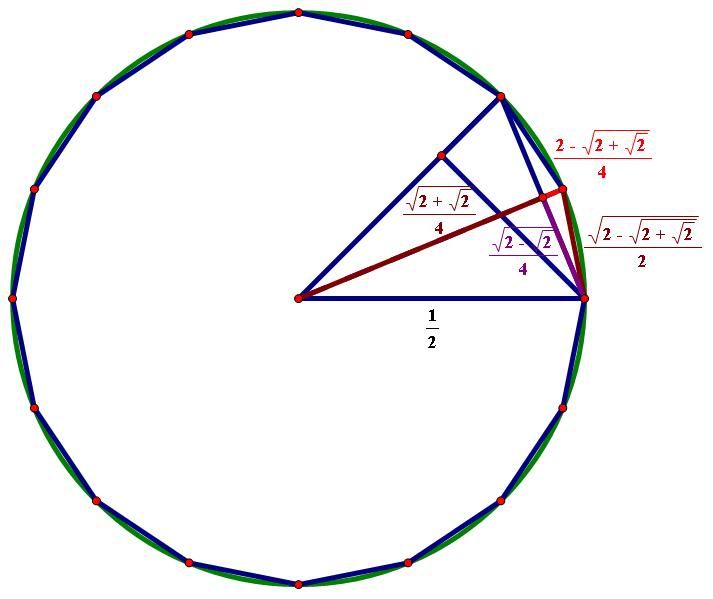
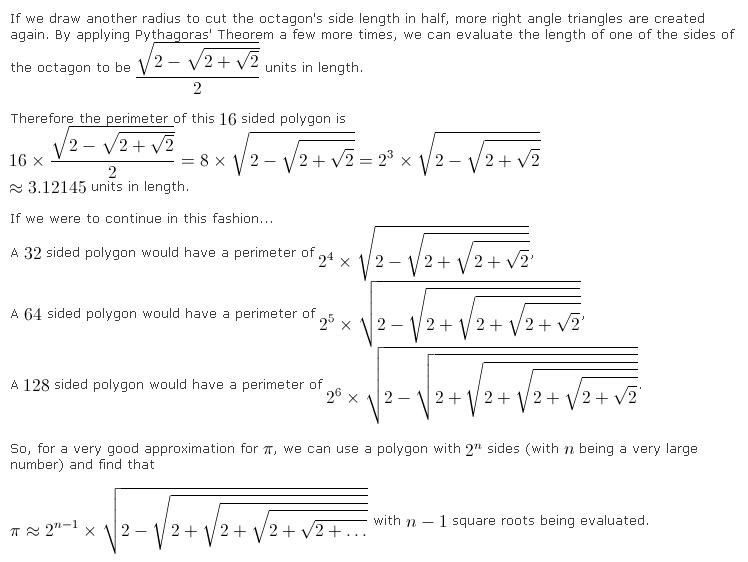
[soroban]

Watch this . . .\pi \;=\;3.141592645

. . = \;3 + 0.141592654 \;=\; 3 + \dfrac{1}{7.062573306} . [1]

. . =\;3 + \dfrac{1}{7 + 0.062573306} \;=\; 3 + \frac{1}{7+ \dfrac{1}{15.99659441}} .[2]

. . =\;3 + \dfrac{1}{7 + \dfrac{1}{15 + 0.99659441}} \;=\; 3 + \dfrac{1}{7 + \dfrac{1}{15 + \dfrac{1}{1.003417228}}}

. . [=\;3 + \dfrac{1}{7 + \dfrac{1}{15 + \dfrac{1}{1 + 0.003417228}}} \;=\;3 + \dfrac{1}{7 + \dfrac{1}{15 + \dfrac{1}{1 + \dfrac{1}{292.6348491}}}} .[3]If we stop at [1]: .\pi \;\approx\;3+\frac{1}{7} \;=\;\frac{22}{7} \;=\;3.142857...

If we stop at [2]: .\pi\;\approx\;3 + \frac{1}{7 + \dfrac{1}{16}} \;=\;\frac{355}{113} \;=\;3.14159292...

If we stop at [3]: .\pi \;\approx\;3 + \frac{1}{7+\dfrac{1}{15+\dfrac{1}{1 + \dfrac{1}{293}}}} \;=\;\frac{104,\!348}{33,\!215} \;=\;3.141592654...

 

[Prove It]

This was part of a series of lessons I did at a Harry Potter site, so disregard the first few sentences :P