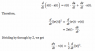- #1
Are a vector and its derivative perpendicular at all times?
- Thread starter TheCanadian
- Start date
In summary, the derivative of a vector has components both normal and tangent to the vector, and for an obvious example, consider a vector whose magnitude but not direction is changing as a function of time. In this case, the derivative must be perpendicular if the magnitude is constant over time, as in the case of acceleration in uniform circular motion.
- #2
- 23,583
- 5,819
No. What if the vector is not changing direction? In general, the derivative of a vector has components both normal and tangent to the vector.
Chet
Chet
- #3
Nugatory
Mentor
- 15,120
- 9,888
TheCanadian said:
No. For an obvious example, consider a vector whose magnitude but not direction is increasing as a function of time: ##\vec{F}(t+\Delta{t})-\vec{F}(t)## points in the same direction as ##\vec{F}(t)##. You're thinking of the case in which the magnitude of the vector is constant over time, in which case the derivative must indeed be perpendicular (as in acceleration in the case of uniform circular motion).
[Edit: Chet got there first but I used more Latex so I still win
]FAQ: Are a vector and its derivative perpendicular at all times?
What is a vector?
A vector is a mathematical object that has both magnitude (size) and direction. It is represented by an arrow pointing in the direction of its magnitude.
What is a derivative?
A derivative is a mathematical concept that represents the rate of change of a function with respect to its input. It can be thought of as the slope of the function's graph at a given point.
How is a vector's derivative calculated?
A vector's derivative is calculated by taking the derivative of each component of the vector separately. This results in a new vector that represents the rate of change of the original vector at a given point.
When is a vector and its derivative perpendicular?
A vector and its derivative are perpendicular when the dot product of the two vectors is equal to zero. This means that the angle between the two vectors is 90 degrees.
Is a vector and its derivative always perpendicular?
No, a vector and its derivative are not always perpendicular. It depends on the specific function or path that the vector represents. In some cases, the vector and its derivative may be parallel or at any other angle.