Calculating Average Acceleration of a Particle Moving in a Circle

[Slimy0233]

Homework Statement

A particle moves with a constant speed along a circle of radius R. Calculate the average acceleration during the time it takes to cover ${\frac{1}{4}}^{th}$ of the circle.

Calculate the average acceleration using the formula, $<f(x)> = \frac{\int_{x_1}^{x_2}f(x)dx}{x_2 - x_1}$

Relevant Equations

$<f(x)> = \frac{\int_{x_1}^{x_2}f(x)dx}{x_2 - x_1}$

$<\vec{a}> = \frac{\vec{V}_f - \vec{V}_i}{\delta t}$

I was given two HW questions, I was supposed to solve on using

$<\vec{a}> = \frac{\vec{V}_f - \vec{V}_i}{\delta t}$ and another using

$<f(x)> = \frac{\int_{x_1}^{x_2}f(x)dx}{x_2 - x_1}$, I was able to solve using the first formula but I wasn't able to do it with second (at least I got the wrong direction maybe)

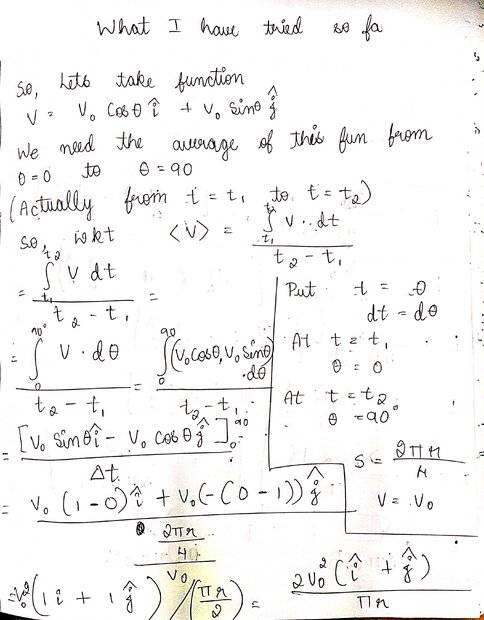
What did I do wrong in "What I have tried so far"? (second image)

Was it wrong to assume $\theta = t$? (Thanks for the help)

 

[kuruman]

It is not clear what you are expected to do with the first formula because acceleration is a vector and the formula with $f(x)$ is not a vector equation. The definition of angular acceleration is the second formula $$\langle \vec a \rangle=\frac{\Delta \vec v}{\Delta t}.$$ This problem should have been clearer about where exactly on the circle one should consider the path of the particle.

 

[Cutter Ketch]

Ok, I see what you are doing. On the third page you use the second equation to get the correct answer as a check, but really you were supposed to get there using the first equation. As @kuruman said the first equation isn’t written as a vector equation, but on your second page you tried to correct that and make it a vector relation.

So what went wrong? I think the problem is that you are averaging velocity over time and not acceleration or force over time. You wrote the velocity vector as a function of theta. You know you can parameterize theta as time. Go ahead and write the velocity as a function of time, take the derivative to get acceleration, and THEN do the integral to get the average of acceleration over that time.

 

[Slimy0233]

It is not clear what you are expected to do with the first formula because acceleration is a vector and the formula with $f(x)$ is not a vector equation.

But, can't you take a vector anyway? also, does that mean, what I did is wrong?

As you might have noticed, I got different answers for (different sign)

edit: as @Cutter Ketch mentioned in #3 2nd para, I was finding out the average velocity instead of acceleration. So, I rectified it and got the right answer. But, one doubt still remains. Was I right in assuming time t was a parameter of $\theta$?

 

[malawi_glenn]

Calculate the average acceleration using the formula,

Was this given in the original problem formulation?

Just calculate $\Delta \vec v$ in some coordinate system and $\Delta t$ and use the formula given in post #2

 

[Slimy0233]

Was this given in the original problem formulation?

It was. It was a lecture actually. He explicitly said so. $\frac{\Delta \vec{v}}{\Delta t}$ is the first method of c

 

[haruspex]

But, can't you take a vector anyway? also, does that mean, what I did is wrong?

As you might have noticed, I got different answers for (different sign)

You do not say whether the motion is clockwise or anticlockwise, nor the initial direction. I assume what is wanted is the magnitude of the average acceleration, so those won't matter.

You started out by writing the expression for average velocity, not average acceleration:
$<\vec v>=\frac{\int \vec v.dt}{\Delta t}$.
This should have produced $\frac{\Delta \vec s}{\Delta t}=\frac {2}{\pi}v(\hat i+\hat j)$.
But then you equated $dt$ to $d\theta$. That is dimensionally wrong; a time is not an angle. The correct translation would be $dt=\frac{dt}{d\theta}d\theta=\frac Rvd\theta$, so instead you got $\frac {2}{\pi}\frac{v^2}R(\hat i+\hat j)$. By sheer luck, that is the average acceleration!

Calculate the average acceleration using the formula, $<f(x)> = \frac{\int_{x_1}^{x_2}f(x)dx}{x_2 - x_1}$

The first challenge is to substitute correctly into that general formula for averages. Since average acceleration means the average wrt time, $x$ must represent time. Since we want an average acceleration, $f$ must represent acceleration. Therefore all occurrences of $f(x)$ become $\vec a(t)$. So to use the formula you need to start by expressing acceleration as a function of time.

 

[Slimy0233]

So what went wrong? I think the problem is that you are averaging velocity over time and not acceleration or force over time. You wrote the velocity vector as a function of theta. You know you can parameterize theta as time. Go ahead and write the velocity as a function of time, take the derivative to get acceleration, and THEN do the integral to get the average of acceleration over that time.

I did do that, thanks to you! and I got the right answer. I still have a doubt tho, was I right* in taking time t as a parameter of $\theta$ as I did in both my solutions? Would you be able to explain this please

 

[Slimy0233]

You do not say whether the motion is clockwise or anticlockwise,

The rotation actually was anticlockwise. I think he wanted us to find the magnitude of the acceleration as well as the direction (so both).

You started out by writing the expression for average velocity, not average acceleration:

Thank you for pointing it out, I actually rectified it (second attempt) and redid it and got the attachment. Unfortunately, I substituted $d\theta$ with $dt$ here too. I got the right answer, but I guess, I would have to redo it.

, so instead you got . By sheer luck, that is the average acceleration!

I think it's not the average acceleration. As you can see in here (second image, not second attempt), the average acceleration would be in the direction $(- \hat{i} + \hat{j})$ and not $(- \hat{i} + \hat{j})$ as you suggested.

But yes, the magnitude is the same.

 

[haruspex]

I think it's not the average acceleration.

Remember that, as noted, I had no info on the rotation direction or starting position, so I could not know the direction of the acceleration, only its magnitude.

But you seem not to have understood the main point of my post #7. It is absolutely not correct to simply take $dt=d\theta$. The only reason it gave you the right magnitude of answer is that the integral you plugged it into was not an expression for the average acceleration in the first place; it was an integral expression for the average velocity.
The blunder of switching $dt$ to $d\theta$ gave an expression with the right magnitude for average acceleration by dumb luck.

However, if you do care about the direction then the mistake will not be so serendipitous. If the rotation is counterclockwise and the initial velocity is along $\hat j$ then the average acceleration will be along $-\hat i-\hat j$, whereas the average velocity will be along $-\hat i+\hat j$.

 

[kuruman]

But, can't you take a vector anyway?

If you want to use the general averaging formula for a vector, you have to time-average the vector components separately because that is how one adds vectors. Write the average acceleration vector as the sum of averages of the components, $$\langle\vec a\rangle=\langle a_x\rangle~\hat i+\langle a_y\rangle~\hat j$$where $$\langle a_x\rangle=\frac{\int_{t_1}^{t_2}a_x ~dt}{\int_{t_1}^{t_2}~ dt}~;~~\langle a_y\rangle=\frac{\int_{t_1}^{t_2}a_y ~dt}{\int_{t_1}^{t_2}~ dt}.$$Note that for uniform circular motion $$\vec a=-\omega^2~\vec r=-\omega^2~\left[R\cos(\omega t+\phi)~\hat i+R\sin(\omega t+\phi)~\hat j\right]$$ where $\omega=v_0/R.$ You should be able to put it together from this point.

Also not that here you have a time interval of a quarter-period. However, the final answer will depend on the choice of the position at time $t=0$ (parametrized by the phase angle $\phi$) that is not given. It would be appropriate to have $\phi$ in your final answer.

 

[Slimy0233]

Remember that, as noted, I had no info on the rotation direction or starting position, so I could not know the direction of the acceleration, only its magnitude.

Oh yes, I am sorry about that.

But you seem not to have understood the main point of my post #7. It is absolutely not correct to simply take .

I am sorry, but I still don't understand how

would be , so

I am sorry, can you tell me how $$\frac{d t}{d\theta}d\theta =\ \frac{R}{v}d\theta$$ ? I understand what you did in post #7 is dimensionally sound and why my method was completely wrong, but I didn't get why $$\frac{d t}{d\theta}d\theta =\ \frac{R}{v}d\theta$$

edit: I am my friend are actually working on it. We will try it! Thank you for your help! it has been very useful!

But you seem not to have understood the main point of my post #7. It is absolutely not correct to simply take $d \theta = dt$.

 

[Slimy0233]

If you want to use the general averaging formula for a vector, you have to time-average the vector components separately because that is how one adds vectors. Write the average acceleration vector as the sum of averages of the components, $$\langle\vec a\rangle=\langle a_x\rangle~\hat i+\langle a_y\rangle~\hat j$$where $$\langle a_x\rangle=\frac{\int_{t_1}^{t_2}a_x ~dt}{\int_{t_1}^{t_2}~ dt}~;~~\langle a_y\rangle=\frac{\int_{t_1}^{t_2}a_y ~dt}{\int_{t_1}^{t_2}~ dt}.$$Note that for uniform circular motion $$\vec a=-\omega^2~\vec r=-\omega^2~\left[R\cos(\omega t+\phi)~\hat i+R\sin(\omega t+\phi)~\hat j\right]$$ where $\omega=v_0/R.$ You should be able to put it together from this point.

Also not that here you have a time interval of a quarter-period. However, the final answer will depend on the choice of the position at time $t=0$ (parametrized by the phase angle $\phi$) that is not given. It would be appropriate to have $\phi$ in your final answer.

I deeply appreciate this post. I am saving it. Thank you!!!

 

[Slimy0233]

To anyone viewing this, I could not put a solved edit on the post, but I believe this no longer requires a great amount of attention. Thank you for everyone who helped me here. I will follow up with what my responsibilities are for having asked this question! :)

 

[haruspex]

can you tell me how $$\frac{d t}{d\theta}d\theta =\ \frac{R}{v}d\theta$$ ?

$\frac{d t}{d\theta}=\frac 1{\frac{d \theta}{dt}}=\frac 1\omega$.
$v_{tangential}=R\omega$.

 

[haruspex]

I did do that, thanks to you! and I got the right answer.

Again, you got the right answer by two errors that happened to cancel.

In post #1, your first error was writing the expression for average velocity instead of average acceleration. In post #8, you effectively did the same but by a different route.
You started with the right integral expression this time, $\frac{\int \vec a.dt}{\Delta t}$, but your expression for $\vec a(t)$ was wrong. From $\vec v=v\cos(\theta)\hat i+v\sin(\theta)\hat j$ you thought you differentiated wrt t, but instead only differentiated wrt $\theta$:
$\frac{dv}{d\theta}=-v\sin(\theta)\hat i+v\cos(\theta)\hat j$.
$\frac{dv}{dt}=\frac{dv}{d\theta}\frac{d\theta}{dt}$.

As in post #1, this error equates to a factor of $\frac vR$, and in each case you cancelled that error by then writing $d\theta=dt$.

The bottom line is that one does not merely integrate or differentiate an expression; these operations are always with respect to a specific variable. The derivative of $\sin(\theta)$ wrt $\theta$ is $\cos(\theta)$, but if you are differentiating wrt another variable then you need a factor representing how changes in those two variables relate.