Capacitors connected in series: Why is the voltage the same?

[iVenky]

I don't think the voltage is the same for all. The charge is same for all, as the internal nets are floating and charge can't come from anywhere else (-Q+Q=0) i.e. the charge conservation holds true.

Q=CV=> implies if C is lower then V is higher

V1+V2+V3=V due to KVL.

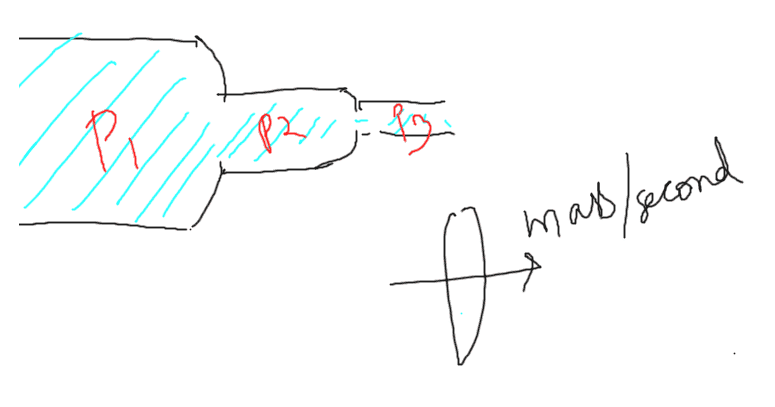
An equivalent analogy is flow of fluid through a pipes P1,P2,P3 of varying diameters as shown.

The mass flow (mass of liquid / second across a cross section area) should be the same for all points (similar to charge)

What changes is the velocity and pressure of pipes P1, P2 and P3 (like voltage described above)

 

[Dale]

Q=CV=> implies if C is lower then V is higher

V1+V2+V3=V due to KVL.

You are talking about the voltages across the capacitors. The OP is asking about the voltage across the wire connecting two capacitors. That voltage is zero so the connected plates are at the same voltage.