- #1
Android Neox
- 23
- 2
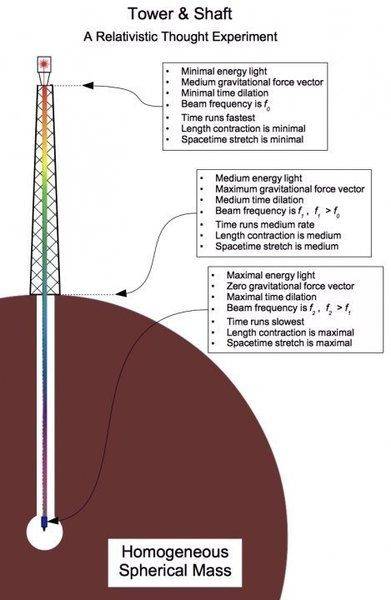
Frames under identical acceleration, regardless of whether due to gravity or accelerated motion, are locally identical but time runs slower in a gravity well. For example, at the center of a large mass (like the one pictured, below) time runs more slowly than for an inertial observer far from any large masses.