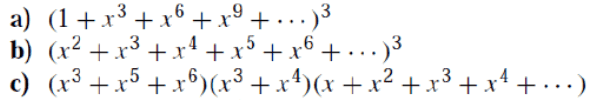MHB Coefficent in a infinite power series

Thread starter pac1337
Start date Jun 3, 2021
Tags

Infinite Power Power series Series

Jun 3, 2021

pac1337

How do I find a coefficent of x^9 in a power series like this:

Physics news on Phys.org

Twisting sound: Scientists discover a new way to control mechanical vibrations in metamaterial
Uncertainty-aware Fourier ptychography enhances imaging stability in real-world conditions
A new scalable approach to realize a quantum communication network based on ytterbium-171 atoms

Jun 3, 2021

HOI

For (a) 9= 0+ 9= 1+8= 2+ 7= 3+ 6= 4+ 5= 5+ 4= 6+ 3= 7+ 2= 8+ 1= 9+ 0.
There are 10 products that give $x^9$ so the coefficient is 10.

Thread 'Formal derivation of statement from Peano Arithmetic system'

I was reading a Bachelor thesis on Peano Arithmetic (PA). PA has the following axioms (not including the induction schema): $$\begin{align} & (A1) ~~~~ \forall x \neg (x + 1 = 0) \nonumber \\ & (A2) ~~~~ \forall xy (x + 1 =y + 1 \to x = y) \nonumber \\ & (A3) ~~~~ \forall x (x + 0 = x) \nonumber \\ & (A4) ~~~~ \forall xy (x + (y +1) = (x + y ) + 1) \nonumber \\ & (A5) ~~~~ \forall x (x \cdot 0 = 0) \nonumber \\ & (A6) ~~~~ \forall xy (x \cdot (y + 1) = (x \cdot y) + x) \nonumber...

View full post »

Thread 'Deductive proof in logic formal systems'

I was reading documentation about the soundness and completeness of logic formal systems. Consider the following $$\vdash_S \phi$$ where ##S## is the proof-system making part the formal system and ##\phi## is a wff (well formed formula) of the formal language. Note the blank on left of the turnstile symbol ##\vdash_S##, as far as I can tell it actually represents the empty set. So what does it mean ? I guess it actually means ##\phi## is a theorem of the formal system, i.e. there is a...

View full post »