- #1
Complex Eigenvalues of system of DE
- Thread starter member 731016
- Start date
-
- Tags
- Complex Differential equations
Click For Summary
Homework Help Overview
The discussion revolves around understanding the equivalence of two expressions in the context of complex eigenvalues related to a system of differential equations.
Discussion Character
- Conceptual clarification, Assumption checking
Approaches and Questions Raised
- Participants are attempting to clarify the transition between two steps in a mathematical derivation, specifically questioning the equivalence of expressions. There is also a focus on identifying potential errors in the expressions presented.
Discussion Status
Some participants have provided guidance on how to approach the problem by suggesting performing multiplications and organizing terms. There is acknowledgment of a typo that contributed to confusion, indicating a productive direction in the discussion.
Contextual Notes
Participants are navigating through expressions that involve complex numbers and matrices, with specific attention to potential sign errors and the implications of these on the overall understanding of the problem.
- #2
- 22,823
- 14,861
Just perform the multiplications and collect real and imaginary terms in their own matrices.
- #3
renormalize
Science Advisor
Gold Member
- 1,184
- 1,365
The yellow expression contains a sign error; the second term should be:$$ChiralSuperfields said:
i\left(\begin{array}{c}
-e^{-2t}\sin3t\\
+e^{-2t}\cos3t
\end{array}\right)
$$
- #4
member 731016
Thank you for your replies @Orodruin and @renormalize ! Yeah that was part of my confusion is that there is a typo
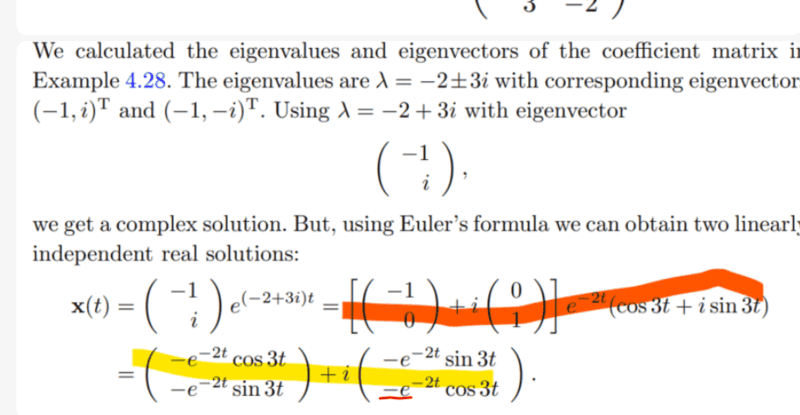
It makes sense now that I know it is a typo!
Thanks!
It makes sense now that I know it is a typo!
Thanks!
Similar threads
- · Replies 4 ·
- · Replies 5 ·
- · Replies 7 ·
- · Replies 6 ·
- · Replies 27 ·
- · Replies 2 ·