Derivation for capacitance of cylindrical capacitor

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

I don't understand how they got from the previous step to the next step of the derivation circled in red:

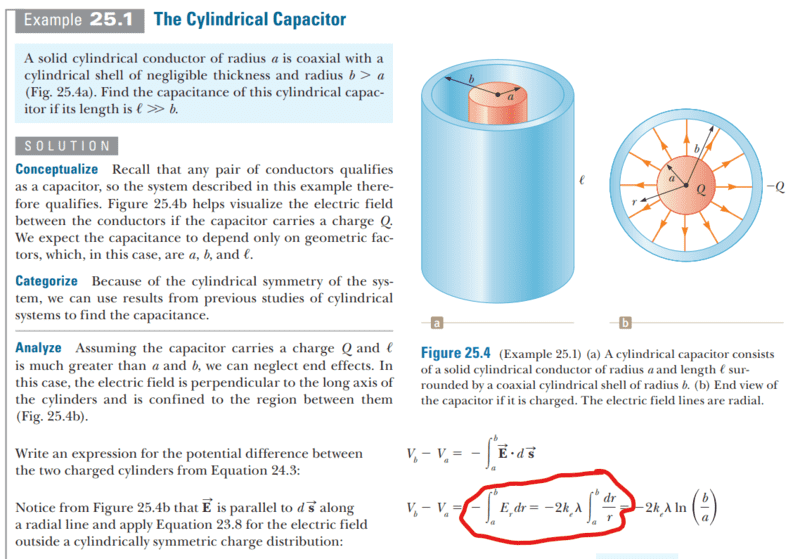
Many thanks!

 

[kuruman]

They replaced $E_r$ with the expression for the radial electric field for a very long rod in the region outside the rod. See equation 23.8 in the textbook that they quote.

 

[member 731016]

They replaced $E_r$ with the expression for the radial electric field for a very long rod in the region outside the rod. See equation 23.8 in the textbook that they quote.

Thank you @kuruman !