Does gas flow from low to high pressure?

[Sailor Al]

TL;DR Summary

Classical Physics states that a gas will only flow from a region of high to one of low pressure and, like water, won't run uphill. This demo of Bernoulli's principle appears to show gas flowing into in the opposite sense, from low to high from the centre constriction to the second wide tube section. What's happening?

Here's a grab at 1:50 from the Bernoulli's Principle Demo: Venturi Tube video

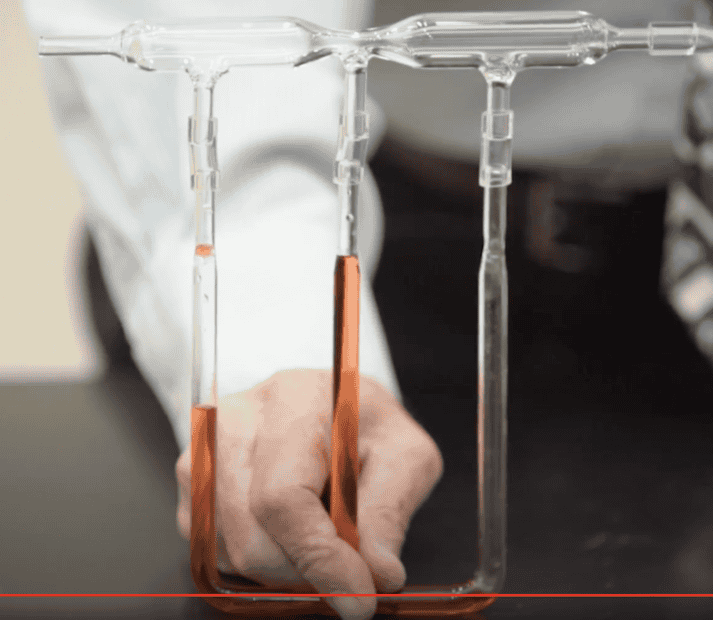
It appears to show the air flowing from the viewer's right to left where the pressure in the third manometer appears to be higher than in the middle one.
Is there some complexity from the manometers being interconnected?
Water won't flow uphill. A gas will only flow from high to low pressure.
How can this be a correct interpretation?

 

[osilmag]

That looks like a drop that is sticking to the side of the tubes. The level on the left is lower than the middle one. It is still a correct demonstration because the downward pressure in the middle tube is lower due to the air having higher velocity laterally in the constricted section.

 

[hmmm27]

Wikipedia : Venturi Effect.

 

[russ_watters]

Wikipedia : Venturi Effect.

More specifically, Bernoulli's principle/equation in the Venturi effect. Try to sort out the issue of there being more than one quantity/measurement with "pressure" in the name.

 

[boneh3ad]

Gas has inertia like any mass. Pressure gradient provides a force, so moving from low to high pressure will slow it down and moving from high to low will speed it up.

 

[Sailor Al]

That looks like a drop that is sticking to the side of the tubes. The level on the left is lower than the middle one. It is still a correct demonstration because the downward pressure in the middle tube is lower due to the air having higher velocity laterally in the constricted section.

I was referring not to the drop of water at the top of the third manometer, but the level of the top of the water column which is indeed lower than in the second column. Isn't the pressure indicated in the second manometer therefore lower than in the third one? Isn't the air moving from a lower pressure in the second one to a higher one in the third one. I don't understand how that can happen.

 

[Sailor Al]

More specifically, Bernoulli's principle/equation in the Venturi effect. Try to sort out the issue of there being more than one quantity/measurement with "pressure" in the name.

Yes, I suspect the manometers are measuring the static pressure and are not detecting the dynamic pressure of the moving air. But I still don't have the complete answer.

 

[Baluncore]

But I still don't have the complete answer.

Where the pressure is lower, the liquid is higher in the vertical manometer tube.

There is a pressure drop across the central constriction.
You can see that from the height of the liquid column on the upstream side (right) and downstream side (left).

The manometer column in the middle shows that the pressure is lower in the central constriction, where the gas flows faster, than it is on either side, in the wide and so, slower flowing sections.

 

[Sailor Al]

Where the pressure is lower, the liquid is higher in the vertical manometer tube.

There is a pressure drop across the central constriction.
You can see that from the height of the liquid column on the upstream side (right) and downstream side (left).

The manometer column in the middle shows that the pressure is lower in the central constriction, where the gas flows faster, than it is on either side, in the wide and so, slower flowing sections.

Yes, and that's what I am having trouble with. The pressure in the third chamber is higher than the pressure in the second, yet the gas is flowing from the second to the third: from low to high pressure. Most references claim that a gas only flows from high to low, but here it seems to be flowing from low to high.

 

[Baluncore]

Yes, and that's what I am having trouble with.

You must be more specific about where your trouble lies.

The pressure in the third chamber is higher than the pressure in the second, yet the gas is flowing from the second to the third: from low to high pressure.

It depends on how you number the chambers. We need clarity.
Flow is from right to left, so maybe you should number them from right to left.

The right-hand side = inlet-chamber has the lowest liquid level in its manometer tube, so the highest pressure is in that chamber.

 

[Baluncore]

The pressure in the third chamber is higher than the pressure in the second, ...

There are only two chambers. The manometer tube in the middle is connected to the restriction between the two chambers.

The fluid velocity is higher in the restriction, so the static pressure there is lower.

Dynamic pressure is irrelevant here because the manometers, that read static pressure, are perpendicular to the flow direction.

 

[Sailor Al]

You must be more specific about where your trouble lies.It depends on how you number the chambers. We need clarity.
Flow is from right to left, so maybe you should number them from right to left.

The right-hand side = inlet-chamber has the lowest liquid level in its manometer tube, so the highest pressure is in that chamber.

OK, let's identify them.
Chambers A, B and C with the gas flowing from A to B to C.
My trouble lies in the flow between chamber B and chamber C.
The pressure in chamber B appears to be lower than the pressure in chamber C.
My problem is that the gas appears to be flowing from a lower pressure (in B) to higher pressure (in C) while most textbooks state that a gas only flows from high pressure to low pressure, so the gas appears to be flowing contrary to the textbooks.

 

[Baluncore]

My problem is that the gas appears to be flowing from a lower pressure (in B) to higher pressure (in C) while most textbooks state that a gas only flows from high pressure to low pressure, so the gas appears to be flowing contrary to the textbooks.

That is because chamber B is a restriction with higher velocity, while A and C have larger diameters, with a lower air velocity.
Air in chamber A flows through the restriction into chamber C, where the pressure is lower. That is exactly what you expect.
Bernoulli's principle says, and conservation of energy requires, a balance of static pressure against velocity in a streamline.

 

[Sailor Al]

That is because chamber B is a restriction with higher velocity, while A and C have larger diameters, with a lower air velocity.
Air in chamber A flows through the restriction into chamber C, where the pressure is lower. That is exactly what you expect.
Bernoulli's principle says, and conservation of energy requires, a balance of static pressure against velocity in a streamline.

Yes, but my issue, as I have stated, is the passage of the air from the narrow chamber B to chamber C: from a lower pressure in B to a higher pressure in C.

 

[Arjan82]

The answer is already given by @boneh3ad here:

Gas has inertia like any mass. Pressure gradient provides a force, so moving from low to high pressure will slow it down and moving from high to low will speed it up.

So air can move just fine towards higher pressure as long as it has enough inertia. The constriction in the middle lowers the pressure but increases the velocity, so here potential energy of the pressure is converted to kinetic energy, and in the downstream part the velocity is lower again, so the kinetic energy is converted to potential energy again.

Bernoulli is an energy conservation statement. It says that the sum between pressure and kinetic energy remains equal (as long as you're not extracting nor providing energy to the flow).

 

[Baluncore]

Yes, but my issue, as I have stated, is the passage of the air from the narrow chamber B to chamber C: ...

I think all you are missing is an understanding of Bernoulli's principle.
https://en.wikipedia.org/wiki/Bernoulli's_principle

 

[jack action]

Water won't flow uphill.

Apparently, you never heard of siphons:

​

As for gas going from low pressure to high pressure, this is literally how an axial compressor works:

​

Note that there are no valves in an axial compressor to prevent the flow from going from the high-pressure zone to the low-pressure zone. Actually, the flow may be reversed in an axial compressor under certain conditions; this is known as compressor surge.

The term you might want to look for is adverse pressure gradient.

 

[boneh3ad]

Apparently, you never heard of siphons:

​

As for gas going from low pressure to high pressure, this is literally how an axial compressor works:

View attachment 326890

​

Note that there are no valves in an axial compressor to prevent the flow from going from the high-pressure zone to the low-pressure zone. Actually, the flow may be reversed in an axial compressor under certain conditions; this is known as compressor surge.

The term you might want to look for is adverse pressure gradient.

Compressors do work on the flow, so it's not a great example. But as I said earlier, all you need is to look at it in terms of $F=ma$. Fluids can flow into higher pressure regions without any external work applied but they will slow down when doing so.

 

[Lnewqban]

Yes, but my issue, as I have stated, is the passage of the air from the narrow chamber B to chamber C: from a lower pressure in B to a higher pressure in C.

In the absence of fluid movement, the air would naturally move from C to B, until both pressures become equal.
But there is movement and therefore velocity and kinetic energy.
That kinetic energy was initially pressure energy.

In order for movement to happen, there must be more mechanical energy in the fluid at the entrance of A (supplied by pressure tank, compressor, blower, etc.) than at the outlet of C (open atmosphere, vacuum, etc).
Disregarding actual loses to friction, the fluid energy between A and C remains constant.

If we measure static pressure and define it as the number (or velocity) of molecules of fluid impacting the walls of a closed container, which free surface of the pink liquid is receiving a higher number of impacts per unit of time and why?
We can't count the number of impacts, we can only see where more or less force of the impacts is being exerted on surfaces.

As the air molecules move horizontally faster through B, there is less time for vertical impacts on the surface of the liquid to happen; therefore, we measure lower static pressure (but higher velocity) there.

As the air molecules move horizontally slower through A and C, there is more time for vertical impacts on the surface of the liquid to happen; therefore, we measure higher static pressure (but lower velocity) there.

The total mechanical energy inside the air is the same in A, B and C, at least in idealized conditions.

Please, see:
https://www.grc.nasa.gov/www/k-12/rocket/pressure.html

https://en.wikipedia.org/wiki/Pressure

https://en.wikipedia.org/wiki/Static_pressure#Static_pressure_in_fluid_dynamics

 

[Baluncore]

Classical Physics states that a gas will only flow from a region of high to one of low pressure and, like water, won't run uphill.

There is a similar effect to Bernoulli, but in an open channel, where water flows uphill in a hydraulic jump. That occurs when the fluid exchanges kinetic energy in a low-sectional-area channel, for potential energy in a higher-sectional-area channel. The water surface rises against gravity, under a fixed atmospheric surface pressure. Energy is conserved.
https://en.wikipedia.org/wiki/Hydraulic_jump

 

[Arjan82]

As the air molecules move horizontally faster through B, there is less time for vertical impacts on the surface of the liquid to happen; therefore, we measure lower static pressure (but higher velocity) there.

As the air molecules move horizontally slower through A and C, there is more time for vertical impacts on the surface of the liquid to happen; therefore, we measure higher static pressure (but lower velocity) there.

I think 'less time for vertical impacts to happen' is a bit vague, since an impact takes a very low (infinitesimally low theoretically?) amount of time.

What I guess you are saying is this:
The velocity of a single molecule increases but if you assume the frequency (or frequency distribution I guess) of its random motion in the vertical direction (or in any direction, i.e. the temperature) to stay equal, then you decrease the number of vertical collisions per unit distance for that single molecule.

This is true for a single molecule, but I've always wondered how this argument would hold when you have large amounts of molecules, like in a gas. Take a pure theoretical example where you have an infinitely long tube of gas and you just by some magic increase the velocity but without changing its density and temperature (e.g. some external forcefield, assume no friction). If what you are saying is true, then the pressure would also need to drop. But if you change your frame of reference to one that moves with the flow through the tube, then the amount of vertical collisions per unit time (i.e. the pressure) remains equal [EDIT] because the temperature and density are equal, this would mean the state of the gas*), or its internal kinetic energy, is equal [/EDIT]. If you then change the frame of reference towards stationary, I don't think the amount of vertical collisions per unit time, i.e. the pressure, changes (I've never done the math here...). This is because if it would, the pressure would change depending on your frame of reference, which is weird.

So I wonder if there is someone at PF that has a better explanation, someone with some good knowledge of the kinetic theory of gasses.

[EDIT]
*) So this is what I'm most unsure about. But I think in this case you can determine the thermodynamic state by just two properties (the state principle?), any two of pressure, temperature, density (or specific volume) or entropy.
[/EDIT]

 

[erobz]

The total mechanical energy inside the air is the same in A, B and C, at least in idealized conditions.

Just to expand on this and maybe explore whether I understand it correctly:

In this experiment there is thermal losses generated from expansion, contraction and viscous shear, which is why the static pressure in the leftmost chamber has not returned to the height of the rightmost chamber. If it were an adiabatic process of an ideal gas, we should expect them to be the same. However, since they are different the adiabatic assumption would not hold here. Heat is escaping to the surroundings.

@Sailor Al
But anyhow, in an attempt to dispel the "useful common misunderstanding" that a flow will never go from lower pressure to a higher pressure...think about this:

Point $A$ is just below the surface of the reservoir, point $C$ is the discharge that is somewhere below point $A$. There is a flow established in the pipe. Imagine manometer taps at $A$ and $B$ something should jump out at you about the flow not being able to go from low to high pressure?

 

[Sailor Al]

Does water flow uphill? No, It won't do so in an open channel, but in a syphon, under capillary action or in a U-bend, water does indeed flow uphill.
In a gas turbine engine, or a desktop fan, air "flows" from Atmospheric pressure to the high pressure downstream of the blades.
Thanks @erobz and @jack action.
And in the video, the air does flow from the low pressure of chamber B to the high pressure of chamber C.
I guess the problem lies in the word "flow".
I don't think the answers will come from quoting Bernoulli or linking to Wikipedia articles, nor will it come from using conversationally non-physics terms such as inertia (the tendency for an object to resist motion!).
Or indeed from considering a gas other than the continuum of an "Ideal gas". Any venture down the path of molecules will end poorly. Air doesn't behave like a stream of ping-pong balls!
I am aware the syphon an U-bend examples involve the conversion of potential to kinetic energy, the gas turbine example has an energy input from the combusting fuel and the desktop fan is using electrical power.
This will take some more thinking about.

 

[russ_watters]

I don't think the answers will come from quoting Bernoulli or linking to Wikipedia articles, nor will it come from using conversationally non-physics terms such as inertia (the tendency for an object to resist motion!).
Or indeed from considering a gas other than the continuum of an "Ideal gas". Any venture down the path of molecules will end poorly. Air doesn't behave like a stream of ping-pong balls!
I am aware the syphon an U-bend examples involve the conversion of potential to kinetic energy, the gas turbine example has an energy input from the combusting fuel and the desktop fan is using electrical power.
This will take some more thinking about.

Do you have a specific question/concern that hasn't been addressed by the answers you've already gotten? I don't feel like this is a difficult issue to understand.

[snip] ...using conversationally non-physics terms such as inertia (the tendency for an object to resist motion!).

Acceleration, not motion....but do you have an issue with inertia too?

 

[TonyStewart]

TL:DR all the threads or video

We know air travels from high to low pressure in a static condition.

There is a vacuum in B is being pumped by the higher velocity air over the orifice coming from the right nozzle with the venturi effects greater over B. So this is not a static condition and the apparent contradiction has a reason.

 

[Sailor Al]

Do you have a specific question/concern that hasn't been addressed by the answers you've already gotten? I don't feel like this is a difficult issue to understand.

The explanation of how water flows upstream in a syphon is answered with simple mechanics: as long as the pressure in the water on the rising side is greater than its vapour pressure, the water will remain a liquid and gets pushed by air pressure over the hump. If the rise is more than 32 feet, then at the top of the hump the water will change form a liquid to a gas the water velocity will reduce to zero.
A similarly simple, basic physics explanation exists for the U-bend and a slightly more complicated one involving surface tension will explain the capillary action.
I still don't have an explanation in similarly basic physics for how the gas can flow from the low pressure of chamber B to the higher pressure chamber B, i.e. one that doesn't depend on the more advanced concepts behind the Bernoulli Principle and Euler's differential equations.

Acceleration, not motion....but do you have an issue with inertia too?

I do have a problem with inertia in that from the physics or mechanics perspective, it doesn't appear to have a clear definition or have any MLT dimensions.

 

[Baluncore]

Does water flow uphill? No, It won't do so in an open channel, but in a syphon, under capillary action or in a U-bend, water does indeed flow uphill.

You missed post #20.

There is a similar effect to Bernoulli, but in an open channel, where water flows uphill in a hydraulic jump.

A bicycle will not roll uphill, unless it rolls downhill gathering speed, across the floor of the valley, then rolls up a hill until it almost stops. There, kinetic energy is being traded for potential energy = m⋅g⋅h.
Gravity * height is the equivalent to the potential energy of pressure in Bernoulli's effect.

 

[russ_watters]

I still don't have an explanation in similarly basic physics for how the gas can flow from the low pressure of chamber B to the higher pressure chamber B, i.e. one that doesn't depend on the more advanced concepts behind the Bernoulli Principle and Euler's differential equations.

I've never considered Bernoulli's Principle to be an advanced concept. I first did some experimenting with it for a project in 9th grade*, before I took my first physics class (though we did have "physical science" that year, which had some physics content). But that doesn't really answer my question, it's just a weird quibble about how advanced the subject is. What's the actual problem?

I do have a problem with inertia in that from the physics or mechanics perspective, it doesn't appear to have a clear definition or have any MLT dimensions.

My understanding is that the term isn't generally used in physics as it is largely redundant with "mass". It's just colloquially a specific property of mass: m=f/a

*For a year-long project I set out with an ambitious goal to design a new fighter-jet (the result looked suspiciously like an F-16 but added a few advanced features I'd read about....). About the only thing I actually accomplished was to build a working wind tunnel powered by an RC plane engine, and measuring the resulting airflow velocity with a home-made pito-static probe and u-tube manometer. The hardest part was figuring out what the heck a "slug" is.

 

[russ_watters]

There is a similar effect to Bernoulli, but in an open channel, where water flows uphill in a hydraulic jump. That occurs when the fluid exchanges kinetic energy in a low-sectional-area channel, for potential energy in a higher-sectional-area channel. The water surface rises against gravity, under a fixed atmospheric surface pressure. Energy is conserved.
https://en.wikipedia.org/wiki/Hydraulic_jump

I was going to point out a more trivial example of water flowing "uphill":



Bernoulli's principle still mostly applies to/explains this.

[actually, I first looked for firefighting videos but didn't quickly find a good one.]

 

[Sailor Al]

You missed post #20.

Sure, that's true, even if it's a bit of a stretch to say that it "flows uphill". But the point has been accepted. Under some circumstances, water does indeed flow uphill.

A bicycle will not roll uphill, unless it rolls downhill gathering speed, across the floor of the valley, then rolls up a hill until it almost stops. There, kinetic energy is being traded for potential energy = m⋅g⋅h.
Gravity * height is the equivalent to the potential energy of pressure in Bernoulli's effect.

Again, no argument, this uphill flowing can be explained in simple physics terms of trading body's KE for PE.
My original claim that water doesn't flow uphill was too much of a generalisation. I'm sorry I mentioned it!
I'm still looking for a similarly simple physics explanation of how a gas flows from low to high pressure. I know it does, I just can't quite work out how.

 

[Baluncore]

Again, no argument, this uphill flowing can be explained in simple physics terms of trading body's KE for PE.

On a bicycle, the lower the road is where you are, the faster you are rolling.
That is the same with the water in the pipe. If it flows faster, it must have a lower pressure.

 

[Sailor Al]

I was going to point out a more trivial example of water flowing "uphill":
[actually, I first looked for firefighting videos but didn't quickly find a good one.]

OK, OK already!

 

[Sailor Al]

On a bicycle, the lower the road is where you are, the faster you are rolling.

WOAH! It's not the altitude ("..the lower the road...") of the road that provides the potential gradient that allows you to trade KE for PE as you roll uphill, it's the gradient of the road.

 

[russ_watters]

WOAH! It's not the altitude ("..the lower the road...") of the road that provides the potential gradient that allows you to trade KE for PE as you roll uphill, it's the gradient of the road.

No. You're misunderstanding here also speaks to part of your apparent issue with Bernoulli I (and several others) mentioned above). How "low" a particular stretch of road is with respect to a baseline speaks to the speed in that stretch. The slope determines the acceleration between "high' and "low" sections.

 

[Arjan82]

I'm still looking for a similarly simple physics explanation of how a gas flows from low to high pressure. I know it does, I just can't quite work out how.

Well, several posts in this thread are devoted to answering this question in the simplest of terms. Apparently that still doesn't explain it for you. I personally wouldn't know how to provide a better answer for you. If you want a better answer, you need to be very specific about what you don't understand about the explanations you already got. Then we can build from there.