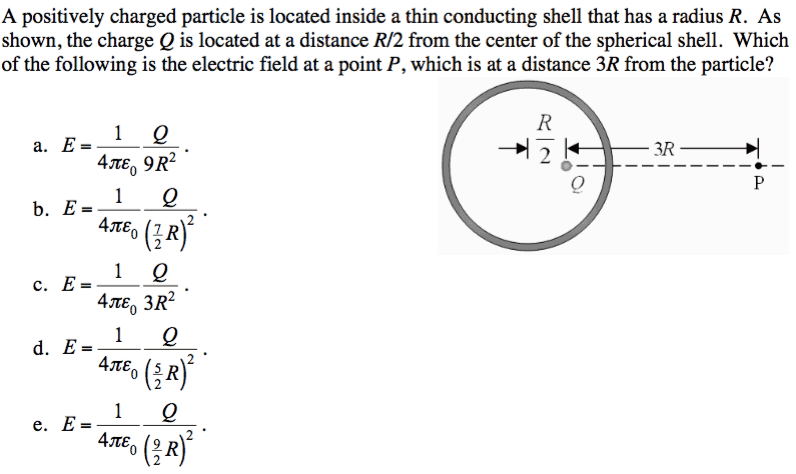The discussion centers on understanding the electric field near a conducting shell with a charge inside. Participants express confusion about the radius and the implications of the charge being within the shell, particularly regarding induced charges on the inner surface. Gauss's law is highlighted as a key tool for analyzing the electric field, emphasizing the importance of symmetry in the problem. The surface of the conducting shell is confirmed to be an equipotential, and the potential outside the shell is determined by boundary conditions. Overall, applying Gauss's law is essential for solving the problem effectively.