Electric Field on the surface of charged conducting spherical shell

[vcsharp2003]

Homework Statement

If a spherical shell conductor having a radius R is uniformly charged with a charge of Q, then what is the electric field at any point on its surface?

Relevant Equations

$E = \dfrac {kQ} {r^2}$ where k is Coulomb's constant, Q is total charge on the spherical shell and r is the distance from center of shell of a point outside the shell at which E needs to be determined. The direction of E is always radial.

For all points within the shell E is zero.

When I look at the relevant equations, then there is no mention of field for a point on the surface of the shell, so it gets confusing. On the other hand, I feel the radial E will get stronger as we approach the surface of shell and magnitude of E will approach infinity.

 

[haruspex]

Homework Statement:: If a spherical shell conductor having a radius R is uniformly charged with a charge of Q, then what is the electric field at any point on its surface?
Relevant Equations:: $E = \dfrac {kQ} {r^2}$ where k is Coulomb's constant, Q is total charge on the spherical shell and r is the distance from center of shell of a point outside the shell at which E needs to be determined. The direction of E is always radial.

For all points within the shell E is zero.

When I look at the relevant equations, then there is no mention of field for a point on the surface of the shell, so it gets confusing. On the other hand, I feel the radial E will get stronger as we approach the surface of shell and magnitude of E will approach infinity.

It's not clear to me whether by "on" the surface the question means a point just outside the surface or a point in the surface.
Either way, it is not infinite. What is the limiting value as the surface is approached from outside?

For the case of a point in the surface, I have seen the question posed in the context of a sphere with a very small circular hole, asking for the field at the centre of the hole. The answer is what you might guess, but it is not trivial to arrive at it analytically.

 

[vcsharp2003]

It's not clear to me whether by "on" the surface the question means a point just outside the surface or a point in the surface.

By on the surface I mean a point on the surface of spherical shell.

 

[vcsharp2003]

What is the limiting value as the surface is approached from outside?

As we come closer to the surface from outside the shell the magnitude of E should increase according to the formula in relevant equations and approach $\dfrac {kQ} {R^2}$. On the other hand approaching the surface from inside we would have E as zero.

 

[haruspex]

By on the surface I mean a point on the surface of spherical shell.

Yes, but I am saying that is still ambiguous. If it means a point outside the shell by an arbitrarily small distance you get one answer. If it means a point in the shell (and I don't mean inside) then you can argue for a different answer by considering it to be at the centre of an arbitrarily small hole in the shell.

 

[vcsharp2003]

Yes, but I am saying that is still ambiguous. If it means a point outside the shell by an arbitrarily small distance you get one answer. If it means a point in the shell (and I don't mean inside) then you can argue for a different answer by considering it to be at the centre of an arbitrarily small hole in the shell.

I am still confused. The field could be zero if approached from inside the shell or $\dfrac {kQ} {R^2}$ if approached from outside as shown in graph below.

I am meaning a point on the shell and not a point that is at an infinitesimal distance outside or inside the shell. The shell is of negligible thickness.

 

[Delta2]

If I remember well from some other threads, doing the integration using coulomb's law that is calculating the integral $$\int_A\frac{\sigma (\vec{r}-\vec{r'})}{|\vec{r}-\vec{r'}|^3}d^2\vec{r'}$$ gives an answer of $\frac{kQ}{2R^2}$ (A the surface area of the sphere and R the radius of the sphere), while using Gauss's law in integral form and taking advantage of the symmetry gives the answer $\frac{kQ}{R^2}$. It's one of the rare cases where coulomb's law and gauss's law are not equivalent.

 

[vcsharp2003]

If I remember well from some other threads, doing the integration using coulomb's law that is calculating the integral $$\int_A\frac{\sigma (\vec{r}-\vec{r'})}{|\vec{r}-\vec{r'}|^3}d^2\vec{r'}$$ gives an answer of $\frac{kQ}{2R^2}$ (A the surface area of the sphere and R the radius of the sphere), while using Gauss's law in integral form and taking advantage of the symmetry gives the answer $\frac{kQ}{R^2}$. It's one of the rare cases where coulomb's law and gauss's law are not equivalent.

By applying Gauss's law and using the enclosed charge for a Gaussian surface we get a field at a point where $r>R$, but not at a point where $r=R$. I was interested for a point $r=R$.

 

[Delta2]

By applying Gauss's law and using the enclosed charge for a Gaussian surface we get a field at a point where $r>R$, but not at a point where $r=R$. I was interested for a point $r=R$.

You can apply Gauss's law for $r=R$ as well. At least that's how I did it. The charge then is at exactly the boundary of the gaussian spherical surface and it still can be considered as enclosed.

 

[vcsharp2003]

You can apply Gauss's law for $r=R$ as well. At least that's how I did it. The charge then is at exactly the boundary of the gaussian spherical surface and it still can be considered as enclosed.

Ok. I was under the impression that enclosed would mean only that charge which is inside the Gaussian surface. The textbooks I have seen always look at $r>R$, so it got confusing for me.

 

[Delta2]

Yes, to be honest, I am not 100% sure about my view, that is if we can consider the charge at exactly the boundary as enclosed but anyway if we consider it as such we get the answer $\frac{kQ}{R^2}$.

 

[vcsharp2003]

Yes, to be honest, I am not 100% sure about my view, that is if we can consider the charge at exactly the boundary as enclosed but anyway if we consider it as such we get the answer $\frac{kQ}{R^2}$.

I saw a similar question where E on the surface is being determined, but with no definite answer at https://physics.stackexchange.com/q...consider-also-the-charge-over-the-gaussian-s#

 

[vcsharp2003]

Yes, to be honest, I am not 100% sure about my view, that is if we can consider the charge at exactly the boundary as enclosed but anyway if we consider it as such we get the answer $\frac{kQ}{R^2}$.

One reason why textbooks don't bother with electric field on the surface may be because we would only want to calculate the electric field at points inside or outside.

 

[Delta2]

One reason why textbooks don't bother with electric field on the surface may be because we would only want to calculate the electric field at points inside or outside.

No, in my opinion they avoid it because they know that the integral of Coulomb's law gives different answer than (Gauss's law in integral form +symmetry).

 

[haruspex]

If I remember well from some other threads, doing the integration using coulomb's law that is calculating the integral $$\int_A\frac{\sigma (\vec{r}-\vec{r'})}{|\vec{r}-\vec{r'}|^3}d^2\vec{r'}$$ gives an answer of $\frac{kQ}{2R^2}$

Which is the result for the point in the middle of a small hole.

There is a requirement for an idealisation to be valid that is too often overlooked: the result must match the limit of all real world versions as they tend to the idealisation.

The thing about the 'hole' model is that in principle it gives a continuous function for the field. If we were to approach the hole from inside the sphere then the field would gradually increase from zero, reaching the above value at radius R, then continuing to be asymptotically the full $\frac{kQ}{R^2}$ further out.

If we insist on there being no hole and the point being exactly at radius R then it becomes indeterminate. We can take three different real scenarios with that as the limiting case and get three different answers: approach from inside, approach from outside, shrink a hole.

Edit: I now withdraw the argument in relation to the limit values as R is approached from above and below. See post #37.

 

[vcsharp2003]

No, in my opinion they avoid it because they know that the integral of Coulomb's law gives different answer than (Gauss's law in integral form +symmetry).

Can we use a Gaussian surface as shown in diagram if we were interested in finding electric field at P, where P is on the spherical shell and shell has negligible thickness? It seems we cannot use this Gaussian surface but not sure. There is something wrong with application of Gauss's law in below manner but cannot pinpoint what is wrong.

The Gaussian surface is denoted by a dashed line in the diagram while the real spherical shell by a solid line.The Gaussian surface is also a perfect sphere. Then we can say that such a Gaussian surface encloses some charge due to the part of actual spherical shell lying with it. We can then say that field inside this Gaussian surface is zero as is the case with a real spherical shell and P being a point inside this surface will also have a zero field.

a

 

[Delta2]

Not sure what you doing there, by choosing a gaussian surface we can talk about the field in points of this surface, not in points on the interior of the surface. Also the surface you are using can't exploit a symmetry.

 

[vcsharp2003]

Not sure what you doing there, by choosing a gaussian surface we can talk about the field in points of this surface, not in points on the interior of the surface. Also the surface you are using can't exploit a symmetry.

But still we know that field inside a spherical shell is zero and I am using that fact. This will be true in any situation. We don't need to use symmetry here.

I was trying to know why my argument is invalid.

 

[vcsharp2003]

Not sure what you doing there, by choosing a gaussian surface we can talk about the field in points of this surface, not in points on the interior of the surface. Also the surface you are using can't exploit a symmetry.

Do you mean that Gaussian surface does not have a symmetrical distribution of charge on it? If yes, then that's the fault with my logic. I can only say that field inside Gaussian surface is zero if this surface has a uniform distribution of charges.

 

[Delta2]

Regardless if the surface exploits a symmetry of the charge distribution or not, you cannot say anything about the field at a point inside the surface, Gauss's law (in integral form) talks about the field in points of the surface, not inside the surface.

 

[kuruman]

As the graph in post #6 shows, the field is discontinuous at the surface. The difference between the field outside and the field inside (i.e. the discontinuity) is $\Delta E=E_{\text{out}}-E_{\text{in}}=\sigma/\epsilon_0$, where $\sigma=\dfrac{Q}{4\pi R^2}$ is the surface charge density. It makes no sense to me to ask what the electric field is right at the surface.

Qualitatively, you can think of it this way. The electric field inside the conductor is zero. Electric field lines, represented by arrows, start on the surface where the charges are. Right at the tail, where one of these arrows starts, what would you say the electric field is and why? Your choices are
(a) zero
(b) $\sigma/\epsilon_0$
(c) Both of the above
(d) None of the above ____________ (explain)

 

[haruspex]

As the graph in post #6 shows, the field is discontinuous at the surface. The difference between the field outside and the field inside (i.e. the discontinuity) is $\Delta E=E_{\text{out}}-E_{\text{in}}=\sigma/\epsilon_0$, where $\sigma=\dfrac{Q}{4\pi R^2}$ is the surface charge density. It makes no sense to me to ask what the electric field is right at the surface.

Qualitatively, you can think of it this way. The electric field inside the conductor is zero. Electric field lines, represented by arrows, start on the surface where the charges are. Right at the tail, where one of these arrows starts, what would you say the electric field is and why? Your choices are
(a) zero
(b) $\sigma/\epsilon_0$
(c) Both of the above
(d) None of the above ____________ (explain)

You left out indeterminate. See post #15.

 

[Delta2]

You left out indeterminate. See post #15.

What do you mean indeterminate? Do you mean infinite? As far as I remember from one thread the coulomb integral converges to $\frac{kQ}{2R^2}$ even without making the small hole assumption.

 

[kuruman]

You left out indeterminate. See post #15.

I didn't really leave it out. You could say that the answer is (d) with the explanation in post #15 filling the blank. @Delta2 could say that it's (d) with the explanation in post #23 filling the blank.

As far as I remember from one thread the coulomb integral converges to $\frac{kQ}{2R^2}$ even without making the small hole assumption.

Do you have a link to this thread? I would like to know more because I am perplexed. If the field is $\frac{kQ}{2R^2}$ at $r=R$ and $\frac{kQ}{r^2}$ at $r= R+\epsilon$ what happens in-between? To me, it looks like the discontinuity at the surface has been shifted from the surface to just outside the surface.

 

[haruspex]

What do you mean indeterminate? Do you mean infinite? As far as I remember from one thread the coulomb integral converges to $\frac{kQ}{2R^2}$ even without making the small hole assumption.

I cannot accept that.
As I wrote, idealisations are only valid if the result matches the limit value of all realistic models that converge to the idealisation. In the present case, we have three realistic models and they produce three different values at the limit. There may be others.
Maybe the Coulomb integral solution you saw implicitly used something equivalent to the shrinking hole model.

I didn't really leave it out. You could say that the answer is (d) with the explanation in post #15 filling the blank.

Except, "none of the above" implies in particular not the values quoted in a, b and c. Indeterminate says you can't rule any of those out.

 

[haruspex]

Do you have a link to this thread?

I found https://www.researchgate.net/publication/345777573_A_proper_integral_for_the_electric_field_at_the_surface_of_a_conducting_sphere
This extract reinforces my suspicion that it is effectively the shrinking hole model:
"We emphasize that the function at the right-hand side of Eq. (1) is undefined only at the point z=R, where it returns the indeterminate form ‘0/0’, remaining bounded and continuous for all other points in the domain −R≤z≤+R.
Then, we have excluded that point from the domain, but we have properly included it there in Eq.
(3), which has led to an elementary, proper integral in Eq.(4).
Mathematically, this can be viewed as a regularization of the improper Riemann integral solved
in Ref. [5], which is a valid procedure since the value of the integral of a bounded function that is continuous in an interval of integration, except at a point belonging to this interval, does not change when we remove this point from the integration domain, as follows from a well-known theorem by Cauchy..."

 

[kuruman]

I found https://www.researchgate.net/publication/345777573_A_proper_integral_for_the_electric_field_at_the_surface_of_a_conducting_sphere
This extract reinforces my suspicion that it is effectively the shrinking hole model:
"We emphasize that the function at the right-hand side of Eq. (1) is undefined only at the point z=R, where it returns the indeterminate form ‘0/0’, remaining bounded and continuous for all other points in the domain −R≤z≤+R.
Then, we have excluded that point from the domain, but we have properly included it there in Eq.
(3), which has led to an elementary, proper integral in Eq.(4).
Mathematically, this can be viewed as a regularization of the improper Riemann integral solved
in Ref. [5], which is a valid procedure since the value of the integral of a bounded function that is continuous in an interval of integration, except at a point belonging to this interval, does not change when we remove this point from the integration domain, as follows from a well-known theorem by Cauchy..."

Thanks for the link. Yes, the passage you quoted seems to be the shrinking hole model. The article also addresses my question "what happens in-between" in the following manner:
The electric field is then discontinuous at the surface of a conducting sphere, leaping from 0 to σ/(2ε₀) when we pass from points inside the sphere to any point at its surface, and then from this to σ/ε₀ for points just outside the sphere.

I find this hard to swallow because I don't know how to reconcile it with the potential that is continuous across the boundary. We know from the Uniqueness Theorem that the potential is constant and given by $V_{\text{I}} = \dfrac{kQ}{R}$ in the region $r<R$ and $V_{\text{II}} = \dfrac{kQ}{r}$ in the region $r>R$. The radial derivative of the potential is well defined in both regions and can be evaluated at $r=R$:$$E_{\text{I}} = -\frac{\partial V_{\text{I}}}{\partial r} =0~;~~E_{\text{II}} = -\frac{\partial V_{\text{II}}}{\partial r}=\frac{kQ}{R^2}=\frac{\sigma 4 \pi R^2}{4\pi \epsilon_0 R^2}=\frac{\sigma}{\epsilon_0}.$$ It doesn't look that there is room for a factor of $\frac{1}{2}$.

 

[haruspex]

I don't know how to reconcile it with the potential that is continuous across the boundary

I don't have a problem with that reconciliation. If we take the proposed behaviour of the field as correct then we can integrate to get the potential, and it produces the standard result. Trouble is, we can change the value of the field at R to anything we like (short of a Dirac delta function) and that still holds true.
In order to go the other way, finding the field from the potential, we have to assume the field is continuous over the interval of interest. That is clearly not the case for an interval spanning the sphere boundary.

 

[Delta2]

Well, the thread I was referring to was here at PF but I 've trouble locating it. I will just redo the coulomb integral myself and report my attempt here. The only thing that changes , when we don't use the infinitesimal hole model, is that the indeterminacy is not of the form 0/0 but of $\sigma/0$ which I don't know if it is enough to make the integral diverge to infinity.

 

[Delta2]

I don't know how to reconcile it with the potential that is continuous across the boundary.

Well, because as you say $E_I$ and $E_{II}$ (which are the left and right derivative of the potential at point $r=R$) are different this means that the derivative at point $r=R$ doesn't exist (at least that's what holds from a purely mathematical point of view when the left and right derivatives are different) and hence the electric field a $r=R$ doesn't exist as well or it is indeterminate as @haruspex says.

PS. "doesn't exist" sounds weird now that I reread my post, maybe I should 've written "it is not defined"

 

[Delta2]

Ok well, If I have setup correctly the integral of coulomb's law and considering only the radial component of electric field, I get that the integral is $$\int_0^{2\pi}\int_0^{\pi}k\sigma\frac{\sin\theta}{2(1-\cos\theta)}\sqrt{1-\frac{\sin^2\theta}{2(1-\cos\theta)}}d\theta d\phi$$. It's a bit complicated on how I end up with this, it involves drawing a good figure , using spherical coordinates for the integration and using law of cosines and law of sines as well to determine the final form of the integrand.

Wolfram does the hard part of the integral for us, that is the integration for theta
https://www.wolframalpha.com/input/?i=integral+sinx/(2(1-cosx))sqrt(1-(sinx)^2/(2(1-cosx)))+from+x=0+to+pi
and it gives an amazing result of 1, so the integral final value after integration with phi is $2\pi k\sigma$ which is equal $\frac{kq}{2R^2}$. I didn't anywhere use an infinitesimal hole, my intuition says that the infinities as we approach from the left and the right are canceled out leaving only the finite radial component $\frac{kq}{2R^2}$.

 

[kuruman]

Ok well, If I have setup correctly the integral of coulomb's law and considering only the radial component of electric field, I get that the integral is $$\int_0^{2\pi}\int_0^{\pi}k\sigma\frac{\sin\theta}{2(1-\cos\theta)}\sqrt{1-\frac{\sin^2\theta}{2(1-\cos\theta)}}d\theta d\phi$$. It's a bit complicated on how I end up with this, it involves drawing a good figure , using spherical coordinates for the integration and using law of cosines and law of sines as well to determine the final form of the integrand.

Wolfram does the hard part of the integral for us, that is the integration for theta
https://www.wolframalpha.com/input/?i=integral+sinx/(2(1-cosx))sqrt(1-(sinx)^2/(2(1-cosx)))+from+x=0+to+pi
and it gives an amazing result of 1, so the integral final value after integration with phi is $2\pi k\sigma$ which is equal $\frac{kq}{2R^2}$. I didn't anywhere use an infinitesimal hole, my intuition says that the infinities as we approach from the left and the right are canceled out leaving only the finite radial component $\frac{kq}{2R^2}$.

Your analysis is correct and is the same as the one shown in @haruspex's link, post #26. Note that by using the half-angle formulas, the integrand in $\theta$ simplifies to $\frac{1}{2}\cos(\frac{\theta}{2})d\theta$ which integrates to $1$.

I don't doubt the result of the Coulomb integral but I still have to explain it to myself in a way that myself can understand it. I was comfortable with "indeterminate" but maybe I shouldn't have been.

 

[vela]

Griffiths considers the force per unit area $\vec f$ on a patch of charge and shows that it's equal to
$$\vec f = \frac 12 (\vec E_{\rm{above}} + \vec E_{\rm{below}}) \sigma,$$ where $\sigma$ is the surface charge density and $\vec E_{\rm{above}}$ and $\vec E_{\rm{below}}$ are respectively the electric field just above and below a small patch of charge. In this case, this result would again say that the electric field at $r=R$ has magnitude $kQ/2R^2$.

 

[hutchphd]

I've lost track of the question. Are you punching a hole in the conducting sphere and assuming the surface charge remains uniform? These seems unusual.

 

[haruspex]

I've lost track of the question. Are you punching a hole in the conducting sphere and assuming the surface charge remains uniform? These seems unusual.

As the hole shrinks to zero the distribution would tend to uniform.

 

[hutchphd]

How is that included in the limit? Clearly they are not independent. Why should this work?

 

[haruspex]

Griffiths considers the force per unit area $\vec f$ on a patch of charge and shows that it's equal to
$$\vec f = \frac 12 (\vec E_{\rm{above}} + \vec E_{\rm{below}}) \sigma,$$ where $\sigma$ is the surface charge density and $\vec E_{\rm{above}}$ and $\vec E_{\rm{below}}$ are respectively the electric field just above and below a small patch of charge. In this case, this result would again say that the electric field at $r=R$ has magnitude $kQ/2R^2$.

That is very interesting since it implies the matter is open to practical demonstration. Even as a thought experiment, there must be a well defined force on the patch, whatever it is, implying a well-defined field.

So I am convinced now, and must find a counter to my argument in post #15.

In reality, the charge is not uniform. There is a finite number of charges with gaps between. Moreover, there is no such thing as a point charge. At the quantum level they are more smeary.
Consider a negative overall charge, and one electron of it that ventures very slightly beyond radius R. Because of the smeared nature of the other charges it does not immediately feel the full force of $kQq/R^2$. Rather, the force will increase smoothly, reaching that over an atomic or subatomic distance.
Likewise, venturing to a shorter radius will not immediately reduce the force to zero.
Thus, the flaw in my reasoning in post #15 is assuming that the usual expressions for the internal and external fields are exact for even the slightest deviation from radius R.

 

[kuruman]

I think I have managed to explain to myself what has been said here so far to my satisfaction. I present it below to summarize my ideas for anyone who might be interested.

Start with $d\vec E=\dfrac{k dq(\vec r-\vec r')}{|\vec r-\vec r')|^3}$. Consider an element on the sphere at $\theta$ and $\phi$. The point of observation is on the $z$-axis. Then
$\vec r = z~\hat z$
$\vec r' = R \sin\theta \cos\phi~\hat x+R \sin\theta \sin\phi~\hat y+ R \cos\theta ~\hat z$
$dq=\sigma R^2\sin\theta~ d\theta d\phi$
$(\vec r-\vec r')=(-R \sin\theta \cos\phi~\hat x-R \sin\theta \sin\phi~\hat y+(z- R \cos\theta) ~\hat z)$
$|\vec r-\vec r'|=(R^2+z^2-2zR\cos\theta)^{1/2}$
$d\vec E=\dfrac{k \sigma R^2\sin\theta (-R \sin\theta \cos\phi~\hat x-R \sin\theta \sin\phi~\hat y+(z- R \cos\theta) ~\hat z)}{(R^2+z^2-2zR\cos\theta)^{3/2}}d\theta~ d\phi$
Integration over $\phi$ results in an axial contribution only,
$dE_z=\dfrac{2 \pi k \sigma R^2\sin\theta (z- R \cos\theta)}{(R^2+z^2-2zR\cos\theta)^{3/2}}d\theta.$
The theta integral is done using the standard substitution $\int_0^{\pi}f(\cos\theta)\sin\theta~d \theta \rightarrow \int_{-1}^{+1}f(u)~du.$ Then
$$E_z=2 \pi k \sigma R^2\int_{-1}^{+1}\frac{(z- R u)}{(R^2+z^2-2zRu)^{3/2}}du.$$The integral can be easily done by a second substitution, $y=R^2+z^2-2zRu$.$$\begin{align}\int_{-1}^{+1}\frac{(z- R u)}{(R^2+z^2-2zRu)^{3/2}}du & =\left. \frac{u z-R}{z^2 \sqrt{R^2-2 R u z+z^2}}\right |_{-1}^{+1} \nonumber \\ &=\frac{z-R}{z^2 \sqrt{R^2-2 R z+z^2}}-\frac{-R-z}{z^2 \sqrt{R^2+2 R z+z^2}}\nonumber \\ & =\frac{z-R}{z^2 \sqrt{(R-z)^2}}+\frac{R+z}{z^2 \sqrt{(R+z)^2}}.\nonumber\end{align}$$ Thus, $$\begin{align}E_z=\frac{2 \pi k \sigma R^2}{z^2}\left[\frac{z-R}{ \sqrt{(R-z)^2}}+\frac{R+z}{ \sqrt{(R+z)^2}}\right]=\frac{2 \pi k \sigma R^2}{z^2}\left[\frac{z-R}{ \sqrt{(R-z)^2}}+1\right]\end{align}$$ We distinguish three cases.
Case I: $R >z$, inside the shell.
Then $\sqrt{(R-z)^2}=R-z$ in which case $$E_z=\frac{2 \pi k \sigma R^2}{z^2}\left[\frac{z-R}{ R-z}+1\right]=\frac{2 \pi k \sigma R^2}{z^2}\left[-\frac{R-z}{ R-z}+1\right]=0$$ as expected.

Case II: $z >R$, outside the shell.
Then $\sqrt{(R-z)^2}=z-R$ in which case $$E_z=\frac{2 \pi k \sigma R^2}{z^2}\left[\frac{z-R}{ z-R}+1\right]=\frac{4 \pi k \sigma R^2}{z^2}=\frac{\sigma R^2}{\epsilon_0 z^2}.$$When we set $z=R$, $E_z=\dfrac{\sigma}{\epsilon_0}.$

Case III: $z =R$, on the shell.
Then $$E_z=\frac{2 \pi k \sigma R^2}{R^2}\left[\frac{R-R}{ \sqrt{(R-R)^2}}+1\right]=2 \pi k \sigma\left[\frac{0}{ \sqrt{(0)^2}}+1\right]=\text{undefined}$$
The article by F.M.S. Lima (reference in post #26) sets $z=R$ before doing the integral. In that case, $$\int_{-1}^{+1}\frac{(z- R u)}{(R^2+z^2-2zRu)^{3/2}}du~\rightarrow~\int_{-1}^{+1}\frac{(R-Ru)}{(R^2+R^2-2R^2u)^{3/2}}du=\frac{1}{2^{3/2}R^2}\int_{-1}^{+1}\frac{(1-u)}{(1-u)^{3/2}}du.$$This last integral is problematic if we allow the upper limit, corresponding to $\cos0$, be equal to 1. This is where the "hole" comes in. We set the upper limit equal to $1-\epsilon~~(\epsilon > 0)$ and proceed. $$\frac{1}{2^{3/2}R^2}\int_{-1}^{1-\epsilon}\frac{(1-u)}{(1-u)^{3/2}}du=\frac{1}{2^{3/2}R^2}\int_{-1}^{1-\epsilon}\frac{du}{(1-u)^{1/2}}=\left .-\frac{2\sqrt{1-u)}}{2^{3/2}R^2}\right |_{-1}^{1-\epsilon}=\frac{1}{R^2}\left(1-\sqrt{\frac{\epsilon}{2}}\right).$$We multiply by the constant and close the "hole" by setting $\epsilon=0$ to get the electric field at $R$, $$E_z=2 \pi k \sigma R^2 \frac{1}{R^2}\left(1-\sqrt{\frac{\epsilon}{2}}\right)= \frac{ \sigma}{2\epsilon_0}.$$Notably, Equation (1) gives the electric field everywhere. If we strip the constants and consider a unit sphere, the functional dependence on $z$ can be rewritten as $$f(z)=\frac{1}{2z^2}(\text{Sgn}[z-1]+1)$$ producing the plot shown below.

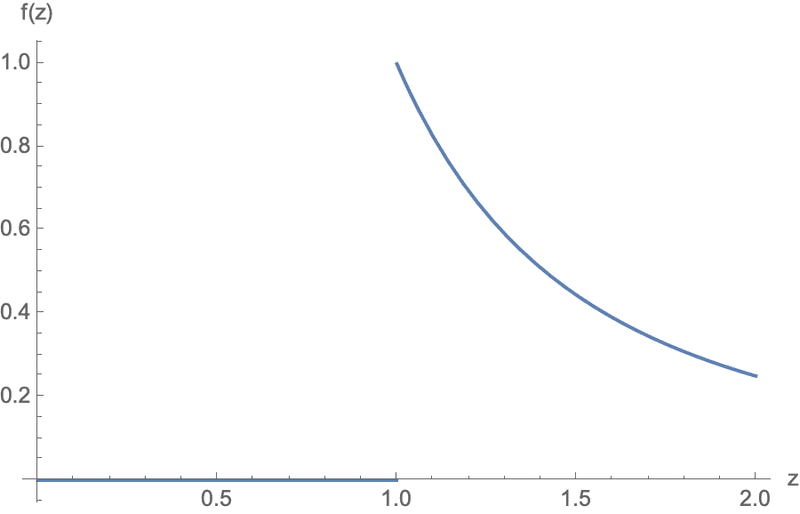
We also note that without the $z^{-2}$ dependence we have a shifted Heaviside step function, $h(z)=\frac{1}{2}(\text{Sgn}[z-1]+1)$ that is equal to zero for $z<1$ and equal to 1 for $z>1.$ Thus, the value of $\frac{1}{2}$ that multiplies the value $\frac{\sigma}{\epsilon_0}$ of the field arbitrarily close to the outer surface of the shell is the Heaviside half-maximum.

I have explained this to myself in a non-mathematical way as follows: Let's say that, for some reason e.g. quantum effects or discreteness of charges, the transition of the electric field from zero (just inside) to $\frac{\sigma}{\epsilon_0}$ (just outside) is not infinitely sharp. Then it can be approximated by some function, that goes smoothly from zero to $\frac{\sigma}{\epsilon_0}$. It is reasonable to say that, by symmetry, the point that is neither inside nor outside the "surface" is the inflection point of the smoothing function, i.e. the Heaviside half-maximum (see Wikipedia article).

 

[hutchphd]

This is the Cauchy principal value argument as I recall...works for me!

 

[Delta2]

It is worth noting that the excellent analysis of post #38 by @kuruman , as well as mine of post #31, is based on Coulomb's law which is not one of Maxwell's equations. The relevant Maxwell equation is Gauss's law in integral form (plus symmetry argument) which agrees with Coulomb's law in the cases r<R and r>R.

But what Gauss's law gives for r=R? If we take a gaussian surface that is the same as the charged sphere then we have a problem: The charges lies exactly on this surface so is it considered enclosed or not?

If we consider it enclosed then Gauss's law gives $\frac{kq}{R^2}$, if we consider it not enclosed then Gauss's law gives 0. In any case the result is different than what we get by Coulomb's law.

 

[alan123hk]

This discussion is very interesting, there are many excellent mathematical arguments
But if my understanding is correct, this is to find the electric field strength in a zero-thickness and zero-volume space, then another feeling of mine is that, apart from theoretical research value, there does not seem to be much practical application demand and value.

 

[haruspex]

apart from theoretical research value, there does not seem to be much practical application demand and value.

As noted in post #33 (which you Liked) it does tell you the pressure exerted on the sphere. That could have practical relevance.

 

[kuruman]

It is worth noting that the excellent analysis of post #38 by @kuruman , as well as mine of post #31, is based on Coulomb's law which is not one of Maxwell's equations. The relevant Maxwell equation is Gauss's law in integral form (plus symmetry argument) which agrees with Coulomb's law in the cases r<R and r>R.

But what Gauss's law gives for r=R? If we take a gaussian surface that is the same as the charged sphere then we have a problem: The charges lies exactly on this surface so is it considered enclosed or not?

If we consider it enclosed then Gauss's law gives $\frac{kq}{R^2}$, if we consider it not enclosed then Gauss's law gives 0. In any case the result is different than what we get by Coulomb's law.

It is not clear to me that Gauss's law gives a different result from Gauss's law. After all, Coulomb's law, an experimental result, is summarized by Gauss's law. The integral form of Maxwell's equation known as Gauss's law is $$\int_S\vec E\cdot \hat n~dS =\frac{1}{\epsilon_0}\int_V \rho(r)~dV$$ where
$S$= a closed surface and $\hat n$ an outward normal to it;
$V$ = the volume enclosed by the surface.
Specifically, if $S$ is a sphere of radius $R$, the radial part of the volume integral on the right has an upper limit $r=R$. Now consider a Gaussian spherical surface of radius $R$ with a concentric, conducting thin shell of radius $a$ inside it ($a<R$) having total charge $Q$ evenly distributed on its surface. The volume charge distribution is $\rho(r) = \frac{Q}{4\pi a^2}\delta (r-a)$. When we apply Gauss's law to this, we use the standard symmetry arguments to write the left-hand side of the equation as $E 4\pi R^2$ regardless of what's on the right-hand side. Here $E$ is the radial component of the field at $r=R$. Thus, $$E 4\pi R^2 =\frac{1}{\epsilon_0}\int_0^R \frac{Q}{4\pi a^2}\delta (r-a)4\pi r^2~dr=Q$$Note that if we shrink $a$ to zero, we have a point charge at the origin and an equation that gives the electric field at distance $R$ from it according to Coulomb's law. Thus, doing the Coulomb integrals to find the electric field at the surface is a superposition of a result obtained from Gauss's law and cannot give a different result from Gauss's law.

Let's apply Gauss's law to the situation of this thread. We expand the radius of the concentric charged shell from $a$ to $R$. Then $$E 4\pi R^2 =\frac{1}{\epsilon_0}\int_0^R \frac{Q}{4\pi R^2}\delta (r-R)4\pi r^2~dr$$How to handle the upper limit in view of the delta function in the integrand? It cannot be changed because it has to match the left-hand side. One can expand $a$ in the charge distribution to $(R+\epsilon)$ in which case the integral is zero or to $(R-\epsilon)$ in which chase the result is $Q$. This is consistent with the results obtained from doing the Coulomb integrals in cases I and II, post #38.

What bothers me now is that, if we accept the results of case III or the Griffiths argument presented by @vela in post #33, the implication is that one can do a delta function integral when the upper limit is the value where the argument of the delta function vanishes: $$E 4\pi R^2 =\frac{1}{\epsilon_0}\int_0^R \frac{Q}{4\pi R^2}\delta (r-R)4\pi r^2~dr=\frac{Q}{2}.$$Can one assert that this is true for the specific case of a charged conductor but not in general? Although Griffiths's argument is based on physical grounds, the Coulomb integral in case III is a mathematical argument.

 

[alan123hk]

As noted in post #33 (which you Liked) it does tell you the pressure exerted on the sphere. That could have practical relevance.

This mathematical formula $\vec f = \frac 12 (\vec E_{\rm{above}} + \vec E_{\rm{below}}) \sigma,~$ is that we can calculate the pressure exerted on the boundary based on the average value of the electric field strength immediately above/below the boundary, but we do not need to know the electric field strength on the boundary.

According to my understanding, here we assume that all charges are distributed on this zero-thickness boundary, and try to find the electric field strength at this zero thickness boundary accurately.

Of course, there may be also a theoretical electric field strength value on this boundary, but I think it seems unnecessary in most practical engineering applications to find out what this value is.

 

[TSny]

Using energy concepts you can argue for $E =\large \frac{kQ}{2R^2}$ at the surface.

The electrostatic energy $U$ in the system can be calculated as $$U =\frac {\varepsilon_0}{2} \int E^2 d\tau$$ where the integration is over all of space. Evaluating this for the uniformly charged spherical surface gives $$U = \frac{kQ^2}{2R}$$ If we allow the sphere to expand an infinitesimal amount $dR$, the energy change $dU$ must equal the negative of the work $dW$ done by the electric force acting on the surface charge. Let $f$ be the force per unit area acting on the surface charge. Then, $-dU = dW$ gives us $$\frac{kQ^2}{2R^2}dR = 4 \pi R^2 f dR$$ $$f = \frac{kQ^2}{(2R^2)(4\pi R^2)} = \frac{kQ}{2R^2}\sigma$$ Since $f$ should equal $\sigma E_{\rm at \, surf}$, we identify $E_{\rm at \, surf} = \large \frac{kQ}{2R^2}$.

 

[haruspex]

This mathematical formula $\vec f = \frac 12 (\vec E_{\rm{above}} + \vec E_{\rm{below}}) \sigma,~$ is that we can calculate the pressure exerted on the boundary based on the average value of the electric field strength immediately above/below the boundary, but we do not need to know the electric field strength on the boundary.

According to my understanding, here we assume that all charges are distributed on this zero-thickness boundary, and try to find the electric field strength at this zero thickness boundary accurately.

Of course, there may be also a theoretical electric field strength value on this boundary, but I think it seems unnecessary in most practical engineering applications to find out what this value is.

You seem to have missed the point.
Consider e.g. a charged bubble. The repulsion results in outward pressure on the bubble, increasing its radius beyond the uncharged version. This is in principle measurable.

 

[vela]

Let's apply Gauss's law to the situation of this thread. We expand the radius of the concentric charged shell from $a$ to $R$. Then $$E 4\pi R^2 =\frac{1}{\epsilon_0}\int_0^R \frac{Q}{4\pi R^2}\delta (r-R)4\pi r^2~dr$$How to handle the upper limit in view of the delta function in the integrand? It cannot be changed because it has to match the left-hand side. One can expand $a$ in the charge distribution to $(R+\epsilon)$ in which case the integral is zero or to $(R-\epsilon)$ in which chase the result is $Q$. This is consistent with the results obtained from doing the Coulomb integrals in cases I and II, post #38.

I found a reference (http://cloud.crm2.univ-lorraine.fr/pdf/uberlandia/Estevez_Delta_Dirac.pdf) which addresses this question. It defines the Dirac Delta function as
$$\int_a^b f(x)\delta(x-x_0)\,dx = \begin{cases}
\frac 12[f(x_0^+)+f(x_0^-)] & x_0 \in (a,b) \\
\frac 12 f(x_0^+) & x_0 = a \\
\frac 12 f(x_0^-) & x_0 = b \\
0 & x_0 \not\in [a,b]
\end{cases}$$ where $f$ is piecewise continuous on $[a,b]$. The other references I checked didn't really discuss the case where $x_0$ is one of the endpoints of the integration.

 

[Delta2]

I found a reference (http://cloud.crm2.univ-lorraine.fr/pdf/uberlandia/Estevez_Delta_Dirac.pdf) which addresses this question. It defines the Dirac Delta function as
$$\int_a^b f(x)\delta(x-x_0)\,dx = \begin{cases}
\frac 12[f(x_0^+)+f(x_0^-)] & x_0 \in (a,b) \\
\frac 12 f(x_0^+) & x_0 = a \\
\frac 12 f(x_0^-) & x_0 = b \\
0 & x_0 \not\in [a,b]
\end{cases}$$ where $f$ is piecewise continuous on $[a,b]$. The other references I checked didn't really discuss the case where $x_0$ is one of the endpoints of the integration.

That's nice but it seems like "semi-nonsense" when we apply Gauss's law like this. We decide that half of the charge is enclosed, and the other half is not enclosed when the gaussian spherical surface is right at $r=R$. While in my opinion the logical thing to do is to either say the whole charge is enclosed, or the whole charge is not enclosed.

 

[vela]

That's nice but it seems like "semi-nonsense" when we apply Gauss's law like this. We decide that half of the charge is enclosed, and the other half is not enclosed when the gaussian spherical surface is right at $r=R$. While in my opinion the logical thing to do is to either say the whole charge is enclosed, or the whole charge is not enclosed.

Consider this case. A charge $Q$ is located at the origin, and you use a hemispherical Gaussian surface centered on the origin. There's no flux across the flat face, and only half the flux from the charge passes through the rest.

 

[Delta2]

Consider this case. A charge $Q$ is located at the origin, and you use a hemispherical Gaussian surface centered on the origin. There's no flux across the flat face, and only half the flux from the charge passes through the rest.

You present another example where we should consider half the charge. Both of the cases involve charge densities that are expressed as Dirac delta functions. Fine. I just can't digest the fact that we seem to use some sort of "Solomonian" solutions in physics, that is we can't decide if the charge is enclosed or not enclosed so we consider half the charge enclosed and half not enclosed.

What if the gaussian surface is 3/4 of a sphere, again centered at the point charge, now we should consider 3/4 of the charge in order for Gauss's law to hold?