unscientific
- 1,728
- 13
In this section, they derive the Sommerfeld formula.
In the first step it seems like they have expanded
Also how did they get from the 2nd to the 3rd step?
Simply by comparing terms we see they are different:
For 2nd step we get terms of
For 3rd step we get terms of
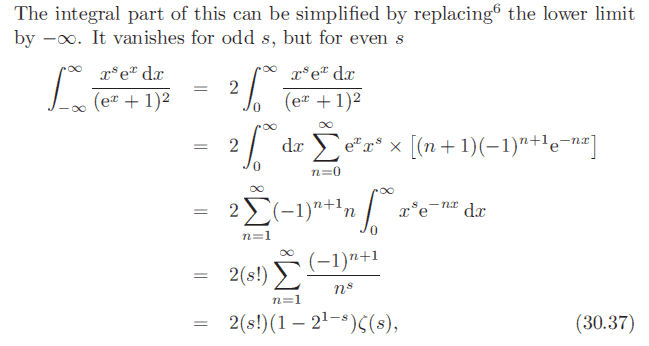
In the first step it seems like they have expanded
Also how did they get from the 2nd to the 3rd step?
Simply by comparing terms we see they are different:
For 2nd step we get terms of
For 3rd step we get terms of