DrChinese said:
2. There are many "alternative" ways to prevent contributions from a section of the mirror. If you blocked it, for example. Or in your version of the experiment, which in my opinion is impossible to implement, there wasn't a wide enough time window for the path to be traversed, that would be an alternative method.
I am fine with the alternative of blocking the grating if that helps anything in this discussion.
I am not sure what you mean by impossible. If you mean my initial example that used times of 10 seconds, that was clearly an in principle experiment not meant to be practical. If you mean your back of an envelope calculation where you used a distance to the mirror of 1000 wavelengths and came up with 0.003 femtoseconds then that was your choice of experimental setup. I came up with some more reasonable numbers in my discussion with gentzen with a difference of 90 nanoseconds. That said, I don't see why in principle you can't make the difference in paths as long as you want.
DrChinese said:
3. I think everyone is saying the same things: there is no FTL effects. So another issue here is that reflection of light is a more complicated process than we are describing. You can't really draw a straight line from a source to a point on the mirror and then to the detector and say "it went this way". As a result, there may appear to be paths that loosely appear to be FTL. But no such path can be demonstrated as such experimentally, because the true picture is quite different.
If that is the truth that there are no FTL effects, I certainly can accept that.
I don't know what you mean by loosely appear FTL.
DrChinese said:
4. I don't follow you here. There are no FTL contributions if you get strict enough. You would be violating the uncertainty principle if you tried to assert you know a particle's position at 2 precise points in time, and momentum in between.
I have not asserted I know anything about a particle position or momentum with any certainty in anything I have presented in this thread. I have asserted that the grating has an effect on the probability at the detector and I have theorized that there is something that goes from the source to the grating to the detector that can't go faster than light. By making this "something" go on a longer path, I was thinking my experiment would demonstrate that this "something" only has an effect on the probability at the detector when allowing for enough time in this experiment for this "something" to transverse its path and make it to the detector.
DrChinese said:
5. There isn't a scenario where you can distinguish A and B. There is always quantum uncertainty in addition to technological constraints. At some point as the time window shrinks, there just won't be any photons emerging from the small time window.
I am confused what you mean that there is no scenario where you can distinguish A and B. First of all that is my goal, to show that I get the same result for scenario A and B. However if you let A and B run for long enough time, I expect it to go to the scenario that Feynman presented which he clearly says there is a difference! So clearly we are not on the same page unless you are agreeing with my expectation which it does not sound like it.
To be clear we are on the same page, let's use the more practical version of my experiment that I presented to gentzen and use a 200 m mirror and assume red light (700 nm, 430e15 cycles / second) and:
put the source in the middle of the mirror, 100 m from the mirror
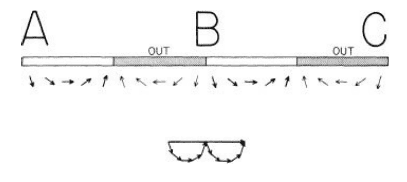
put the grating at the far left end of the mirror (Scenario B only)
put the detector at the far right end of the mirror, 100 m from the mirror
Then as a rough calculation:
The time for light to reach the grating is about 470 ns.
The time for light to reflect off the grating and go past the source is about 840 ns.
The time for light to reflect off the grating and reach the detector is about 1200 ns.
The time for light to take the shortest path, reflect off the mirror and reach the detector is 750 ns.
In this experiment, I would turn on the light source and look at the data from detector for up to 840 ns.
In scenario A there is no grating. We simply turn on the light source and record the accumulated intensity at the detector for 840 ns. We run this scenario many times to get an average accumulated intensity.
In scenario B there is a grating at the far left end of the mirror. We simply turn on the light source and record the accumulated intensity at the detector for 840 ns. We run this scenario many times to get an average accumulated intensity.
If I compare scenarios A and B, my expectation is that I will NOT see any difference in the average accumulated intensity (i.e. the intensity I averaged over many trials).
If I run scenarios A and B for longer than 840 ns they will become closer and closer, the longer I run them, to the scenarios Feynman described and I will see a difference in accumulated intensity.
So now that you clearly understand what I am comparing. Can you say whether you agree or disagree with my expectations and explain why?
DrChinese said:
------------------------------------
As you continue to battle about conducting your experiment, you are actually attempting - whether you know it or not - to say that you can perform an experiment that would yield complete certainty in measurements of non-commuting observables. You either already accept that as impossible per QM, or you are tilting at a windmill using the mirror as a disguise in the process.
I don't understand what you mean when you say "whether you know it or not - to say that you can perform an experiment that would yield complete certainty in measurements of non-commuting observables",
That said if you are implying I am trying to say I can perform an experiment that would yield complete certainty in my measurements. I would not say that at all. I am collecting averages over many trials. I am not expecting individual trials to measure the same intensity, but I do expect given enough trials that I will converge on some consistent average value. Even if I am measuring random noise, I will converge on some consistent average value.
So can you explain what the non-commuting observables are in my experiment?
DrChinese said:
I don't think any of us can help you much further, as it has already been demonstrated no FTL events are occurring. Once you accept that, your question is fully answered.
My expectation has always been that there would be no FTL events and no FTL effects in this scenario. So I have no problem accepting it and a little confused that you think I would.
In my mind an FTL effect would be like the EPR scenario, but in my scenario there is no local preparation (as vanhees71 has described in other threads) between the source and the grating and so that has been my rationale for expecting no FTL effect.
So if there are clearly no FTL effects then I am mostly satisfied. I would still appreciate a definitive answer on my experimental discrepancies that I list above. If you clearly understand what I am doing in the experiment, you should be able to definitively say what the result of the experiment will be. You can tell me that I can't conclude anything useful because my result but I would still like you to tell me what the result will be.
I look forward to completing this thread as soon as I can, but I would like to end on some clarity.
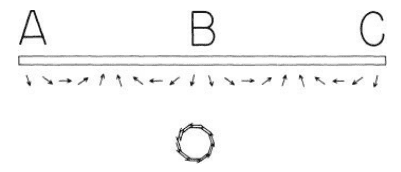
 Feynman then explains how the probability amplitudes of reflections around A and B tend to cancel out and thus don't contribute very much to the final probability. Here is the illustration of this:
Feynman then explains how the probability amplitudes of reflections around A and B tend to cancel out and thus don't contribute very much to the final probability. Here is the illustration of this: