- #1
kurt101
- 284
- 35
- TL;DR Summary
- Do events that can't actually happen because of the speed of light limitations contribute to probabilities that are calculated using the Feynman's path integral method?
In Richard Feynman's book "The Strange Theory of Light and Matter", in chapter 2, he explains how to calculate the probability that light from some source will be reflected by a mirror and be detected at some location. He explains how you sum up all of the probability amplitudes (represented as vectors) for each way light can reflect off of a mirror and end up at the detector and then square this result to get the final probability. Here is the illustration of this:
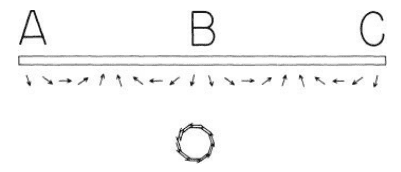
 Feynman then explains how the probability amplitudes of reflections around A and B tend to cancel out and thus don't contribute very much to the final probability. Here is the illustration of this:
Feynman then explains how the probability amplitudes of reflections around A and B tend to cancel out and thus don't contribute very much to the final probability. Here is the illustration of this:
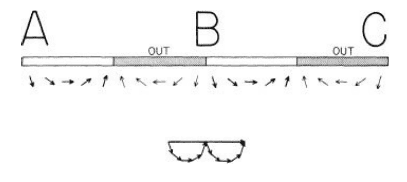
Feynman then explains that if you remove sections of the mirror at A and B that you can change the contributions of the probability amplitudes around A and B so that they do contribute significantly to the final probability. Here is the illustration of this:
My Question:
What happens if you turn on the light source for a small amount of time and only detect the light that could have reflected from the middle of the mirror (around say E through I) in the amount of time that it takes light from the source traveling at the speed of light to reflect and arrive at the detector. In this case would you eliminate the contributions to the final probability from the reflections around A and B because they could not have possibly contributed because of the speed of light?
Feynman then explains that if you remove sections of the mirror at A and B that you can change the contributions of the probability amplitudes around A and B so that they do contribute significantly to the final probability. Here is the illustration of this:
My Question:
What happens if you turn on the light source for a small amount of time and only detect the light that could have reflected from the middle of the mirror (around say E through I) in the amount of time that it takes light from the source traveling at the speed of light to reflect and arrive at the detector. In this case would you eliminate the contributions to the final probability from the reflections around A and B because they could not have possibly contributed because of the speed of light?