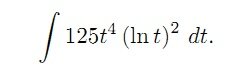Find Velocity of Particles: Indefinite Integrals

[Madu]

View attachment 8167

To help find the velocity of particles requires the evaluation of the indefinite integral of the acceleration
function, a(t), i.e.
v = Z a(t) dt.

Your help greatly appreciated.

 

[MarkFL]

I would think IBP could be used to obtain:

$$\int 125t^4\ln^2(t)\,dt=t^5\left(25\ln^2(t)-10\ln(t)+2\right)+C$$

If you want help actually deriving this formula, please let me know. :)

 

[Greg]

For some useful information in understanding IBP, see here.

$$\begin{align*}\int 125t^4\ln^2(t)\,dt&=25t^5\ln^2(t)-50\int t^4\ln(t)\,dt \\
&=25t^5\ln^2(t)-50\left(\frac15t^5\ln(t)-\frac15\int t^4\,dt\right) \\
&=25t^5\ln^2(t)-10t^5\ln(t)+2t^5+C\end{align*}$$