Finding the force on an inclined plane

[cbarker1]

Dear Everybody,

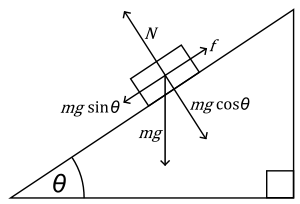
What force (in N) must be applied to a 150.0 kg crate on a frictionless plane inclined at 30° to cause an acceleration of 7.1 m/s² up the plane?

Work:
I know the sum of the force in the x direction must be equal to mass multiply by acceralation.Thanks
Carter

 

[MarkFL]

Let's assume the applied force is parallel to the incline plane...and so we require:

$$F_{\text{net}}-F_g=m\cdot7.1\,\frac{\text{m}}{\text{s}^2}$$

What is the magnitude of the force due to gravity ($F_f$) along the plane?

 

[cbarker1]

Let's assume the applied force is parallel to the incline plane...and so we require:

$$F_{\text{net}}-F_g=m\cdot7.1\,\frac{\text{m}}{\text{s}^2}$$

What is the magnitude of the force due to gravity ($F_f$) along the plane?

I believe it is $$m\cdot g\cdot \cos\left({30}\right)$$.

 

[MarkFL]

I believe it is $$m\cdot g\cdot \cos\left({30}\right)$$.

Think about the cases where the plane is either vertical or horizontal...does the cosine function makes sense?

 

[cbarker1]

Think about the cases where the plane is either vertical or horizontal...does the cosine function makes sense?

NO, it does not make any sense. so it must be sine function.

 

[MarkFL]

NO, it does not make any sense. so it must be sine function.

Yes, it is the sine function...here's a free-body diagram:

What do you find for $F_{\text{net}}$?

 

[cbarker1]

Yes, it is the sine function...here's a free-body diagram:
What do you find for $F_{\text{net}}$?

Is that $$F_N=mass\cdot 7.1+m\cdot g\cdot sin 30$$ correct?

Sorry, I have misread the question that there is a force pushing it up the inclined plane.

 

[MarkFL]

Is that $$F_N=mass\cdot 7.1+m\cdot g\cdot sin 30$$ correct?

Sorry, I have misread the question that there is a force pushing it up the inclined plane.

That's correct, although we know:

$$\sin\left(30^{\circ}\right)=\frac{1}{2}$$

And so we may write:

$$F_{\text{net}}=m\left(7.1+\frac{g}{2}\right)\text{ N}$$

Next, use:

$$m=150.0\text{ kg},\,g=9.8\,\frac{\text{m}}{\text{s}^2}$$

So, what do you get?

 

[cbarker1]

the answer is 1800 N

 

[MarkFL]

the answer is 1800 N

Yes, I concur. (Yes)

 

[cbarker1]

I did the problem correctly.