- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Paolo Aluffi's book: Algebra: Chapter 0 ... ...
I am currently focussed on Section 5.4 Free Abelian Groups ... ...
I need help with an aspect of Aluffi's preamble to introduce Proposition 5.6 ...
Proposition 5.6 and its preamble reads as follows:
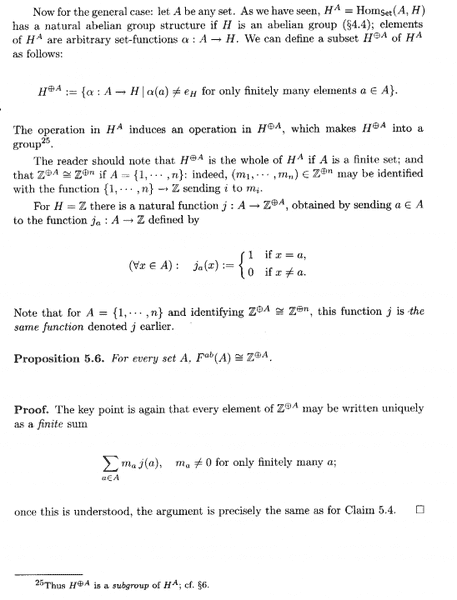
In the above text from Aluffi's book we find the following:
" ... ... For ##H = \mathbb{Z}## there is a natural function ##j \ : \ A \longrightarrow \mathbb{Z}^{ \oplus A }## , obtained by sending ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## ... ... "
My problem is in (precisely and rigorously) understanding the claim that ##j## sends ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## ... ...
The function ##j_a## is actually a set of ordered pairs no two of which have the same member ... BUT ... ##j## does not (exactly anyway) seem to send ##a \in A## to this function ...If we pretend for a moment that A is a countable ordered set ... then we can say that what ##j## seems to do is send ##a \in A## to the image-set of ##j_a##, namely
##( \ ... \ ... \ ,0,0, 0, \ ... \ ... \ 0,1,0 \ ... \ ... \ ... \ \ ,0,0, 0, \ ... \ ... )##
where the ##1## is in the ##a##'th position ...
So then ##j## seems to send ##a \in A## to the image-set of ##j_a## and not to ##j_a## itself ... ...(... ... not sure how to put this argument for the case where the set ##A## is uncountable and not ordered ... ... )
Given my analysis ... how do we justify or make sense of Aluffi's claim that " there is a natural function ##j \ : \ A \longrightarrow \mathbb{Z}^{ \oplus A }## , obtained by sending ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## "
Hope someone can critique my analysis and clarify the issue to which I refer ...
Help will be much appreciated ...
Peter*** EDIT ***
Just another concern over possibly missing something in fully understanding Aluffi's text above ... he introduces the general case with a general abelian group ##H## ... ... and then defines ##H^{ \oplus A}## ... ... but never uses ##H## ... he just puts it equal to ##\mathbb{Z}## ... if you are just going to put ##H = \mathbb{Z}## ... ... why bother with ##H## ... does anyone have an idea what Aluffi is doing ... ... ? ... ... he does a similar thing when explaining free modules ... ... am I missing something ... ... ? ... ...
======================================================
To give Physics Forum members reading this post a sense of the approach and notation of Aluffi to free Abelian groups I am here providing Aluffi's introduction to free Abelian groups up to and including Proposition 5.6 ... as follows:
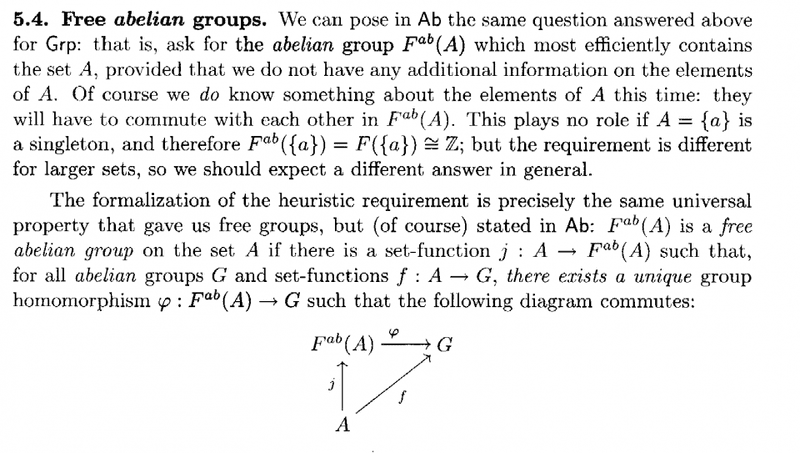


I am currently focussed on Section 5.4 Free Abelian Groups ... ...
I need help with an aspect of Aluffi's preamble to introduce Proposition 5.6 ...
Proposition 5.6 and its preamble reads as follows:
In the above text from Aluffi's book we find the following:
" ... ... For ##H = \mathbb{Z}## there is a natural function ##j \ : \ A \longrightarrow \mathbb{Z}^{ \oplus A }## , obtained by sending ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## ... ... "
My problem is in (precisely and rigorously) understanding the claim that ##j## sends ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## ... ...
The function ##j_a## is actually a set of ordered pairs no two of which have the same member ... BUT ... ##j## does not (exactly anyway) seem to send ##a \in A## to this function ...If we pretend for a moment that A is a countable ordered set ... then we can say that what ##j## seems to do is send ##a \in A## to the image-set of ##j_a##, namely
##( \ ... \ ... \ ,0,0, 0, \ ... \ ... \ 0,1,0 \ ... \ ... \ ... \ \ ,0,0, 0, \ ... \ ... )##
where the ##1## is in the ##a##'th position ...
So then ##j## seems to send ##a \in A## to the image-set of ##j_a## and not to ##j_a## itself ... ...(... ... not sure how to put this argument for the case where the set ##A## is uncountable and not ordered ... ... )
Given my analysis ... how do we justify or make sense of Aluffi's claim that " there is a natural function ##j \ : \ A \longrightarrow \mathbb{Z}^{ \oplus A }## , obtained by sending ##a \in A## to the function ##j_a \ : \ A \longrightarrow \mathbb{Z}## "
Hope someone can critique my analysis and clarify the issue to which I refer ...
Help will be much appreciated ...
Peter*** EDIT ***
Just another concern over possibly missing something in fully understanding Aluffi's text above ... he introduces the general case with a general abelian group ##H## ... ... and then defines ##H^{ \oplus A}## ... ... but never uses ##H## ... he just puts it equal to ##\mathbb{Z}## ... if you are just going to put ##H = \mathbb{Z}## ... ... why bother with ##H## ... does anyone have an idea what Aluffi is doing ... ... ? ... ... he does a similar thing when explaining free modules ... ... am I missing something ... ... ? ... ...
======================================================
To give Physics Forum members reading this post a sense of the approach and notation of Aluffi to free Abelian groups I am here providing Aluffi's introduction to free Abelian groups up to and including Proposition 5.6 ... as follows:
Attachments
Last edited:



