PainterGuy
- 938
- 73
Hi,
Could you please help me with the queries below?
Question 1:
Do you think that the free-body diagram for M1 correct? It looks fine except that, I think, the arrow for M_{1}\frac{d^{2}}{dt^{2}}x_{1} should point downward.
Question 2:
Similarly, I think that the free-body diagram for M2 is okay but the arrow for M_{2}\frac{d^{2}}{dt^{2}}x_{2} should point downward instead. Could you please confirm it?
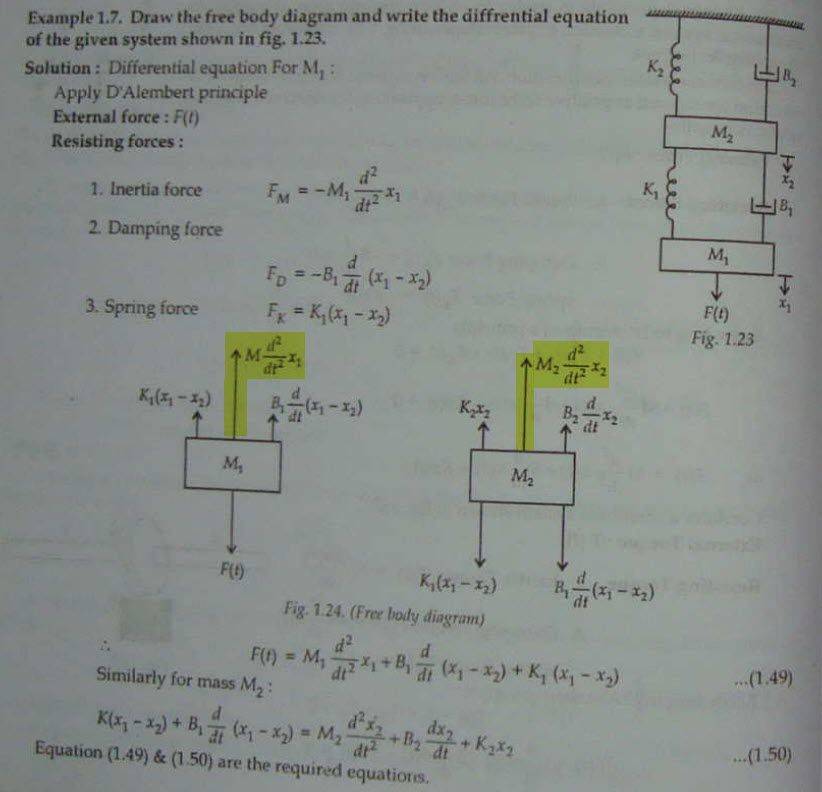
Could you please help me with the queries below?
Question 1:
Do you think that the free-body diagram for M1 correct? It looks fine except that, I think, the arrow for M_{1}\frac{d^{2}}{dt^{2}}x_{1} should point downward.
Question 2:
Similarly, I think that the free-body diagram for M2 is okay but the arrow for M_{2}\frac{d^{2}}{dt^{2}}x_{2} should point downward instead. Could you please confirm it?