Gravitons Replacing General Relativity: Can it Work?

[SamRoss]

TL;DR Summary

If gravitons act only on particles with mass, how can they replace general relativity?

Einstein's theory describes gravity as a curvature of spacetime. As such, everything is affected by it. This includes light, which has no mass, as was made clear for the first time during the famous 1919 solar eclipse. In the standard model, the cause of gravity is supposed to be gravitons, which, at least according to the chart below, only act on objects with mass.

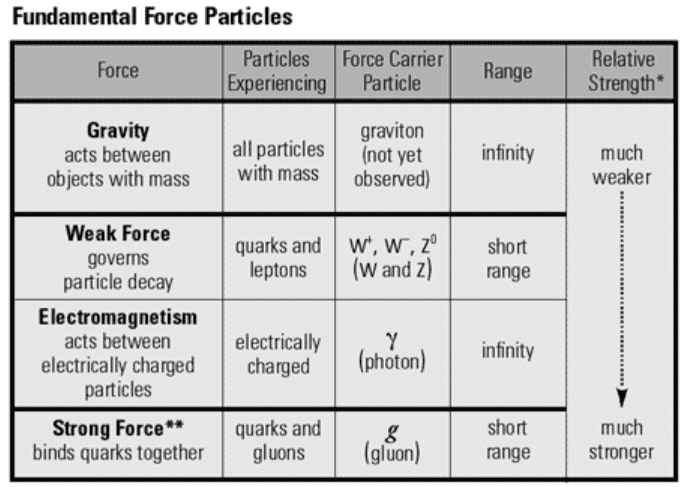
How could this possible be a replacement for general relativity if we know that massless light is affected by gravity?

 

[anuttarasammyak]

You are right so the description in the chart does not seem trustworthy.

Say an electron and a positron annihilate and two photon appear. Moments before and after the reaction gravity field around the point does not change.

 

[Vanadium 50]

Expecting a panel with 70 words on ito to accurately capture every aspect of physics is a bit unrealistic.

 

[Ibix]

Where did you get the chart from? You should always cite your sources. Sometimes when you do so you'll realize that the source is or is not trustworthy. And we may be able to assess that too, possiblt better than you.

You are correct that the chart is oversimplified. If mass was the only source of gravity, frame dragging (detected by Gravity Probe B) and gravitational waves (LIGO) would not be a thing.

 

[vanhees71]

It's plain wrong. It's unfortunately a quite common misconception about the gravitational interaction mixing different theories of their description, i.e., the Newtonian theory of gravity with GR as the standard relativistic theory of it.

In Newtonian gravitation theory it's correct that mass is the source of the gravitational field, obeying the field equation
$$\Delta \Phi=-\gamma \rho,$$
where $\rho$ is the mass density and $\Phi$ the gravitational potential. Also the coupling constant of a test particle to the gravitational field is its mass $m$, i.e., the force on a test particle in the gravitational field is given by
$$\vec{F}=-m \vec{\nabla} \Phi.$$
The fact that we set both the "active mass", i.e., mass distributions as source of the gravitational field and the "passive mass", i.e., the mass as a coupling strength to the gravitational field equal makes use of the strong equivalence principle, because it also sets these two notions of mass equal to the third one which in Newtonian theory is most fundamental, i.e., the mass as measure of inertia in Newton's 2nd Law, $m \vec{a}=\vec{F}$.

For Einstein, famously the weak equivalence principle, i.e., the equality of the "inertial mass" and the "passive gravitational mass", was the starting point to develop a relativistic theory of gravitation, but it became quite quickly clear to him that then it cannot be mass which is determining the coupling strength to the gravitational field, because in special relativity it was clear from the very beginning that energy is the measure of inertia (where I use mass exclusively in the modern sense of "invariant mass"). Then using the strong equivalence principle this also implies that the source of gravity cannot be mass but must have to do with the energy distribution of matter (and then also electromagnetic fields, because it's plausible to consider gravity as a universally acting interaction with all energy distributions).

Now it's immediately clear that energy is not a scalar but part of the four-momentum vector and in the continuum description of matter thus the only covariant object is the energy-momentum stress tensor, and indeed the final solution of Einstein's problem to find a relativistic theory of the gravitational interaction was general relativity with the surprising twist that gravity and inertia is simply the same phenomenon and that the gravitational interaction can be reinterpreted as the effect of a spacetime manifold taking part in the dynamics of matter and radiation. The equivalence principle then is reformulated as the existence of a local inertial reference frame at any point in spacetime, and thus that spacetime can be described as a pseudo-Riemannian manifold with a fundamental form of signature (1,3) (I'm following the west-coast convention). In standard GR it's also assumed that the connection is torsion free, and since it should be "metric compatible" it's uniquely determined by the fundamental form, defining the Christoffel symbols wrt. arbitrary local coordinates in the usual way, and the Einstein field equations
$$G_{\mu \nu}=\kappa T_{\mu \nu},$$
where $G_{\mu \nu}$ is the Einstein tensor, involving the curvature of spacetime and $T_{\mu \nu}$ the energy-momentum tensor of matter and the em. field.

An important input in Einstein's finding GR was that indeed it has a non-relativistic limit, where $T_{\mu \nu}$ can be approximated by "free-streaming dust", $T_{\mu \nu}=\rho u_{\mu} u_{\nu}$ with $\rho$ the mass density and $u_{\mu}$ the four-velocity field of the dust. And then having $\vec{v}=\vec{u}/u^0$. Then you can derive Newton's theory of gravitation as the non-relativistic limit of GR, and it becomes clear that in Newtonian gravity one can consider only mass as being the source of the gravitational field, because in the non-relativistic limit the rest-mass density of matter is much larger than any other contributions to the energy density.

 

[PeterDonis]

In the standard model, the cause of gravity is supposed to be gravitons

No. Gravitons do not appear in the Standard Model of particle physics at all.

at least according to the chart below, only act on objects with mass

No. In the quantum field theory of a spin-2 field on flat spacetime, which was investigated by many physicists in the 1960s and 1970s, the graviton (the spin-2 field) couples to anything with energy. That includes light.

I don't know where you got the chart you posted from, but it is not accurate.

 

[SamRoss]

I don't know where you got the chart you posted from, but it is not accurate.

Are there any other problems with it?

 

[PeterDonis]

Are there any other problems with it?

The main problem right now is that you haven't told us where it comes from. You need to reference your source.

 

[SamRoss]

The main problem right now is that you haven't told us where it comes from. You need to reference your source.

I got it from Google images after typing something like "four fundamental forces" or "force carriers". I don't remember exactly. It seemed to outline some useful information in a concise way. Other than the words "acts between objects with mass" and "all particles with mass", is the information accurate?

 

[member 656954]

I got it from Google images after typing something like "four fundamental forces" or "force carriers". I don't remember exactly.

@SamRoss, you can use Google reverse image search and / or tineye to backtrack image sources. Tineye suggests it was first online early in 2016 and this article may be the source. I'm not sure that NOVA is the original artist, but they would likely attribute third-party images and they haven't so they're likely the source:

https://www.pbs.org/wgbh/nova/education/activities/3012_elegant_02.html

Futurism then used it in this article:

https://futurism.com/physicists-think-they-might-have-just-detected-a-fifth-force-of-nature

 

[Vanadium 50]

Other than the words "acts between objects with mass" and "all particles with mass", is the information accurate?

Expecting a panel with 70 words on ito to accurately capture every aspect of physics is a bit unrealistic.

 

[phinds]

Expecting a panel with 70 words on it to to accurately capture every aspect of physics is a bit unrealistic.

What is "a bit" doing in that sentence at all? Ah, wait. I get it. Sarcasm (and well deserved).

 

[PeterDonis]

I got it from Google images

Sorry, this isn't a valid reference. Either give us a specific URL or this thread will be closed.

Other than the words "acts between objects with mass" and "all particles with mass", is the information accurate?

It's too vague to be called "accurate".

 

[pervect]

A simple check of Wiki confirms that gravity is not part of the standard model. That's one of the first things that the current wiki article on the standard model, https://en.wikipedia.org/w/index.php?title=Standard_Model&oldid=987548423, mentions.

The Standard Model of particle physics is the theory describing three of the four known fundamental forces (the electromagnetic, weak, and strong interactions, and not including the gravitational force) in the universe, as well as classifying all known elementary particles.

So the OP's claim

In the standard model, the cause of gravity is supposed to be gravitons, which, at least according to the chart below, only act on objects with mass.

is based on a misunderstanding of the standard model, possibly fueled by references that have no clear origins.

It is true that light does not have mass, though it does have energy and momentum, and it also true that gravity deflects light. The classical solution to this dilemma is well known. In the end, it is not "mass" that causes gravity in General Relativity, it is the combination of energy, momentum, and pressure, in the form of the geometrical object (or tensor) known as the stress-energy tensor, that causes gravity.

While it is not classical general relativity, one author, Strauman has an interesting take in his paper, "Reflections on Gravity", as to how gravity can be motivated from an approach that starts with "gravitions". The gravitons of this theory are spin-2 particles which exist in a hypotehtical flat space-time with no space-time curvature. Pursuing the theory further yields that this hypothetical flat space-time is not observable. What is actually observable turns out to be the curved space-time of classical General relativity.

Strauman's approach to gravity may have some limits of applicability if the topology of GR is not constrained to be simply connected, though the author unfortunately does not discuss these issues. But it can serve a useful motivational tool for describing how GR can arise from a non-geometrical approach - which is basically the author's point.

A pedagogical description of a simple ungeometrical approach to General Relativity is given, which follows the pattern of well understood field theories, such as electrodynamics. This leads quickly to most of the important weak field predictions, as well as to the radiation damping of binary pulsars. Moreover, certain consistency arguments imply that the theory has to be generally invariant, and therefore one is bound to end up with Einstein's field equations. Although this field theoretic approach, which has been advocated repeatedly by a number of authors, starts with a spin-2 theory on Minkowski spacetime, it turns out in the end that the flat metric is actually unobservable, and that the physical metric is curved and dynamical.

 

[PeterDonis]

The gravitons of this theory are spin-2 particles which exist in a hypotehtical flat space-time with no space-time curvature. Pursuing the theory further yields that this hypothetical flat space-time is not observable. What is actually observable turns out to be the curved space-time of classical General relativity.

Yes, this is the spin-2 field theory I referred to in post #6.

 

[vanhees71]

It doesn't matter too much, where this comes from. It seems a bit superficial anyway, as the discussion so far already shows.

In my opinion it's generally misleading to tell that interactions are "mediated by particles" when discussing quantum field theories for the public. The usual narrative starts with electromagnetism, which is a good choice since we have at least some everyday experience with electromagnetic interactions. Most directly the electrostatic force comes to mind or the force which holds a magnet on the fridge. A not so direct but still intuitive fact is that light, radio/cell-phone waves, X-rays, etc. all are electromagnetic wave fields of different frequency/wave lengths.

Then it's also not too difficult to provide a qualitative picture about the "field paradigm", i.e., the idea that forces like the electrostatic force between two charges are discribed as "local phenomena", which is the great achievement of Faraday's idea of the electromagnetic field: It's not a "spooky action at a distance" as the Newtonian picture of forces suggest but that any electric charge implies the existence of an electromagnetic field around it (an electric field if the charge is at rest in an inertial frame of reference and in addition a magnetic field if it is somehow moving) and that the force on another charge is due to the presence of the electromagnetic field at the place of this charge, i.e., it introduces a local concept.

Then you can come back to the observation that temporal changes in the electromagnetic field due to the (accelerated) motion of charges leads to electromagnetic waves, which carry energy, momentum and angular momentum with them, which implies that they taking part in the dynamics as do the ("material") particles.

All this is challenging for the popular-science writer to explain and the reader to understand, but usually it seems to be managed not too badly by the popular-science writers (I'd have to check out good or bad examples in the popular-science literature).

Usually the trouble starts when the popular-science writers try to provide the idea of quantized fields. The first problem is to motivate the necessity to "quantize" fields to begin with. Usually the narrative is along the historical development, which is not bad in general, beause it can make a quite exciting story to tell about Planck, Einstein, de Broglie, Bohr (old quantum theory) and finally Born, Jordan, Heisenberg, Schrödinger, and Dirac (new quantum theory). The real trouble usually starts, in my opinion, when the popularization ends with the "wave-particle dualism" of the old quantum theory rather than trying to avoid this self-contradictory picture, which was the main motivation of all the above mentioned celebreties to invent (or discover?) the modern version of quantum mechanics and finally relativistic quantum field theory.

Concerning the electromagnetic field, I'd emphasize Plancks standpoint, i.e., the discovery that in order to explain some aspects of the interaction between the em. field with matter, one needs the quantization of the exchange of energy and momentum with electromagnetic waves of a given frequency/wavelength in terms of the corresponding quanta $E=h \nu=\hbar \omega$ and $p=h/\lambda=\hbar k$ (Planck 1900, Einstein 1905, de Broglie 1923) and tell about the usual experimental facts (Planck radiation law, which started the quantum revolution, photoelectric effect, Compton effect,...). I would avoid to talk about photons as "particles" completely but stick to the field picture with the said qualification about the discreteness of exchange of energy, momentum (and angular momentum) between the em. field and (charged) particles. Of course one also has to argue that the "particles" themselves have also to be described by quantum theory and (quantized) wave fields. Then it's clear from the very beginning, that the most modern point of view is rather a field than a particle view and that particle-like phenomena are understandable from this field view through field quantization. Then there's no trouble with the interactions ("forces") due to the exchange of particles, but it's still mediated by fields, which gives a much more comprehensible and consistent picture even on the popular-science level. It's of course no question that this is the case for any science study from the high school to university level.

 

[pervect]

It doesn't matter too much, where this comes from. It seems a bit superficial anyway, as the discussion so far already shows.

In my opinion it's generally misleading to tell that interactions are "mediated by particles" when discussing quantum field theories for the public. The usual narrative starts with electromagnetism, which is a good choice since we have at least some everyday experience with electromagnetic interactions. Most directly the electrostatic force comes to mind or the force which holds a magnet on the fridge. A not so direct but still intuitive fact is that light, radio/cell-phone waves, X-rays, etc. all are electromagnetic wave fields of different frequency/wave lengths.

It takes a bit more effort to make the "particle" narrative work for quantum mechanics than the field narrative, I agree. The narrative doesn't handle diffraction (single slit or double slit) very gracefully. Single slit diffraction is already a bit of a stretch for the narrative, when one adds in the interference fringes and the nulls that result from the double slit experiment, the narrative really struggles.

One can always add onto to the popular narrative, as Feynman did in QED, that the particles take "all possible paths" and "interfere with themselves". Unfortunately, Feynman doesn't really demonstrate how to use the narrative to calculate much of anything in QED.

The narrative does suggest that the Euler-Lagrange equations are the classical limit of QED, which is a good thing to know. But it may be lost on the reader who isn't familiar with the Euler - Lagrange equations, arising from the principle of least action.

I do think that starting with the principle of least action, the Lagrangian of discrete systems, and then moving onto the field concept as a Lagrangian density, i.e. Lagrangian field theory, for continuous systems, is more productive. And certainly, classical gravity can be understood as a Lagrangian field theory. With the right background, the resulting mathematics is very simple to write down

$$\mathcal{L}_g = \sqrt{-g} R$$

which basically relates the contribution of gravity to the Lagrangian as being proportional to a single number R, which is an invariant associated with the curvature of space-time.

While this doesn't result in an understanding of quantum gravity, at the moment there is no agreed-on theory of quantum gravity, so it's unrealistic to expect a popularization of quantum gravity.

Unfortunately, popular readers get very attached to their narrative, it's easier to give them a sequel to their existing narrative than to have them start reading a new narrative, a new story, even if it turns out to be a very good and gripping story.

Still, it may be good to at least point out the existence of such narratives, even if experience leads me to believe it will be difficult to get a reader to pay attention to them, that they'd rather stick with the familiar stories than read new ones.

 

[bobob]

How could this possible be a replacement for general relativity if we know that massless light is affected by gravity?

One way is to think of it by analogy to the "curvature" in qed. In qed, the curvature is the electromagnetic tensor, $F^{\mu\,\nu}$. It may be obtained by taking the commutator of the covariant derivatives:
$$[D_\mu, D_\nu] = ieF_{\mu\, \nu}$$

Where $D_\mu = \partial_\mu - ieA_\mu,$ $\,A_u$ is the electromagnetic field, and $e$ is the electromagnetic coupling constant. The commutator implicitly operates on scalar field. You then quantize $A_\mu$ . The analogous "Bianchi" identity for the electromagnetic field is:
$$D_\alpha F_{\beta\,\gamma} + D_\gamma F_{\alpha\,\beta} + D_\beta F_{\gamma\,\alpha} = 0$$
You can look at the graviton similarly (although naively). The covariant derivative for the gravitational field operates on a vector field and is given by:
$$\nabla_\mu V^\alpha = (\partial_\mu + \Gamma^\alpha_{\mu\,\nu})V^{\nu}$$
and,
$$[\nabla_\mu, \nabla_\nu] V^\alpha = R^\alpha_{\,\beta\,\mu\,\nu} V^\beta$$

The Riemann tensor plays the same role for gravity that the electromagnetic tensor does for qed. The connection coefficients, $\Gamma^\alpha_{\mu\,\nu},$ play the role of the gravitational field analagous to the electromagnetic field $A^\mu$. So, the graviton would in someway, be associated with the connection coefficients. Take $A^\mu$ to be the vector field. (By the way, this apparently does not work for quantizing gravity. It's intended to address your question about how gravitons could affect light in a heuristic way. )

 

[vanhees71]

That analogy is not "naive" but mathematically natural since both E&M and GR are gauge theories.