- #1
MichaelTam
- 93
- 6
- Homework Statement
- MIT pretest.
- Relevant Equations
- 𝐅⃗=−𝑏𝑒^(𝑐𝑣)𝐢̂ , find v(t), by using differential equation of F=ma
There ‘s an equation, I think it should like a “drag factor” because the -(b) now has a negative symbol, which means it should be affecting the particle going parallel of the x-axis to the right, I suppose F=ma need to be apply into this equation, but before this, I need to get it into a differential equation first, but I don’t know how to isolate the exponential element, what I know is to give natural log to them , but the next step I don’t know how to do.
A particle of mass 𝑚 moving parallel to the x-axis is acted on by a velocity dependent force directed against its motion. The force is given by:
where 𝑏 is a positive constant (units N), 𝑐 ia also a positive constant (units s·m−1), and 𝑣 is the speed, the magnitude of the particle's velocity (units m·s−1).
If at 𝑡=0, the particle is moving with speed 𝑣0. Find the speed 𝑣(𝑡) as a function of time 𝑡. Express your answer in terms of some or all of the following: 𝑏, 𝑚, 𝑡, 𝑐, and v_0 for 𝑣0. Use ln() for the natural logarithm function and e^() for the exponential function if needed.
This problem I can’t reach the solution have two main reason, the first is , I get too few knowledge of exponential equation (mathematical problem) and I still feel confuse about the equation of how to derive it with an exponential element in the equation in order to get the differential equation and substitute the v(t).
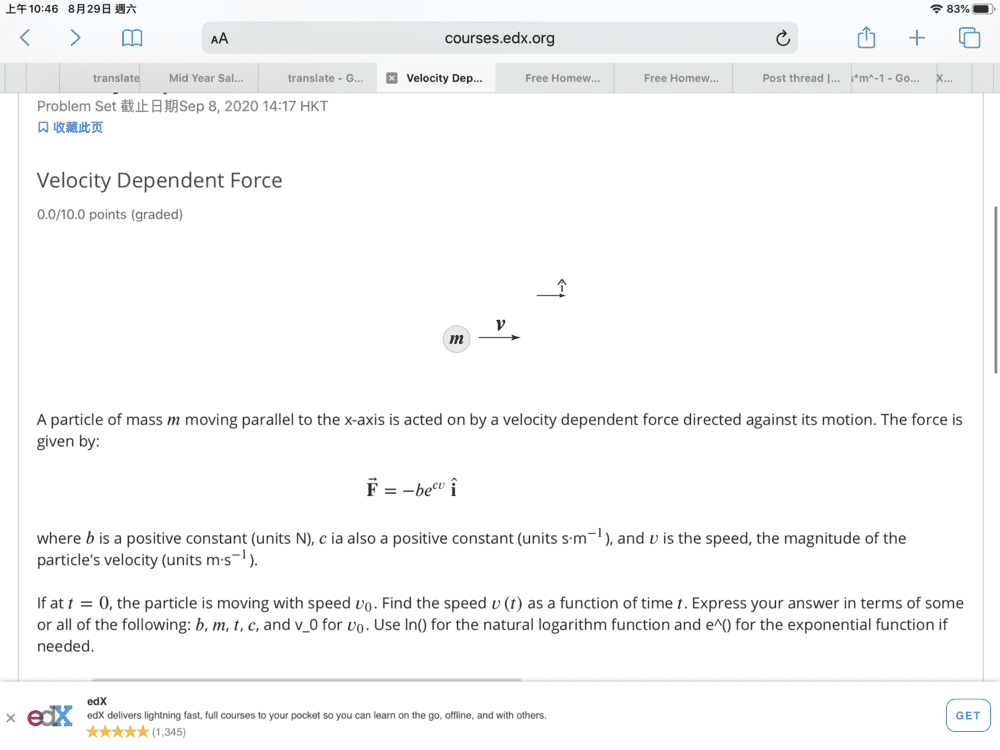
A particle of mass 𝑚 moving parallel to the x-axis is acted on by a velocity dependent force directed against its motion. The force is given by:
| 𝐅⃗=−𝑏𝑒^(𝑐𝑣)𝐢̂ |
If at 𝑡=0, the particle is moving with speed 𝑣0. Find the speed 𝑣(𝑡) as a function of time 𝑡. Express your answer in terms of some or all of the following: 𝑏, 𝑚, 𝑡, 𝑐, and v_0 for 𝑣0. Use ln() for the natural logarithm function and e^() for the exponential function if needed.
This problem I can’t reach the solution have two main reason, the first is , I get too few knowledge of exponential equation (mathematical problem) and I still feel confuse about the equation of how to derive it with an exponential element in the equation in order to get the differential equation and substitute the v(t).
Last edited: