How do I know that the angular acceleration is the same for both wheels?

[simphys]

Homework Statement

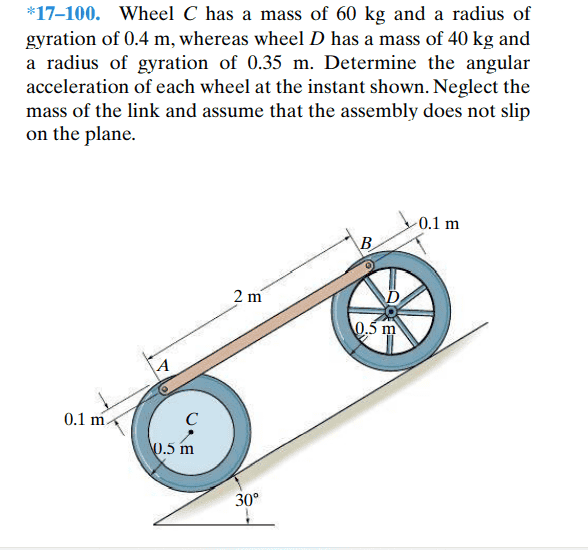
Wheel C has a mass of 60 kg and a radius of
gyration of 0.4 m, whereas wheel D has a mass of 40 kg and
a radius of gyration of 0.35 m. Determine the angular
acceleration of each wheel at the instant shown. Neglect the
mass of the link and assume that the assembly does not slip
on the plane

Relevant Equations

ok

how do I know that both angular accelerations are the same for both wheels here? should I apply relative motion analysis for the acceleration at A(with $a_x,A and a_y,A$) and B(with $a_{x,B} and a_{y,B}$) here, or is just a_A=r*alpha_C and a_B = r*alpha_D enough from which a_A=a_B and thus both have the same acceleration. Thanks in advance

 

[Halc]

how do I know that both angular accelerations are the same for both wheels here?

The wheels are linked, same as if the rod connected via the axles, but this is more of a locomotive link. Point is, it forces both wheels to rotate in sync and thus have identical angular acceleration. Were they not linked, the rear one (D) would accelerate faster.
Calculation of that angular acceleration rate involves the total mass, total moment, and slope angle.

 

[Lnewqban]

...
how do I know that both angular accelerations are the same for both wheels here?...

How would you define angular acceleration for each wheel?

 

[simphys]

How would you define angular acceleration for each wheel?

Change of angular velocity over dt, but what i meant was, if I where to actually show that the angular velocities are the same, can I say that a_A=0.9 x alpha_C due to rolling without slip, or is that only for the acceleration at the COG

 

[Lnewqban]

Change of angular velocity over dt, but what i meant was, if I where to actually show that the angular velocities are the same, can I say that a_A=0.9 x alpha_C due to rolling without slip, or is that only for the acceleration at the COG

I don’t know exactly what kind of answer is expected from you, but the following may help you.

Let’s forget about accelerated movement for a second.
For the shown mechanism to work beyond a fraction of one turn, the tangential velocity of point A and point B must be identical, not only in magnitude but also in direction at any instant.

In order to achieve the above (regardless constant or for accelerated rotational speed), the wheels must have the same radii, as well as both points, and the linkage length or linear distance between A and B must be equal to the distance between the centers of the non-slipping wheels.

The surface, the wheels and the link form what is called a parallelogram four-bar linkage.

Please, see:
https://en.m.wikipedia.org/wiki/Four-bar_linkage#Classification

 

[Steve4Physics]

if I where to actually show that the angular velocities are the same,

There's nothing to show. You can simply state that the angular accelerations must be equal. It should be clear from the geometry - look at @Lnewqban's excellent animation.

(At any instant the angular velocities and accelerations of A and B must be equal - though that doesn't mean their values are necessarily constant during each rotation.)

can I say that a_A=0.9 x alpha_C due to rolling without slip, or is that only for the acceleration at the COG

The 'trick' is to realise that the link could be in tension, compression or unstressed. For the orientation shown, you can draw free body diagrams of A and B and set up some equations. I'd guess it then involves some messy algebra/arithmetic.

[Minor edits made.]

 

[anuttarasammyak]

Just to sketch the system, let us state conservation of energy with no slip, i.e.
\frac{1}{2}(M r^2 +I )(\frac{d\theta}{dt})^2 + Mg(h-h_0)=0
where
h_0-h=r\theta sin\phi
M=M_C+M_D
I=I_C+I_D
r is radius of wheel. h_0 is height of some point of assembly when it is at rest initially. h is height of that point at time t. $\phi$ is angle of slope. $\theta$ is wheels rotation angle which is zero initially. The equation has a form of
(\frac{d\theta}{dt})^2=2a\theta
where a is constant angular acceleration.

 

[simphys]

Just to sketch the system, let us state conservation of energy with no slip, i.e.
\frac{1}{2}(M r^2 +I )(\frac{d\theta}{dt})^2 + Mg(h-h_0)=0
where
h_0-h=r\theta sin\phi
M=M_C+M_D
I=I_C+I_D
r is radius of wheel. h_0 is height of some point of assembly when it is at rest initially. h is height of that point at time t. $\phi$ is angle of slope. $\theta$ is wheels rotation angle which is zero initially. The equation has a form of
(\frac{d\theta}{dt})^2=2a\theta
where a is constant angular acceleration.

There's nothing to show. You can simply state that the angular accelerations must be equal. It should be clear from the geometry - look at @Lnewqban's excellent animation.

(At any instant the angular velocities and accelerations of A and B must be equal - though that doesn't mean their values are necessarily constant during each rotation.)The 'trick' is to realise that the link could be in tension, compression or unstressed. For the orientation shown, you can draw free body diagrams of A and B and set up some equations. I'd guess it then involves some messy algebra/arithmetic.

[Minor edits made.]

I don’t know exactly what kind of answer is expected from you, but the following may help you.

Let’s forget about accelerated movement for a second.
For the shown mechanism to work beyond a fraction of one turn, the tangential velocity of point A and point B must be identical, not only in magnitude but also in direction at any instant.

In order to achieve the above (regardless constant or for accelerated rotational speed), the wheels must have the same radii, as well as both points, and the linkage length or linear distance between A and B must be equal to the distance between the centers of the non-slipping wheels.

The surface, the wheels and the link form what is called a parallelogram four-bar linkage.

Please, see:
https://en.m.wikipedia.org/wiki/Four-bar_linkage#Classification

View attachment 319742

OH I see, thank guys I get it now! .. Had a bit of difficulty 'actually' visualizing it.
And I have a related question to this one actually, one that problem from a couple days ago, which I still haven't figured out. If we look at this structure, will the link AB also undergo translation due to the fact that link AC and link BD are at the same height or will it have an angular acceleration? I think it'll only translate, but I can't really visualize it for sure.
Thanks in advance.

 

[Steve4Physics]

OH I see, thank guys I get it now! .. Had a bit of difficulty 'actually' visualizing it.
And I have a related question to this one actually, one that problem from a couple days ago, which I still haven't figured out.

Ideally, a different question should be in its own thread. The risk is that you now get interleaved replies to 2 different questions - causing great confusion!

If we look at this structure, will the link AB also undergo translation due to the fact that link AC and link BD are at the same height or will it have an angular acceleration? I think it'll only translate, but I can't really visualize it for sure.
Thanks in advance.
View attachment 319757

Please ignore the deleted comment below. I now believe the question is only about the system at a particular instant in time when the configuration is as shown in the diagram. My deleted comment is inapplicable because AC and BD would not have both rotated through 90º in the same time.

To help 'visualisation' it sometimes helps to consider an extreme case, e.g. suppose:
Link AB (4m long) is removed.
AC rotates 90º clockwise about C.
BD rotates the same angle, 90º clockwise, about D.
Is the line AB still horizontal? Is the distance between A and B still be 4m?

 

[Lnewqban]

Can you see the following?
Point B will “drop” faster than point A.
Link AB will have a clockwise rotation respect to A or B.

 

[simphys]

Ideally, a different question should be in its own thread. The risk is that you now get interleaved replies to 2 different qestions - causing great confusion!To help 'visualisation' it sometimes helps to consider an extreme case, e.g. suppose:
Link AB (4m long) is removed.
AC rotates 90º clockwise about C.
BD rotates the same angle, 90º clockwise, about D.
Is the line AB still horizontal? Is the distance between A and B still be 4m?

it'll 'rotate' about A clockwise, and yes my apologies.

Can you see the following?
Point B will “drop” faster than point A.
Link AB will have a clockwise rotation respect to A or B.

oh.. wait so I'm confused. If I would consider the extreme case of A and B horizontal to the right, I'd say that it rotates about A. But when I look at it by considering that B will drop faster due to the link being smaller than I see a rotation about B clockwise.

 

[Steve4Physics]

it'll 'rotate' about A clockwise, and yes my apologies.

oh.. wait so I'm confused. If I would consider the extreme case of A and B horizontal to the right, I'd say that it rotates about A. But when I look at it by considering that B will drop faster due to the link being smaller than I see a rotation about B clockwise.

Apologies, I think I misunderstood the question. I have updated Post #9.

I now believe that the diagram (in Post #8) only specifies what is happening at a particular instant, when AB’s angular velocity is instantaneously zero (which is why “$\omega = 0$” is specified for AB on the diagram!).

Ath the instant shown on the diagram:
The velocity of point A is $\omega_A r_A = 3 \text {rad/s} \times 2 \text{m} = 6$ m/s horizontally to the right
The velocity of point B is $\omega_B r_B = 6 \text {rad/s} \times 1 \text {m} = 6$ m/s horizontally to the right.

So at the instant depicted in the diagram, AB’s instantaneous velocity is 6m/s horizontally to the right and its instantaneous angular velocity is zero.

A short time one or both of the angular velocities must change in order that length AB is maintained at 4m. But that’s not what the question is about.

Well, that’s my interpretation.

 

[simphys]

Apologies, I think I misunderstood the question. I have updated Post #9.

I now believe that the diagram (in Post #8) only specifies what is happening at a particular instant, when AB’s angular velocity is instantaneously zero (which is why “$\omega = 0$” is specified for AB on the diagram!).

Ath the instant shown on the diagram:
The velocity of point A is $\omega_A r_A = 3 \text {rad/s} \times 2 \text{m} = 6$ m/s horizontally to the right
The velocity of point B is $\omega_B r_B = 6 \text {rad/s} \times 1 \text {m} = 6$ m/s horizontally to the right.

So at the instant depicted in the diagram, AB’s instantaneous velocity is 6m/s horizontally to the right and its instantaneous angular velocity is zero.

A short time one or both of the angular velocities must change in order that length AB is maintained at 4m. But that’s not what the question is about.

Well, that’s my interpretation.

well let's put it like that, so on two of the links there's no angular acceleration given. The following is where I had my doubts: So for link AB for the moment equation, the doubt was whether the angular acceleration will also be 0 at that point then or whether I should take it as an unkown. And for link BD it's obvious that there's an angular acceleration (which is another unkown) as there's a force exerted on link AC whilst they're connected

 

[Steve4Physics]

well let's put it like that, so on two of the links there's no angular acceleration given.

No. You are given two of the three instantaneous angular accelerations. (I won’t keep stating ‘instantaneous’ but it is implied.). AC’s angular acceleration is given as 2rad/s² clockwise about C. AB’s angular acceleration is given as 0.

EDIT - apologies. You are correct. I'm carelessly muddling-up angular velocity and angular acceleration. Sorry for the confusion.

Can I suggest you
a) Find the linear acceleration of point A. This gives the linear acceleration of point B.
b) Use this to find the angular acceleration of BD.

You might then want to draw three free body diagrams (one per link) and construct relevant equations.

 

[simphys]

No. You are given two of the three instantaneous angular accelerations. (I won’t keep stating ‘instantaneous’ but it is implied.). AC’s angular acceleration is given as 2rad/s² clockwise about C. AB’s angular acceleration is given as 0.

Can I suggest you
a) Find the linear acceleration of point A. This gives the linear acceleration of point B.
b) Use this to find the angular acceleration of BD.

You might then want to draw three free body diagrams (one per link) and construct relevant equations.

Hello that is indeed what I did. I had 9 unkowns, 5 forces with 5 eqn's of motion from the 3 bodies and the rest from the kinematics. but.. the thing is. I took AB's angular acceleration as an unknown and I indeed got a nonzero value. And it says in the picture that only the angular velocity is 0, so howcome can I then conclude that its' angular acceleration is also zero? (my initial question was similar)

 

[Steve4Physics]

Sorry. My mistake (Instantaneous) angular velocity = 0 certainly does not require that angular acceleration = 0.

I'll give it some further thought!

 

[simphys]

Sorry. My mistake (Instantaneous) angular velocity = 0 certainly does not require that angular acceleration = 0.

I'll give it some further thought!

thanks a lot! but I think you were totally correct in your previous comment. :) Knowing that in the extreme cases it's not horizontal anymore gives us an indication that there actually is an angular acceleration for the middle link.

 

[Lnewqban]

oh.. wait so I'm confused. If I would consider the extreme case of A and B horizontal to the right, I'd say that it rotates about A. But when I look at it by considering that B will drop faster due to the link being smaller than I see a rotation about B clockwise.

At the very instant that the posted diagram shows, the tangential velocities of A and B must be identical, not only in magnitude but in direction.
An instant later, the geometry makes that equality disappear.

The reason is that the directions of both tangential velocities begin to diverge, as each must keep perpendicular to each rotating link AC and BD.

We then have two velocity vectors at the ends of link AB, with a distance separating their lines of action, and the movement of that link respect to the ground of fixed pivots C and D must show some rotation.

 

[Steve4Physics]

Further thoughts...

The problem is to write equations which allow $F$ to be found. Note that $F$ acts in the +x direction.
We can resolve the reaction forces at A and B into x and y components. Only the y-components can cause AB to have angular acceleration about AB’s centre of mass. And these y-components contribute zero moment to AC and BD.

It should be possible to construct a set of equations which only use x-direction forces. This means the y-components - and therefore any angular acceleration of AB - can be ignored.

The two forces determining AC’s angular acceleration are $F$ and the x-component of the reaction at A; call it $R_{Ax}$.

The single force determining DB’s angular acceleration is the x-component of the reaction at B; call it $R_{Bx}$.

The two forces determining AB’s linear x-acceleration are $R_{Ax}$ and $R_{Bx}$ (well, technically the Newtpn's 3rd law partners of these).

The angular acceleration of DB and the linear acceleration of AB can be found from the data (see Post #12). You should then end up with three equations and three unknowns: $F, R_{Ax}$ and $R_{Bx}$.

 

[anuttarasammyak]

And I have a related question to this one actually, one that problem from a couple days ago, which I still haven't figured out. If we look at this structure, will the link AB also undergo translation due to the fact that link AC and link BD are at the same height or will it have an angular acceleration? I think it'll only translate, but I can't really visualize it for sure.
Thanks in advance.

On xy Cartesian coordinate
C(0,0)\ 　D(4,1)
A(2\cos\theta, 2\sin\theta)
B (\cos\phi+4, \sin\phi+1)
AB^2=16=(\cos\phi+4-2\cos \theta)^2+(\sin\phi+1-2\sin\theta)^2
where
\theta_0=\phi_0=\frac{\pi}{2}
initially. This equation of geometry should provide the relation between $\theta$ and $\phi$ during the motion. From this I observe
\dot{\phi}_0=2\dot{\theta}_0
\ddot{\phi}_0=2\ddot{\theta}_0
if I do not fail.