How Does Magnetic Force Affect a Falling Coil's Velocity and Kinetic Energy?

[jaumzaum]

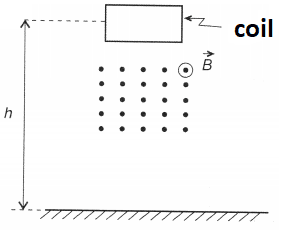
Consider a coil perpendicular to the ground falling with gravity. Under it, there is a magnetic field also perpendicular to the coil. When the coil starts penetrating the magnetic field there will be an induced current and therefore a magnetic force upwards. This magnetic force will reduce coil's acceleration. The same will happen when the coil is exiting the field. The coil will hit the ground, therefore, with a velocity smaller than $\sqrt(2gh)$. As the magnetic foce is upwards and the electrons are moving with a velocity that has a component downwards (same direction as the force), I would conclude that the magnetic force is doing work, negative work, and becausse of that the kinetic energy is reducing. But everyone says magnetic force does not do work, so what's really happening?

 

[Merlin3189]

I don't like to enter into the work question, but we know where all the energy is, still in the coil.
It starts with gravitational PE, converts that to KE and the magnetic field causes some of that to be converted to heat from the induced current flow.

 

[etotheipi]

The magnetic field never does work because its power $P = q (\mathbf{v} \times \mathbf{B}) \cdot \mathbf{v} = 0$. But clearly, in your example, negative work is being done on the coil. The work done on the coil is not due to to the external magnetic field but rather internal forces exerted by charge carriers on the coil.

I know hardly anything about this, however, so best take this with a grain of salt until someone else can provide good justification!

 

[Delta2]

Check this thread https://www.physicsforums.com/threads/the-work-of-magnetic-force-on-the-wire.966793/#post-6137766
In sort it is about as @etotheipi states it, the work done on the coil is not by the magnetic field but by internal forces between free electrons and surface charges. Magnetic field plays an important role in the generation of the surface charges but it is not the one that does the work.

 

[Delta2]

The core of your misunderstanding lies at this sentence:

As the magnetic foce is upwards and the electrons are moving with a velocity that has a component downwards (same direction as the force), I would conclude that the magnetic force is doing work...

By what you call as "magnetic force" I believe you mean the macroscopic manifestation of the Lorentz force ($\vec{F_L}=q(\vec{v}\times\vec{B})$), usually called Laplace force or just "BiL" force $F=\vec{B}I\times\vec{L}$ force in many textbooks, is not actually a magnetic force but an electric force. You have got to read my post linked above and the post in the subsequent link to understand why.

To sum it up, the Lorentz force is a magnetic force, but its macroscopic manifestation, the Laplace force , is an electric force (even though magnetic field vector appears in its formula and not electric field vector).

 

[vanhees71]

The missing energy is due to the generation of heat by the friction of the electrons in the wire, aka Ohmic resistance. The corresponding current is induced by the magnetic field, but the Lorentz force itself doesn't do work for the reasons given in #5.

 

[Delta2]

The missing energy is due to the generation of heat by the friction of the electrons in the wire, aka Ohmic resistance. The corresponding current is induced by the magnetic field, but the Lorentz force itself doesn't do work for the reasons given in #5.

Or if the coil doesn't have ohmic resistance the missing kinetic energy is stored as magnetic field energy in the interior of the coil.

 

[jaumzaum]

Thanks you everybody! @Delta2, I read your post, very helpful! So, let's see if I understood it properly. Consider the image below of a wire conducting a current. There is a voltage difference V in the wire so that the current is to the right and there is also a magnetic field pointing into the paper:

As the wire is a conductor, there are a lot of free electrons in it. These electrons move freely, so I will consider them to be a separate body. The electrons are the only charges who are actually moving, so only on them there is a magnetic force upwards $Fm=qvB$. The Hall effect will make the electrons go up and a positive vacancy be created in the bottom. As the positive vacancy is not moving, and is not free, there is no magnetic force being made on it, and we will also consider it to be part of the wire body. The Hall force created is equal to $Fe$. By the equilibrium of forces in the electron (considering electron mass is almost zero) $N=Fm-Fe$, so the net force in the wire body is $N+Fe=Fm$. Until now, did I understand everything properly?

Ok, now my real problem is when I try to see who is doing work. I understand that, if we consider the above forces to be the only forces acting in the wire, there will be an acceleration upwards and a net work being done. By what I think I understood, the force doing the work is N? What is the nature of that force?

Also, when the electrons move upwards due to Hall effect, it seems to be a work being done on them (in this case by the magnetic force that is upwards and the trajectories that seem to be upwards too), I can think of an explanation where the electrons move in a circular path as when we study a single charged particle in a magnetic field, and the work is zero because the magnetic force is acting as centripetal (is it true?).

What when the electrons get to their final vertical position relative to the wire, in the top of it, and the wire starts to accelerate upwards because of N? The electrons will also accelerate upwards. Will the magnetic force on the electrons continue to act as centripetal? How? In that case, what will be the trajectory of the wire (will it accelerate upwards in a straight line or will the wire starts to rotate)?

I'm asking this because the velocity of the electrons in the vertical plane will be much higher than the drift velocity in the horizontal plane. So there will be another "Hall effect" on the electrons where magnetic field will produce a force to thhe right. If the wire accelerate upwards in a straight line this effect will effectively reduce V. We could calculate the force acting on the electrons because of V. This would be $Ve/d$ where d in the length of the wire. The magnetic force acting on the electrons when they are accelerating upwards is evB. If we consider V=1V, d=10m, B=0,01T and v=10m/s (I think these values can happen in real life) we would zero net force effectively stopping the current. Is this true?

 

[Delta2]

Thanks you everybody! @Delta2, I read your post, very helpful! So, let's see if I understood it properly. Consider the image below of a wire conducting a current. There is a voltage difference V in the wire so that the current is to the right and there is also a magnetic field pointing into the paper:
View attachment 263004

As the wire is a conductor, there are a lot of free electrons in it. These electrons move freely, so I will consider them to be a separate body. The electrons are the only charges who are actually moving, so only on them there is a magnetic force upwards $Fm=qvB$. The Hall effect will make the electrons go up and a positive vacancy be created in the bottom. As the positive vacancy is not moving, and is not free, there is no magnetic force being made on it, and we will also consider it to be part of the wire body. The Hall force created is equal to $Fe$. By the equilibrium of forces in the electron (considering electron mass is almost zero) $N=Fm-Fe$, so the net force in the wire body is $N+Fe=Fm$.Until now, did I understand everything properly?

Unfortunately you still have some vital misunderstanding. (Your figure is not exactly correct, I wish I could do my own figure to show you exactly what I mean, but I am not good in using drawing programs, so I ll just have to use words to explain you the whole thing).

First all in my analysis I took that the wire doesn't move as a rigid body (otherwise there would be motional EMF, besides the Hall voltage and that would complicate things).

Now, the hall voltage has an associated electric field $E_{Hall}$. The sources of this field are the surface charges that are located in the upper and lower side of the conducting wire (if I could do a figure I would draw ----- minus charges in the upper portion of wire and ++++ charges in the lower portion of wire (as you did) and with the free electrons being in the middle). These surface charges are "created " during the mini-transient state during which the Lorentz force $e(\vec{v_{drift}}\times \vec{B})$ is unbalanced by the electric force $eE_{Hall}$. But soon we reach equilibrium and we ll have $e\vec{E_{hall}}=e(\vec{v_{drift}}\times \vec{B})$. So in a free electron and once the equilibrium has being reached,we ll have those two forces (the electric force due to $E_{Hall}$ and the magnetic Lorentz force) that are equal and opposite in direction. So $e E_{Hall}$ is the electric force exerted on a free electron from the hall field, or to be more precise from the afore mentioned surface charges. Therefore by Newton's 3rd Law the free electron exerts on the surface charges, a force that is equal and opposite to $eE_{Hall}=e(\vec{v_{drift}}\times \vec{B})$. If we do the math, we ll find out that the sum of these reaction forces (which are essentially coulomb electric forces) from all the free electrons let's call this sum force $\vec{N}$ (because it is a sum of reaction forces), is equal to $I(\vec{B}\times\vec{L})$ which is the well known BiL force or Laplace force. It should be clear by now that N is an electric force
If you want more details on how the surface charges are created during the transient state I ll try to post.

Ok, now my real problem is when I try to see who is doing work. I understand that, if we consider the above forces to be the only forces acting in the wire, there will be an acceleration upwards and a net work being done. By what I think I understood, the force doing the work is N? What is the nature of that force?

The work is done by the force N which is an electric force.

Also, when the electrons move upwards due to Hall effect, it seems to be a work being done on them (in this case by the magnetic force that is upwards and the trajectories that seem to be upwards too), I can think of an explanation where the electrons move in a circular path as when we study a single charged particle in a magnetic field, and the work is zero because the magnetic force is acting as centripetal (is it true?).

The magnetic Lorentz force, during the transient state which is left unbalanced by the Hall Force, deflects the free electrons along a circular (or more generally a curved path) path and is always normal to this path so its work is zero. Once the equilibrium has been reached there is no more deflection, the electrons move according to $\vec{v_{drift}}$ like there is no external magnetic field.

What when the electrons get to their final vertical position relative to the wire, in the top of it, and the wire starts to accelerate upwards because of N? The electrons will also accelerate upwards. Will the magnetic force on the electrons continue to act as centripetal? How? In that case, what will be the trajectory of the wire (will it accelerate upwards in a straight line or will the wire starts to rotate)?
I'm asking this because the velocity of the electrons in the vertical plane will be much higher than the drift velocity in the horizontal plane. So there will be another "Hall effect" on the electrons where magnetic field will produce a force to thhe right. If the wire accelerate upwards in a straight line this effect will effectively reduce V. We could calculate the force acting on the electrons because of V. This would be $Ve/d$ where d in the length of the wire. The magnetic force acting on the electrons when they are accelerating upwards is evB. If we consider V=1V, d=10m, B=0,01T and v=10m/s (I think these values can happen in real life) we would zero net force effectively stopping the current. Is this true?

Here you start taking the case where the wire starts moving because of the force N (and indeed it will start moving unless the force N is balanced by an external mechanical force on the rigid wire), so there will be motional EMF, and things get complex because other forces appear to the free electrons which are quantum mechanical in nature. However the magnetic Lorentz force is always perpendicular to the velocity of an electron, therefore its work is always zero, and the work is done by the force N which is essentially coulomb electric force.

 

[jaumzaum]

Thank you very much for that explanation! It's extremely detailed, and now I really got everything I wanted. As a last question, which are these forces that you are referring here?

and things get complex because other forces appear to the free electrons which are quantum mechanical in nature.

Do you know any article that I can read that studies a movement similar to that?

 

[Delta2]

Thank you very much for that explanation! It's extremely detailed, and now I really got everything I wanted. As a last question, which are these forces that you are referring here?

Do you know any article that I can read that studies a movement similar to that?

Nope unfortunately I don't know. We are talking here for the "push" force that the free electrons experience as the lattice of ions starts moving, and they are either due to pauli exclusion principle or due to electromagnetic interactions at a quantum level.

One other thing I feel I should say is that the $\vec{N}$ force mentioned in post #9 is an internal force in the rigid wire and therefore it cannot change the momentum of the rigid wire. Internal forces cannot change the momentum of a system. However they can do work and change the kinetic energy of the system (rigid wire in this case). The change in momentum of the rigid wire is attributed to the sum of the Lorentz forces in each free electron.
So we have here the subtlety that for the change in momentum is responsible an external force, but for the change in kinetic energy is responsible an internal force. The most popular (but mechanical example) of such forces is the friction force in the wheels of a car, and the internal forces in the engine of a car.