Inner product between velocity and acceleration is zero (parametric)

[Lambda96]

Homework Statement

I should show the following $\bigl\langle \dot{\textbf{r}}'(t) ,\ddot{\textbf{r}}'(t) \bigr\rangle=0$

Relevant Equations

none

Hi,

I am having problems with task b

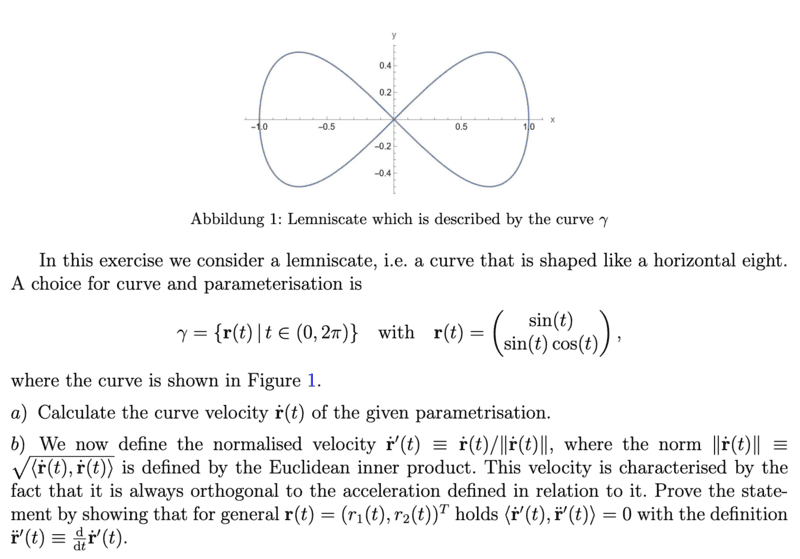
I then defined the velocity vector and the acceleration vector as follows

$dot{\textbf{r}}'(t) = \frac{1}{||\dot{\textbf{r}}(t)||} \left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right)$

and

$ddot{\textbf{r}}'(t) = \frac{1}{||\dot{\textbf{r}}(t)||} \frac{d}{dt}\left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right)$

Then I calculated the following:

$bigl\langle \dot{\textbf{r}}'(t) ,\ddot{\textbf{r}}'(t) \bigr\rangle=\dot{\textbf{r}}'(t) = \frac{1}{||\dot{\textbf{r}}(t)||^2} \left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right) \cdot \frac{d}{dt}\left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right)= \frac{1}{||\dot{\textbf{r}}(t)||^2} \Bigl( \dot{r_1}(t) \frac{d}{dt} \dot{r_1}(t) + \dot{r_2}(t) \frac{d}{dt} \dot{r_2}(t) \Bigr)=\frac{1}{||\dot{\textbf{r}}(t)||^2} \Bigl( \dot{r_1}(t) \ddot{r_1}(t) + \dot{r_2}(t) \ddot{r_2}(t) \Bigr)$

Unfortunately, I can't get any further. The following must apply $\bigl\langle \dot{\textbf{r}}'(t) ,\ddot{\textbf{r}}'(t) \bigr\rangle=0$ so that this is the case, the expression $\dot{r_1}(t) \ddot{r_1}(t) + \dot{r_2}(t) \ddot{r_2}(t)=0$, but why is this the case?

My derivation is probably already wrong, but I don't know how else to solve the problem.

 

[Lambda96]

I don't know why my formula with Latex is not displayed correctly, does anyone know why?

In overleaf it is displayed correctly

 

[Hill]

In $$dot{\textbf{r}}'(t) = \frac{1}{||\dot{\textbf{r}}(t)||} \left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right)$$ a "\" is missing in the beginning: $$\dot{\textbf{r}}'(t) = \frac{1}{||\dot{\textbf{r}}(t)||} \left(\begin{array}{c} \dot{r_1}(t) \\ \dot{r_2}(t) \end{array}\right)$$

 

[Orodruin]

ddotr′(t)=1||r˙(t)||ddt(r1˙(t)r2˙(t))

no. The normalized acceleration vector should be the derivative of the normalized velocity vector.

 

[Orodruin]

To add a hint to that, what you are looking for is a property of any vector of constant magnitude. Don’t get bogged down in the particular expression in terms of a normalized vector.

 

[Lambda96]

Thank you Hill and Orodruin for your help

I have now proceeded as follows

$\bigl\langle \dot{r}', \dot{r}' \bigr\rangle=\dot{r}' \cdot \dot{r}' =1$

Now I have simply formed the derivative $\frac{d}{dt}$

$\ddot{r}' \cdot \dot{r}' + \dot{r}' \cdot \ddot{r}' =0$
$2 \dot{r}' \cdot \ddot{r}' =0$
$\dot{r}' \cdot \ddot{r}' =0$

 

[Orodruin]

Thank you Hill and Orodruin for your help

I have now proceeded as follows

$\bigl\langle \dot{r}', \dot{r}' \bigr\rangle=\dot{r}' \cdot \dot{r}' =1$

Now I have simply formed the derivative $\frac{d}{dt}$

$\ddot{r}' \cdot \dot{r}' + \dot{r}' \cdot \ddot{r}' =0$
$2 \dot{r}' \cdot \ddot{r}' =0$
$\dot{r}' \cdot \ddot{r}' =0$

Indeed.