- #36
Hak
- 709
- 56
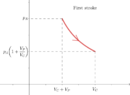
I'm getting:TSny said:That should be easy. It's very similar to the first stroke of the small pump.
$$W_2=P_{atm}(V_C+ 4V_p)\ln{\frac{V_C}{V_C+4V_p}}$$.
Is that correct?