- #36
inuk2600
- 17
- 3
sophiecentaur said:Do you have any other suggestions?
That's all I got.
sophiecentaur said:Do you have any other suggestions?
Since the star and occulting object is so far away, you can assume the light rays coming from the disc of the star to be parallel. The percentage of luminosity dip caused by the occulting object is then just the percentage of the disc of the star that is covered by the object. In other words, the dip is proportional to the ratio of the cross-sectional areas of the object and the star.Lanniakea said:Can someone point me towards the mathematics behind estimating the size of an object from the dip in light curve? I read somewhere (I can't remember for the life of me where) that if the object blocking this star was the size of Jupiter, the dip in flux would have been less than 1%. This is a periodic dip of 15-22%, which is insane if correct. Can anyone verify these estimations?
Bandersnatch said:Since the star and occulting object is so far away, you can assume the light rays coming from the disc of the star to be parallel. The percentage of luminosity dip caused by the occulting object is then just the percentage of the disc of the star that is covered by the object. In other words, the dip is proportional to the ratio of the cross-sectional areas of the object and the star.
You know how Jupiter is roughly 10 times smaller than the Sun by radius? Compare the area of a circle with radius 1/10 R (Jupiter) with the area of a circle with radius R (Sun):
##\frac{A_{planet}}{A_{star}}##
##\frac{\pi (0.1R_{Sun})^2}{\pi R_{Sun}^2}=0.01##
Or 1%. The star being the subject of this discussion is larger than the Sun, so a Jupiter-sized planet would occult less than the 1%. To calculate how much less, do the same calculation as above, only with the radius of the star equal to 1.58 R.
newjerseyrunner said:@Lanniakea that's why it's more likely a fairly small object (in terms of mass.) If it were huge, we'd also see the star getting pulled around by it's gravity.
Dense objects like planets have small radii, defuse objects can be much much larger. If you were looking at our solar system, the widest object isn't Jupiter, it's Saturn. A comet approaching the sun's coma also can be significantly wider than Jupiter.
I wonder if a large moon or plutoid being pushed inward could create the affect. What would happen if a fairly large stray object (rogue planet) passed close enough to Jupiter to pull one of it's moon away. Would it act like a short term comet? I imagine if a comet 50 miles across can create a coma thousands of miles across, an icy object 500 miles across could make a mind bogglingly huge coma.
The distance doesn't contribute, because the thing you're looking at is so far away.Lanniakea said:My only question is, how much would the distance from the star contribute? For example let's say Jupiter was orbiting this star at 2 AU away, would it not theoretically block more light from our perspective if it were say 8 AU away?
Well, equivalent to. As a cloud of smaller objects, it won't be and object of that radius.Lanniakea said:In the paper they say a broken up exocomet is the most likely scenario of all they considered, and I with my completely unprofessional opinion agree, a broken up/smeared out object seems to be by far the most likely explanation, but 61-74% solar radius? Wow
EDIT: The 4th biggest planet I mentioned is basically a big ball of gas that is speculated to have a huge tail and hilariously low density. I don't see what density has to do with anything here?
Minimum?DaveC426913 said:Let's start with a basic ideal shape. Let's say the cloud is entirely contained within the sun's disc, and let's say the cloud it is spherical. What is the minimum mass of the cloud to occlude 22% of the sun's disc?
You stopped reading halfway though, didn't you?Bandersnatch said:Minimum?
Then you need only one particle in a whole cloud per line of sight. That is, you can compress the spherical cloud of particles into a solid disc, with thickness equal to the size of the particles (assuming they're uniform).
We'd have to assume some typical shape to the cloud. (If it were a flat disk, seen face-on, like a wall then you'd need far smaller mass, but that's not a plausible shape)
I kinda didDaveC426913 said:You stopped reading halfway though, didn't you?
Bandersnatch said:The distance doesn't contribute, because the thing you're looking at is so far away.
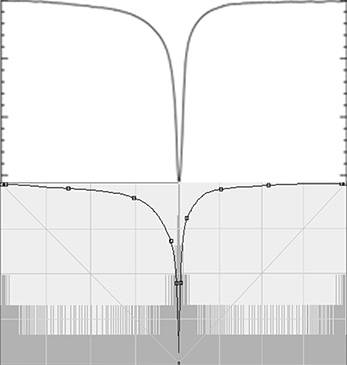
If you were to draw light rays coming to your eye from the edges of an object, you'd get something like this:
View attachment 90551
If you place an object between the source and the observer, how much light reaches the observer will depend on the proportion of the distance between the two lines that the object obscures.
Now imagine the distance d increases to an absurdly large value as compared to the size of the object being observed (here, ~1500 light-years vs 1.58 Sun radii). The angle alpha becomes very small, and the lines of light rays become indistinguishable from parallel on the scale of a stellar system. So whether the object is 1 AU or 1 ly from the star, the distance between the lines of light rays is practically unchanged, and the same amount of light gets blocked.

You're still missing the point. We have to assume a plausible shape to the dust cloud. Flat discs (whose plane is tangential to the star) are impossible, and no such shape will give us any first order approximation of the mass required. Reject it.Bandersnatch said:I kinda did, but then I read again and realized that editing is unnecessary as the answer is still correct
Ignorant question: what is a sol? A solar diameter?DaveC426913 said:You're still missing the point. We have to assume a plausible shape to the dust cloud. Flat discs (whose plane is tangential to the star) are impossible, and no such shape will give us any first order approximation of the mass required. Reject it.
Let's assume a spherical cloud. So a sphere of diameter 1.58 Sols. (This too is not a likely shape, but it'll be a lot closer.)
Simpler. A Sol is simply our sun.newjerseyrunner said:Ignorant question: what is a sol? A solar diameter?
Pretty sure migration happens over astronomical times scales, i.e. tens of millions of years.newjerseyrunner said:I have a question: how does a big planet migrate inward? I know that many of the first exoplanets discovered were gas giants orbiting very closely to their star, to close to have formed there. Do they migrate in slowly or does it happen in a few well timed gravitational tugs? If gas giant with icy moons migrates inwards, wouldn't all of those moon start to sublimate all at once and produce a monstrous cloud / ring system? If the planet started out on it's side like Uranus, wouldn't it create a flat disc perpendicular to the planet's orbit?
DaveC426913 said:You're still missing the point. We have to assume a plausible shape to the dust cloud. Flat discs (whose plane is tangential to the star) are impossible, and no such shape will give us any first order approximation of the mass required. Reject it.
Let's assume a spherical cloud. So a sphere of diameter 1.58 Sols. (This too is not a likely shape, but it'll be a lot closer.)
I think you might be missing the point, though. I did not say that the cloud is shaped like a disc. What I was saying, is that a maximally-diffuse spherical cloud obscures as much light as a compact disc of the same mass.DaveC426913 said:You're still missing the point. We have to assume a plausible shape to the dust cloud. Flat discs (whose plane is tangential to the star) are impossible, and no such shape will give us any first order approximation of the mass required. Reject it.
Let's assume a spherical cloud. So a sphere of diameter 1.58 Sols. (This too is not a likely shape, but it'll be a lot closer.)
My understanding is that the object has to be cold, and can not have resulted from a planetary catastrophe.Artribution said:It seems a disintegrating planet shouldn't create a spherical cloud, though. It should be a long, diffuse trailing cloud - a ring fragment. Like when moons and asteroids disintegrate around planets. You get a ring of debris, not a spherical cloud.
On the other hand, what if the disintegrating planet has moons that survive? Then they could act like shepherd moons, distorting or constraining the shape of the cloud between them. Or they would begin gravitating toward each other, pulling a portion of the cloud into a blob that will eventually form a new planet.
For that matter, could this just be a planet that hasn't finished forming yet?
Agreed, but we've no way of determining that.Artribution said:It seems a disintegrating planet shouldn't create a spherical cloud, though. It should be a long, diffuse trailing cloud - a ring fragment. Like when moons and asteroids disintegrate around planets. You get a ring of debris, not a spherical cloud.
Hm. I see your point.Bandersnatch said:I think you might be missing the point, though. I did not say that the cloud is shaped like a disc. What I was saying, is that a maximally-diffuse spherical cloud obscures as much light as a compact disc of the same mass.
If, for a given luminosity dip, you assume a solid sphere on one end, it'll provide you with the maximum mass boundary. If, on the other end, you assume a sphere so diffuse that no two particles are on the same line of sight, you get the minimum mass boundary (for spherical distributions).
It might turn out that the maximally-diffuse cloud would have to be larger than the disc of the star, which would provide a correction to the minimum mass.
In any case the actual cloud will be somewhere in-between these two, but where exactly would have to be deduced from the shape of the curve, which is something I've no idea how to do.
newjerseyrunner said:What type of technology is required for us to figure out what the object is made out of? Isn't it possible to take spectral readings from planets around other stars? If it's diffuse, some light would shine through and allow us to determine what it is? If the most likely candidate is water ice, shouldn't it be fairly easy to check?
Pretty sure NJR was thinking of chemical spectro analysis.Artribution said:I don't know, but if it can't be observed directly, then it should be possible to narrow down the possibilities using computer simulations.
It's been noted that the curve is not symmetrical, but what do you mean by diffuse on the sides?Artribution said: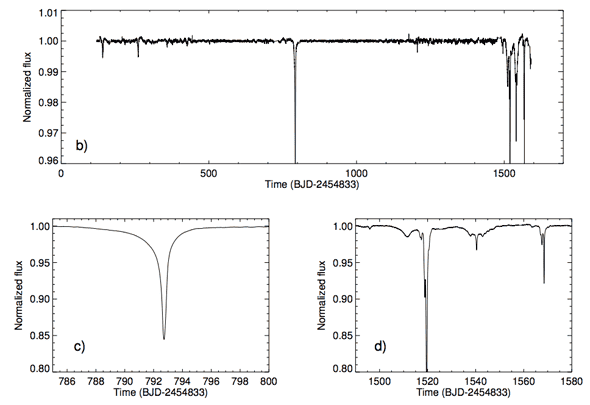
I noticed a couple of things about that light curve. First: it's not symmetrical. One side is steeper than the other. Second: It's diffuse on the sides but sharp in the middle. That's the opposite of what an exoplanet transit tends to look like:
DaveC426913 said:It's been noted that the curve is not symmetrical, but what do you mean by diffuse on the sides?
Artribution said:In Photoshop, I converted the object's light curve into a gradient curve:
It's not exact, but it gives a rough approximation of what the light curve represents in visual terms:
Debris cloud? Or ships orbiting alongside the ringworld?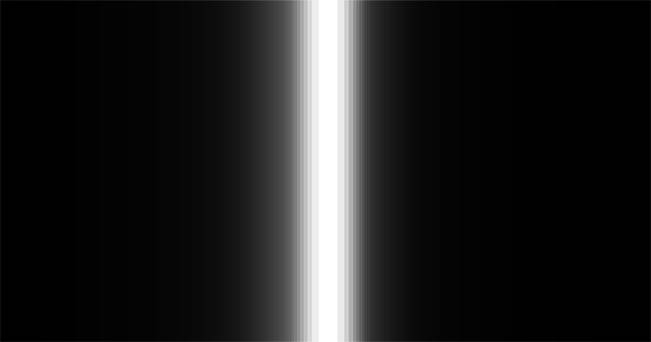
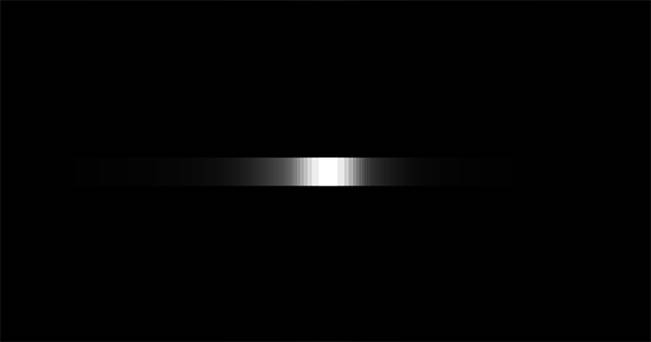
OK. I thought you were suggesting the curve itself was diffuse, as in you were seeing detail in it.Artribution said:The light curves from KIC 8462852 are the opposite: a smooth ingress and egress that instead of leveling out, drop down into a sharp point.
The shape suggests an object surrounded by a more diffuse cloud of matter, which is what it looks like in the gradient curves.
inuk2600 said:I see, so the leading edge is more diffuse than the trailing edge?
None of the considered scenarios require a large mass.RealTwistedTwin said:But as far as I know all the other explanations require huge amounts of masses orbiting the star, so they would surely leave a hint in the spectrum of the star.
A comet coma bigger than the sun. Yes.newjerseyrunner said:Has any comet we've seen in our solar system created comas anywhere near that size?
That doesn't seem to fit where I'm at in my head. I look at it this way...Artribution said:I made a more mathematically accurate gradient: