Manifolds - Charts on Real Projective Spaces

[Math Amateur]

I am reading John M. Lee's book: Introduction to Smooth Manifolds ...

I am focused on Chapter 1: Smooth Manifolds ...

I need some help in fully understanding Example 1.3: Projective Spaces ... ...

Example 1.3 reads as follows:

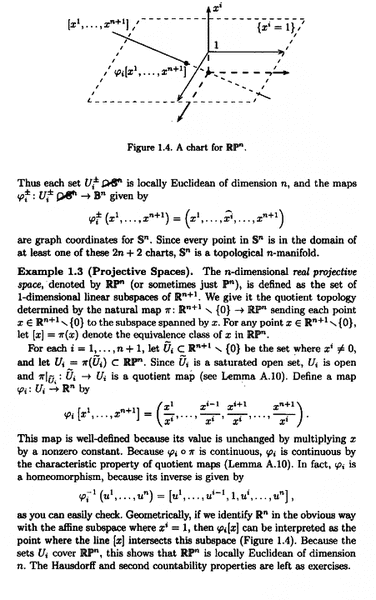My questions are as follows:Question 1In the above example, we read:

" ... ... define a map $\phi_i \ : \ U_i \longrightarrow \mathbb{R}^n$ by$\phi_i [ x^1, \ ... \ ... \ , x^{n+1} ] = ( \frac{x^1}{x^i} , \ ... \ , \frac{x^{i-1}}{x^i} , \frac{x^{i+1}}{x^i}, \ ... \ , \frac{x^{n+1}}{x^i} )$This map is well defined because its value is unchanged by multiplying x by a nonzero constant. ... ... "Now, in the above, the domain of $\phi_i$ is shown as an $(n+1)$-dimensional point ... ... BUT ... ... $\phi_i$ is a map with a domain consisting of lines in $\mathbb{R}^{n + 1}$, so shouldn't the dimension of the domain be $n$ ... ?

Maybe we have to regard the equivalence classes of the quotient topology involved as $(n+1)$-dimensional points and recognise that points $x = \lambda x$ where $\lambda \in \mathbb{R}$ ... ... is that right?

(The statement about the map being well defined is presumably about recognising equivalence classes as on point in the projective space ... ... is that right? ... ...)

==========================================================

Question 2In the above text from Lee's book we read:

"... ... Because $\phi_i \circ \pi$ is continuous ... ... "How do we know that $\phi_i \circ \pi$ is continuous ... ?

============================================================

Question 3

In the above text from Lee's book we read:

"... ... In fact $\phi_i$ is a homeomorphism, because its inverse is given by

${\phi_i}^{-1} [ u^1, \ ... \ ... \ , u^{n} ] = [ u^1, \ ... u^{i-1}, 1, u^i, \ ... \ , u^{n} ]$

as you can easily check ... ... "I cannot see how Lee determined this expression to be the inverse ... why is the inverse of $\phi_i$ of the form shown ... how do we get this expression ... and why is it continuous (as it must be since Lee declares $\phi_i$ to be a homeomorphism ... ...

===========================================================================

Question 4

Just a general question ... in seeking a set of charts to cover $\mathbb{RP}^n$, why does Lee bother with the $\tilde{U_i}$ and $\pi$ ... why not just define the $U_i$ as an open set of $\mathbb{RP}^n$ and define the $\phi_i$ ... ... ?

============================================================================Hope someone can help with the above three questions ...

Help will be appreciated ... ...

Peter

 

[andrewkirk]

I'll do one now (the easiest one, of course), then come back later and have a look at the others.

Question 1In the above example, we read:

" ... ... define a map $\phi_i \ : \ U_i \longrightarrow \mathbb{R}^n$ by$\phi_i [ x^1, \ ... \ ... \ , x^{n+1} ] = ( \frac{x^1}{x^i} , \ ... \ , \frac{x^{i-1}}{x^i} , \frac{x^{i+1}}{x^i}, \ ... \ , \frac{x^{n+1}}{x^i} )$This map is well defined because its value is unchanged by multiplying x by a nonzero constant. ... ... "Now, in the above, the domain of $\phi_i$ is shown as an $(n+1)$-dimensional point ... ... BUT ... ... $\phi_i$ is a map with a domain consisting of lines in $\mathbb{R}^{n + 1}$, so shouldn't the dimension of the domain be $n$ ... ?

The operand of $\phi_i$ is $[ x^1, \ ... \ ... \ , x^{n+1} ]$, which is not an $(n+1)$-dimensional point but an equivalence class consisting of all points in $\mathbb{R}^{n+1}$ in the same 1D subspace as $( x^1, \ ... \ ... \ , x^{n+1} )$. That is indicated by the use of square, rather than round brackets. See the last line of the first para of Example 1.3. Taking equivalence classes reduces the dimension from $n+1$ to $n$.

They've abused notation a little, which may have confused you. They should have written $\phi_i\big([(x^1, \ ... \ ... \ , x^{n+1} )]\big)$ not $\phi_i[ x^1, \ ... \ ... \ , x^{n+1} ]$, but they omitted both sets of round brackets.

I get the impression you may have guessed this from what you wrote next, but I wasn't sure what you were getting at there, so I wrote this.

 

[andrewkirk]

Just one more quick one:

Question 2In the above text from Lee's book we read:

"... ... Because $\phi_i \circ \pi$ is continuous ... ... "How do we know that $\phi_i \circ \pi$ is continuous ... ?
Peter

The map $\phi_i\circ\pi$ is much simpler than it looks, because the composition of the two removes most of the complexity involved in taking quotients. What the composed map does is simply (1) divide all components by the $i$th component, which we know to be nonzero, then (2) drop the $i$th component. Both of these steps are easily shown to be continuous by epsilon-delta arguments, and the composition of continuous functions - in this case the functions representing step 1 and step 2, not $\phi_i$ and $\pi$ - is continuous.

 

[Math Amateur]

I'll do one now (the easiest one, of course), then come back later and have a look at the others.

The operand of $\phi_i$ is $[ x^1, \ ... \ ... \ , x^{n+1} ]$, which is not an $(n+1)$-dimensional point but an equivalence class consisting of all points in $\mathbb{R}^{n+1}$ in the same 1D subspace as $( x^1, \ ... \ ... \ , x^{n+1} )$. That is indicated by the use of square, rather than round brackets. See the last line of the first para of Example 1.3. Taking equivalence classes reduces the dimension from $n+1$ to $n$.

They've abused notation a little, which may have confused you. They should have written $\phi_i\big([(x^1, \ ... \ ... \ , x^{n+1} )]\big)$ not $\phi_i[ x^1, \ ... \ ... \ , x^{n+1} ]$, but they omitted both sets of round brackets.

I get the impression you may have guessed this from what you wrote next, but I wasn't sure what you were getting at there, so I wrote this.

Thanks for the help, Andrew

Peter

 

[Math Amateur]

Just one more quick one:
The map $\phi_i\circ\pi$ is much simpler than it looks, because the composition of the two removes most of the complexity involved in taking quotients. What the composed map does is simply (1) divide all components by the $i$th component, which we know to be nonzero, then (2) drop the $i$th component. Both of these steps are easily shown to be continuous by epsilon-delta arguments, and the composition of continuous functions - in this case the functions representing step 1 and step 2, not $\phi_i$ and $\pi$ - is continuous.

Thanks again Andrew

Peter

 

[andrewkirk]

Question 3

In the above text from Lee's book we read:

"... ... In fact $\phi_i$ is a homeomorphism, because its inverse is given by

${\phi_i}^{-1} [ u^1, \ ... \ ... \ , u^{n} ] = [ u^1, \ ... u^{i-1}, 1, u^i, \ ... \ , u^{n} ]$

as you can easily check ... ... "I cannot see how Lee determined this expression to be the inverse ... why is the inverse of $\phi_i$ of the form shown ... how do we get this expression ... and why is it continuous (as it must be since Lee declares $\phi_i$ to be a homeomorphism ... ...

Careful now! That's not exactly what Lee wrote.
What he wrote was:
${\phi_i}^{-1} (u^1, \ ... \ ... \ , u^{n}) = [ u^1, \ ... u^{i-1}, 1, u^i, \ ... \ , u^{n} ]$
and what he should have written is:
${\phi_i}^{-1} \big(\ ( u^1, \ ... \ ... \ , u^{n} )\big) = [ u^1, \ ... u^{i-1}, 1, u^i, \ ... \ , u^{n} ]$
The difference between your and his version is somewhat important, because I suspect the inverse function won't work if the element it's operating on is an equivalence class rather than an ordinary old point in $\mathbb{R}^n$.

To check that it's an inverse, you just have to show that
$\phi_i\left({\phi_i}^{-1} \left(\langle u^1, \ ... \ ... \ , u^{n} \rangle\right)\right) =\langle u^1, \ ... \ ... \ , u^{n} \rangle$
and
$\phi_i^{-1}\left({\phi_i}\left(\left[\langle x^1, \ ... \ ... \ , x^{n+1} \rangle\right]\right)\right) = \left[\langle x^1, \ ... \ ... \ , x^{n+1} \rangle\right]$

where I've used angle brackets to delimit points in Euclidean space, to avoid bracket overload.

Just apply the formulas for these two functions that Lee gives above, and these should work out.

For continuity of $\phi_i^{-1}$ it is enough to show that for any open ball $B$ in $\mathbb{R}^n$, $\phi^{-1}(B)$ is open in $\mathbb{RP}^{n}$. To do that, you'll need to think a bit about what open sets in $\mathbb{RP}^n$ look like, which is a useful exercise in itself. I suspect there's a quicker way of proving continuity by using $\pi$, but in this case doing it the long way may have more lasting value.

Good luck!

 

[Math Amateur]

hI Andrew,

Thanks for helping me to make progress on understanding the basics of differential topology ... really appreciate the support ...

Just working on what you suggested ...

Peter

 

[andrewkirk]

Might as well do the last one as well:

Question 4

Just a general question ... in seeking a set of charts to cover $\mathbb{RP}^n$, why does Lee bother with the $\tilde{U_i}$ and $\pi$ ... why not just define the $U_i$ as an open set of $\mathbb{RP}^n$ and define the $\phi_i$ ... ... ?

The function $\pi:\mathbb{R}^{n+1}\smallsetminus\{0\}\to\mathbb{RP}^{n}$ is needed because to create a quotient space you need a quotient map. $\pi$ is that map. The continuity of $\pi$ is used to establish the continuity of each $\phi_i$.

I like the $\tilde{U}_i$ sets because they are easier to visualise than their images $U_i$ in $\mathbb{RP}^{n}$. THey provide an easy way to visualise open sets in $\mathbb{RP}^n$. They may also be used in establishing continuity of various maps.

 

[Math Amateur]

Thanks for your posts Andrew ... they have been most helpful ...

Peter