- 20,683
- 28,041
Summary: countability, topological vector spaces, continuity of linear maps, polynomials, finite fields, function theory, calculus1. Let ##(X,\rho)## be a metric space, and suppose that there exists a sequence ##(f_i)_i## of real-valued continuous functions on ##X## with the property that a Cauchy sequence ##(x_n)_n## is convergent whenever each of the sequences ##(f_i(x_n))_i## is bounded. Then ##X## can be remetrized (with equivalent metrics) so as to be complete.2. Let ##X=C([0,1])## be the topological space of real-valued continuous functions,
$$
\rho(f,g):=\operatorname{sup}_{x\in [0,1]}|f(x)-g(x)|
$$
the uniform metric induced by the ##L^\infty ## norm,
$$
\sigma (f,g):=\int_0^1 |f(x)-g(x)|\,dx
$$
the ##L^1## induced metric, and for n∈N
$$
E_n:=\left\{\left. f\in X\,\right|\,\exists \,x\in \left[0,1-1/n\right]\,\forall \,h\in (0,1-x)\, : \,|f(x+h)-f(x)|\leq nh\right\}.
$$
Show that
$$
p\,|\,\operatorname{card}(V)
$$
8. (solved by @julian ) A linear fractional transformation ##S\, : \,\mathbb{C}_\infty \longrightarrow \mathbb{C}_\infty ## defined by ##S(z)=\dfrac{az+b}{cz+d}## is called a Möbius transformation if ##ad-bc\neq 0##. Here ##S(\infty )=a/c## and ##S(-d/c)=\infty .## Show that Möbius transformations form a group by composition, and that there is a unique Möbius transformation ##S(z)## which takes ##(z_1,z_2,z_3)## to ##(1,0,\infty ).## Which one?9. Let ##G## be a connected open set and let ##f:G\longrightarrow \mathbb{C}## be an analytic function. Show that the following statements are equivalent:
$$
|f(z)+g(z)|<|f(z)|+|g(z)|
$$
on ##\gamma ,## then
$$
Z_f-P_f=Z_g-P_g\;.
$$

High Schoolers only
11. (solved by @Not anonymous ) A gardener holds a water hose horizontally and wants to water a bed ##6\,\rm m## away. The water exits the hose at a speed of ##8\,\rm m/s\,.## Calculate the minimum height the gardener needs to hold the hose for the water to reach the bed, the speed at which the water droplets hit the bed, and the angle at which the water droplets hit the bed.12. (solved by @Not anonymous ) A faucet delivers a volume flow of ##V'=6\,\dfrac{\it l}{\rm min} ##. The connected garden hose has an inner diameter of ##d_1=18\,\rm mm ##, the nozzle a cross-section of ##d_2=5\,\rm mm.## Calculate the mass flow in the garden hose, the speed of the water in the garden hose, and the speed of the water at the nozzle. It is observed that the water jet widens after the nozzle. Why?13. (solved by @Not anonymous and @kshitij ) As a result of the refraction, the light bundle emanating from the slit ##S## produces two bundles that overlap in the screen area of width ##D## and appear to arise from two virtual slit images ##A## and ##B##. Since the two virtual slit images originate from the same slit, the light emanating from them is coherent and can interfere in the area of overlap.
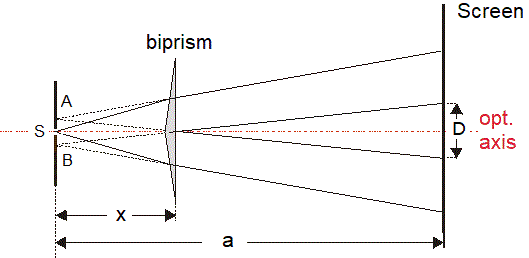 Calculate the wavelength if monochromatic light is used from the quantities given in the sketch and the distance ##\Delta y## between two adjacent interference strips? Assume that the dimensions parallel to the optical axis can be viewed as large compared to those perpendicular to the optical axis.14. (solved by @Not anonymous ) A galaxy is ##42\,\rm MLy## away and oriented in space, such that its rotation axis is perpendicular to the line of sight. The ##\alpha## line of hydrogen is measured to occur at ##\lambda_1=658.003\,\rm nm## instead of ##\lambda _0=656.28 \,\rm nm## widened to ##b=0.438\,\rm nm.##
Calculate the wavelength if monochromatic light is used from the quantities given in the sketch and the distance ##\Delta y## between two adjacent interference strips? Assume that the dimensions parallel to the optical axis can be viewed as large compared to those perpendicular to the optical axis.14. (solved by @Not anonymous ) A galaxy is ##42\,\rm MLy## away and oriented in space, such that its rotation axis is perpendicular to the line of sight. The ##\alpha## line of hydrogen is measured to occur at ##\lambda_1=658.003\,\rm nm## instead of ##\lambda _0=656.28 \,\rm nm## widened to ##b=0.438\,\rm nm.##
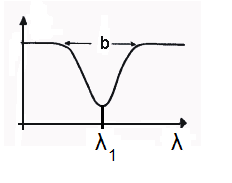
Assume that the main cause of the widening is the rotation of the stars around the center of the galaxy. Assume further that the different wavelength is only due to the radial motion of the galaxy compared to our solar system.
What is the maximal rotational velocity of the observed stars, and what is the maximal velocity the galaxy is moving, and in which direction as seen from our solar system?
15. (solved by @Not anonymous ) The spiral galaxy ##\rm M81## near Ursa Major can already be viewed by a small telescope. It has an apparent magnitude of ##M=6.9.## The angle to the celestial pole is about ##21°.## Is it possible to observe ##\rm M81## the entire year, if you live in Toronto?
The following diagram shows data-points of light from the cepheid ##\rm C27## in ##\rm M81.##
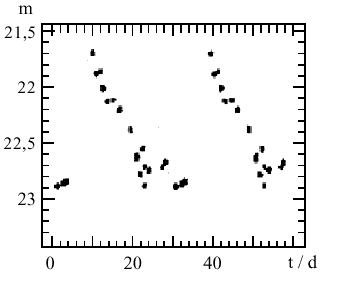
Calculate our distance from ##\rm M81## in lightyears. (Use an average value of magnitude ##22.3## at a pulsation rate of ##30## per day and the relation ##M=-1.67-2.54\cdot\log_{10} p##.)
The second diagram is a comparison between ##\rm M81## and Milky Way. It shows the radial orbit velocity ##v## of the stars in relation to their distance ##r## from the galaxy center. Optical wavelengths are hardly to observe from around ##16\,kpc## on, so radio wavelengths are used.
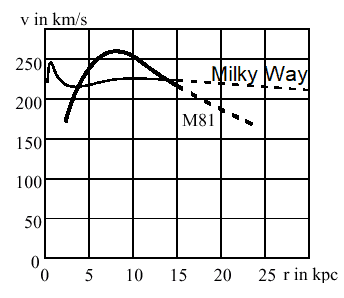
Verify that if a celestial body orbits a center of great mass, then we can calculate the central mass approximately by ##M=\dfrac{v^2\cdot r}{G}.## Show by choosing two data-points that the rotation curve of ##\rm M81## is approximately ##v\sim \dfrac{1}{\sqrt{r}}## for ##r=10\,\rm{kpc}.## What does that mean for the mass distribution in ##\rm M81\,##? Estimate the mass of ##\rm M81## within the optical spectrum in units of sun masses.
The rotation curves of ##\rm M81## and the Milky Way differ a lot for great distances from the center. What does that mean for the mass distribution in our Milky Way?
The wavelength of the ##\alpha ##-line of hydrogen from the optical center of ##\rm M81## is measured to be ##\lambda_1=656.38\,\rm nm## in comparison to ##\lambda_0 =656.47 \,\rm nm.## Can we apply Hubble's law to ##\rm M81##?#
$$
\rho(f,g):=\operatorname{sup}_{x\in [0,1]}|f(x)-g(x)|
$$
the uniform metric induced by the ##L^\infty ## norm,
$$
\sigma (f,g):=\int_0^1 |f(x)-g(x)|\,dx
$$
the ##L^1## induced metric, and for n∈N
$$
E_n:=\left\{\left. f\in X\,\right|\,\exists \,x\in \left[0,1-1/n\right]\,\forall \,h\in (0,1-x)\, : \,|f(x+h)-f(x)|\leq nh\right\}.
$$
Show that
- ##(X,\rho)## is complete.
- ##(X,\rho) \not\cong (X,\sigma)## is not complete.
- ##E_n\subseteq (X,\rho)## is closed.
- For each ##V\in \mathcal{B},## there exists ##U\in \mathcal{B}## such that ##U+U\subseteq V.##
- Every ##V\in \mathcal{B}## is radial (i.e. there exists a ##\lambda_0 \in K## such that whenever ##|\lambda|\geq |\lambda_0| ## we have ##F\subseteq \lambda V## for each finite subset ##F\subseteq L##) and circled (##\lambda V\subseteq V## whenever ##|\lambda |\leq 1##).
- There exists ##\lambda \in K,\,0<|\lambda |<1,## such that ##V\in \mathcal{B}## implies ##\lambda V\in \mathcal{B}.##
$$
p\,|\,\operatorname{card}(V)
$$
8. (solved by @julian ) A linear fractional transformation ##S\, : \,\mathbb{C}_\infty \longrightarrow \mathbb{C}_\infty ## defined by ##S(z)=\dfrac{az+b}{cz+d}## is called a Möbius transformation if ##ad-bc\neq 0##. Here ##S(\infty )=a/c## and ##S(-d/c)=\infty .## Show that Möbius transformations form a group by composition, and that there is a unique Möbius transformation ##S(z)## which takes ##(z_1,z_2,z_3)## to ##(1,0,\infty ).## Which one?9. Let ##G## be a connected open set and let ##f:G\longrightarrow \mathbb{C}## be an analytic function. Show that the following statements are equivalent:
- ##f\equiv 0##
- There is a point ##a\in G## such that ##f^{(n)}(a)=0## for each ##n\geq 0.##
- ##\{z\in G\,|\,f(z)=0\}## has a limit point in ##G.##
$$
|f(z)+g(z)|<|f(z)|+|g(z)|
$$
on ##\gamma ,## then
$$
Z_f-P_f=Z_g-P_g\;.
$$
High Schoolers only
11. (solved by @Not anonymous ) A gardener holds a water hose horizontally and wants to water a bed ##6\,\rm m## away. The water exits the hose at a speed of ##8\,\rm m/s\,.## Calculate the minimum height the gardener needs to hold the hose for the water to reach the bed, the speed at which the water droplets hit the bed, and the angle at which the water droplets hit the bed.12. (solved by @Not anonymous ) A faucet delivers a volume flow of ##V'=6\,\dfrac{\it l}{\rm min} ##. The connected garden hose has an inner diameter of ##d_1=18\,\rm mm ##, the nozzle a cross-section of ##d_2=5\,\rm mm.## Calculate the mass flow in the garden hose, the speed of the water in the garden hose, and the speed of the water at the nozzle. It is observed that the water jet widens after the nozzle. Why?13. (solved by @Not anonymous and @kshitij ) As a result of the refraction, the light bundle emanating from the slit ##S## produces two bundles that overlap in the screen area of width ##D## and appear to arise from two virtual slit images ##A## and ##B##. Since the two virtual slit images originate from the same slit, the light emanating from them is coherent and can interfere in the area of overlap.
Assume that the main cause of the widening is the rotation of the stars around the center of the galaxy. Assume further that the different wavelength is only due to the radial motion of the galaxy compared to our solar system.
What is the maximal rotational velocity of the observed stars, and what is the maximal velocity the galaxy is moving, and in which direction as seen from our solar system?
15. (solved by @Not anonymous ) The spiral galaxy ##\rm M81## near Ursa Major can already be viewed by a small telescope. It has an apparent magnitude of ##M=6.9.## The angle to the celestial pole is about ##21°.## Is it possible to observe ##\rm M81## the entire year, if you live in Toronto?
The following diagram shows data-points of light from the cepheid ##\rm C27## in ##\rm M81.##
Calculate our distance from ##\rm M81## in lightyears. (Use an average value of magnitude ##22.3## at a pulsation rate of ##30## per day and the relation ##M=-1.67-2.54\cdot\log_{10} p##.)
The second diagram is a comparison between ##\rm M81## and Milky Way. It shows the radial orbit velocity ##v## of the stars in relation to their distance ##r## from the galaxy center. Optical wavelengths are hardly to observe from around ##16\,kpc## on, so radio wavelengths are used.
Verify that if a celestial body orbits a center of great mass, then we can calculate the central mass approximately by ##M=\dfrac{v^2\cdot r}{G}.## Show by choosing two data-points that the rotation curve of ##\rm M81## is approximately ##v\sim \dfrac{1}{\sqrt{r}}## for ##r=10\,\rm{kpc}.## What does that mean for the mass distribution in ##\rm M81\,##? Estimate the mass of ##\rm M81## within the optical spectrum in units of sun masses.
The rotation curves of ##\rm M81## and the Milky Way differ a lot for great distances from the center. What does that mean for the mass distribution in our Milky Way?
The wavelength of the ##\alpha ##-line of hydrogen from the optical center of ##\rm M81## is measured to be ##\lambda_1=656.38\,\rm nm## in comparison to ##\lambda_0 =656.47 \,\rm nm.## Can we apply Hubble's law to ##\rm M81##?#
Last edited:
 . I have read about dark matter and dark energy in science articles and the enigma surrounding them only gets deeper every time!
. I have read about dark matter and dark energy in science articles and the enigma surrounding them only gets deeper every time!