Math Challenge - December 2019

[fresh_42]

Questions

1. Let $(X,d)$ be a metric space. The open ball with center $z\in X$ of radius $r > 0$ is defined as
$$
B_r(z) :=\{\,x\in X\,|\,d(x,z)<r\,\}
$$
a.) Give an example for
$$
\overline{B_r(z)} \neq K_r(z) :=\{\,x\in X\,|\,d(x,z)\leq r\,\}
$$
Does at least one of the inclusions $\subseteq$ or $\supseteq$ always hold?

b.) What are the answers in the previous case, if we additionally assume that $(X,d)$ has an inner metric?

An inner metric $d_0$ associated to $d$ is defined as the infimum of all lengths of rectified curves between two points:
Let $\sigma \, : \,[0,1]\longrightarrow X$ with $\sigma(0)=x\, , \,\sigma(1)=y$ a rectified curve with length
$$
L(\sigma)=\sup \left\{ \left. \sum_{k=1}^n d(\sigma(t_{k-1}),\sigma(t_k) )\,\right|\,0=t_0<t_1<\cdots < t_n=1\, , \,n\in \mathbb{N} \right\}
$$
Then $d_0(x,y)=\inf L(\sigma)\,.$

2. Let $f(z)=\dfrac{7z-51}{z^2-12z+27}$ be a complex function.
a.) Determine the Laurent series of $f(z)$ and their radius of convergences around $z=3$ in the cases where $0$ is in the area of convergence, and $10$ is in the area of convergence.
b.) Determine $\lim_{z \to 3}f(z)\, , \,\operatorname{Res}(f,3)$ and the kind of singularity in $z=3\,.$

3. Write the following groups as amalgamated products of cyclic groups:
a.) $G=\langle x,y\,|\, x^3y^{-3},y^6 \rangle$
b.) $H=\langle x,y\,|\, x^{30}, y^{70},x^3y^{-5} \rangle$

4. Prove that there are uncountably many groups, which are generated by two elements, and not finitely presented.
Hint: There are uncountably many non-isomorphic groups with two generators [Bernhard Neumann, 1937].

5. (solved by @archaic ) Let $f : [1,\infty) \longrightarrow [0,\infty)$ be a continuously differentiable function. Write $S$ for the solid of revolution of the graph $y = f(x)$ about the $x-$axis. If the surface area of $S$ is finite, then so is the volume.

6. Calculate $\sum_{k,j=1}^\infty \dfrac{1}{kj(k+j)^2}$

7. (solved by @Fred Wright ) Calculate $S:= \displaystyle{\sum_{n=0}^\infty}\,\displaystyle{\sum_{k=0}^n}\,\dfrac{3^k(2n-2k)!(2k)!}{2^k8^n[(n-k)!]^2[k!]^2(2n(1+2k)+(1-4k^2))}$

8. (solved by @Mastermind01 ) Solve $y'x-y=\sqrt{x^2-y^2}$

9. Let $(a_n)_{n\in \mathbb{N}}\, , \,(b_n)_{n\in \mathbb{N}} \subseteq \mathbb{R}_{\geq 0}$ be two sequences of nonnegative numbers, where not all sequence elements vanish, and be $p,q\in \mathbb{R}$ with $1<p,q<\infty\, , \,\frac{1}{p}+\frac{1}{q}=1\,.$ Prove
$$
\sum_{n=1}^\infty \sum_{m=1}^\infty \dfrac{a_nb_m}{n+m} < \dfrac{\pi}{\sin (\pi/p)} \cdot \left(\sum_{n=1}^\infty a_n^p\right)^{\frac{1}{p}} \cdot \left(\sum_{m=1}^\infty b_m^q\right)^{\frac{1}{q}}
$$

10. Let $f\, : \,\mathbb{R}_{\geq 0} \longrightarrow \mathbb{R}_{\geq 0}$ be an integrable function and $p>1\,.$ Prove
$$
\int_0^\infty\left(\dfrac{1}{x}\int_0^x f(t)\,dt\right)^p\,dx \leq \left(\dfrac{p}{p-1}\right)^p \int_0^\infty (f(x))^p\,dx
$$
Hint: Substitute $t=xu^{p/r}$ and at the end $r=p-1\,.$

High Schoolers only

11. (solved by @Not anonymous ) Choose any odd prime, square it and subtract one. Show that the result is always divisible by twenty-four except for three.
What can be said, if we take the prime up to the power four, and subtract one?

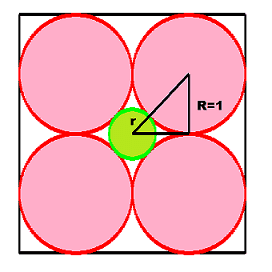
12. (solved by @Not anonymous ) In a square of side length $4$, there is a circle of radius $1$ in each corner. In the center of the square is another circle that touches the other four. Analogously, in the three-dimensional case, in the center of a cube of edge length $4$, there would be a sphere which would touch eight spheres of radius $1$ placed in the corners of the cube. In which dimension does the central hypersphere become so large that it touches all sides of the hypercube?

13. (solved by @Not anonymous ) There is only one rule at Christmas at the world's richest family: The gifts have to be expensive, heavy and glamorous. So they all present statues of pure gold. It may be large figure, a tiger sculpture or an opulent candlestick. The eldest son who doesn't live at home anymore receives gifts of nine tons total, but none of which is heavier than a ton. He wants to bring home all of them, but only could rent trucks which can load three tons maximal. How many trucks are needed to at least be able to transport all gifts of gold at the same time?

14. (solved by @Not anonymous ) Prove $\dfrac{a}{b+c}+\dfrac{b}{c+a}+\dfrac{c}{a+b}\geq \dfrac{3}{2}$ for $a,b,c >0$

15. (solved by @Not anonymous ) Let $\mathbf{x}=(x_1,\ldots ,x_n)\, , \,\mathbf{y}=(y_1,\ldots ,y_n)$ be tuples of positive numbers. Prove
$$
\prod_{k=1}^{n} (x_k+y_k)^{1/n} \geq \prod_{k=1}^{n} x_k^{1/n} + \prod_{k=1}^{n} y_k^{1/n}
$$

 

[Mastermind01]

8. Solve $y′x−y=\sqrt{x^2−y^2}$

Spoiler

Dividing by x throughout we get $$y' - \frac{y}{x} = \frac{\sqrt{x^2 - y^2}}{x} \implies y' - \frac{y}{x} = \sqrt{\frac{x^2 - y^2}{x^2}}$$.

Now using the substitution $y = vx$ and differentiating it throughout we get $y' = xv'+ v$

Using the above relations and substituting them for $y$ and $y'$ respectively, we get $xv' + v - v = \sqrt{1-v^2} \implies xv' =\sqrt{1-v^2}$
Now we separate the variables i.e. $x\frac{dv}{dx} = \sqrt{1-v^2} \implies \frac{dv}{\sqrt{1-v^2}} = \frac{dx}{x}$ and integrate $\int \frac{dv}{\sqrt{1-v^2}} = \int \frac{dx}{x}$. The integral on the R.H.S is simply $\ln{|x|}$ for the L.H.S we use the subsitution $v = sin z$ to get $\int \frac{\cos{z} dz}{cos z} \implies z$ Therefore the L.H.S integral is $\arcsin{v}$ (by substituting z for v). Using the integral values in the original equation we get $v = \sin(\ln{|x|} + C)$. Replacing v, the final answer is $y = x\sin(\ln{|x|} + C)$

 

[fresh_42]

8. Solve $y′x−y=\sqrt{x^2−y^2}$

Spoiler

Dividing by x throughout, $y' - \frac{y}{x} = \sqrt{\frac{x^2 + y^2}{x^2}}$. Now using the subsitution $y = vx$ and $y' = xv'+ v$ we get $xv' + v - v = \sqrt{1-v^2}$ Seperating and integrating we get $v = \sin(\ln{x} + C)$. Replacing v, the final answer is $y = x\sin(\ln{x} + C)$

Can you write down the calculation for those less familiar with differential equations? And I assume you meant $\ln|x|$! Nevertheless, it's only part of the answer.

 

[Mastermind01]

Can you write down the calculation for those less familiar with differential equations? And I assume you meant $\ln|x|$! Nevertheless, it's only part of the answer.

I amended my post to explain things better and yes I missed the || on both lines

 

[fresh_42]

I amended my post to explain things better and yes I missed the || on both lines

Still not the complete answer.

 

[Mastermind01]

Still not the complete answer.

What am I missing?

 

[fresh_42]

What am I missing?

You cannot rule out that $v(x_0)^2=1$ for some point $x_0$. This means we have $\sqrt{1-v(x_0)^2}=0$ or $v(x_0)=\pm 1$, i.e. $y=\pm x$ are also solutions to the Jacobian Differential Equation $y'x-y=\sqrt{x^2-y^2}.$

 

[Mastermind01]

You cannot rule out that $v(x_0)^2=1$ for some point $x_0$. This means we have $\sqrt{1-v(x_0)^2}=0$ or $v(x_0)=\pm 1$, i.e. $y=\pm x$ are also solutions to the Jacobian Differential Equation $y'x-y=\sqrt{x^2-y^2}.$

Did not realize I was doing that. I do now. Thank you.

 

[Fred Wright]

6. Calculate $\sum_{k,j=1}^{\infty}\frac{1}{kj(k+j)^2}$

Spoiler

$$\sum_{k,j=1}^{\infty}\frac{1}{kj(k+j)^2}= \frac {1}{2^2} + \frac {1}{3^2 6^2} + \frac{1}{4^2 8^2} + \frac{1}{5^2 10^2} + ...
\\ = \frac{1}{2^2} +\frac{1}{2^2 2^4} + \frac{1}{2^2 3^4} + \frac{1}{2^2 4^4} + \frac{1}{2^2 5^4} + ...
\\ = \frac{1}{4}\sum_{n=1}^{\infty}\frac{1}{n^4}$$
I observe that a piecewise continuous function f can be expressed as a Fourier series,$$f(x)=\frac{a_0}{2}+\sum_{n=1}^{\infty}a_ncos(\frac{n\pi x}{L}) + b_nsin(\frac{n\pi x}{L})
\\a_n=\frac{1}{L}\int_{-L}^{L}f(x)cos(\frac{n\pi x}{L})dx
\\b_n=\frac{1}{L}\int_{-L}^{L}f(x)sin(\frac{n\pi x}{L})dx$$ I also recall Parseval's identity,$$\frac{1}{\pi}\int_{-\pi}^{\pi}| f(x) |^2dx=\frac{a_{0}^2}{2}+\sum_{n=1}^{\infty} (a_{n}^2 + b_{n}^2)$$I assert without proof that for $L=\pi$ the Fourier series for $x^2$ is$$ x^2= \frac{\pi^2}{3} + 4(cos(x) -\frac{cos(2x)}{2^2} + \frac{cos(3x)}{3^2}-...
\\a_0=\frac{2\pi^2}{3}
\\a_n= \frac{4(-1)^{n}}{n^2}$$From Parseval's identity,$$\frac{1}{\pi}\int_{-\pi}^{\pi}x^4dx=\frac{(\frac{2\pi^2}{3})^2}{2} + \sum_{n=1}^{\infty}\frac{16}{n^4}
\\ \frac{2\pi^4}{5}=\frac{(\frac{2\pi^2}{3})^2}{2} + \sum_{n=1}^{\infty}\frac{16}{n^4}
\\ \sum_{n=1}^{\infty}\frac{1}{n^4} = \frac{\pi^4}{90}
\\\sum_{k,j=1}^{\infty}\frac{1}{kj(k+j)^2}=\frac{\pi^4}{360}$$

 

[fresh_42]

I cannot follow you. In your very first equation I get different terms:
$$
\sum_{k,j=^1}^\infty \dfrac{1}{kj(k+j)^2}=\underbrace{\dfrac{1}{1\cdot 2^2}}_{(1,1)}+\underbrace{\dfrac{2}{2\cdot 3^2}}_{(1,2)}+\underbrace{\dfrac{1}{4\cdot 4^2}}_{(2,2)}+\underbrace{\dfrac{2}{3\cdot 4^2}}_{(1,3)}+\underbrace{\dfrac{2}{6\cdot 5^2}}_{(2,3)}+\underbrace{\dfrac{1}{9\cdot 6^2}}_{(3,3)}+\ldots
$$
so you must have used a summation I cannot see. And the result is a different one.

The trick is indeed to rewrite the summands. My proof uses integrals.

 

[Not anonymous]

11. Choose any odd prime, square it and subtract one. Show that the result is always divisible by twenty-four except for three.
What can be said, if we take the prime up to the power four, and subtract one?

Spoiler: Question 11

Any odd prime $p$ other than 3 can we written as $p = (2k + 3)$ where $k$ is a positive integer and also meets the criterion $k \neq 0\ mod\ 3$.

Let $A$ denote $p^2 - 1$. $A = p^2 - 1 = (p - 1)(p + 1) = (2k + 2)(2k + 4) = 4(k + 1)(k + 2)$. We now show that $(k + 1)(k + 2)$ must be a multiple of 6. $(k + 1)(k + 2)$ must be an even number since it is a multiple of 2 consecutive integers. If $(k + 1)$ is odd then $(k + 2)$ must be even and if $(k + 1)$ is even then $(k + 1)$ must be odd. Therefore $(k + 1)(k + 2) \equiv 0\ mod\ 2$, i.e. it is a multiple of 2.

Secondly, we show that $(k + 1)(k + 2)$ must be a multiple of 3. $k \neq 0\ mod\ 3$ (as stated earlier) $\Rightarrow k \equiv 1\ mod\ 3$ or $k \equiv 2\ mod\ 3 \Rightarrow (k + 1)(k + 2) \equiv (2\ mod\ 3)(3\ mod\ 3) = 0\ mod\ 3$ or $(3\ mod\ 3)(4\ mod\ 3) = 0\ mod\ 3$.

Combining the above 2 observations, it follows that $(k + 1)(k + 2) = 0\ mod\ 6$, i.e. $(k + 1)(k + 2)$ is a multiple of 6. Therefore, $4(k + 1)(k + 2)$ must be a multiple of $4 \times 6 = 24$. Hence proved.

For the second part of the question, we need to consider $B = p^4 - 1 = (p^2 - 1)(p^2 + 1)$. It has already been proved that $(p^2 - 1)$ is a multiple of 24. Now, since $p$ is an odd number, $(p^2 + 1)$ must be ab even number, i.e. a multiple of 2. Therefore $p^4 - 1$ must be a multiple of $24 \times 2 = 48$

 

[fresh_42]

Spoiler: Question 11

Any odd prime $p$ other than 3 can we written as $p = (2k + 3)$ where $k$ is a positive integer and also meets the criterion $k \neq 0\ mod\ 3$.

Let $A$ denote $p^2 - 1$. $A = p^2 - 1 = (p - 1)(p + 1) = (2k + 2)(2k + 4) = 4(k + 1)(k + 2)$. We now show that $(k + 1)(k + 2)$ must be a multiple of 6. $(k + 1)(k + 2)$ must be an even number since it is a multiple of 2 consecutive integers. If $(k + 1)$ is odd then $(k + 2)$ must be even and if $(k + 1)$ is even then $(k + 1)$ must be odd. Therefore $(k + 1)(k + 2) \equiv 0\ mod\ 2$, i.e. it is a multiple of 2.

Secondly, we show that $(k + 1)(k + 2)$ must be a multiple of 3. $k \neq 0\ mod\ 3$ (as stated earlier) $\Rightarrow k \equiv 1\ mod\ 3$ or $k \equiv 2\ mod\ 3 \Rightarrow (k + 1)(k + 2) \equiv (2\ mod\ 3)(3\ mod\ 3) = 0\ mod\ 3$ or $(3\ mod\ 3)(4\ mod\ 3) = 0\ mod\ 3$.

Combining the above 2 observations, it follows that $(k + 1)(k + 2) = 0\ mod\ 6$, i.e. $(k + 1)(k + 2)$ is a multiple of 6. Therefore, $4(k + 1)(k + 2)$ must be a multiple of $4 \times 6 = 24$. Hence proved.

For the second part of the question, we need to consider $B = p^4 - 1 = (p^2 - 1)(p^2 + 1)$. It has already been proved that $(p^2 - 1)$ is a multiple of 24. Now, since $p$ is an odd number, $(p^2 + 1)$ must be ab even number, i.e. a multiple of 2. Therefore $p^4 - 1$ must be a multiple of $24 \times 2 = 48$

Yes, correct.

But $48\,|\,p^4-1$ can be made a bit stronger. Can someone show that in fact $240\,|\,p^4-1$ for $p>5$?

 

[archaic]

5. Let $f : [1,\infty) \longrightarrow [0,\infty)$ be a continuously differentiable function. Write $S$ for the solid of revolution of the graph $y=f(x)$ about the $x$-axis. If the surface area of $S$ is finite, then so is the volume.

Spoiler: Question 5

The solid of revolution about the $x$-axis:
$$S=\pi\int_1^\infty f^2(x)dx$$
The surface area $S$:
$$A=2\pi\int_1^\infty f(x)\sqrt{1+(f'(x))^2}dx$$
For $A$ to converge, $\lim_{x\to\infty} f(x)$ needs to be $0$ (if the limit is finite but non-zero, the limit of $f'(x)$ would be $0$, but the limit of $f(x)\sqrt{1+(f'(x))^2}$ would have the limit of $f(x)$ and the integral will diverge). It follows that the limit of $f'(x)=0$ as $x\to\infty$ (since $f(x)$ is becoming constant).
We then have:
$$f(x)\sqrt{1+(f'(x))^2}dx\underset{\infty}{\sim}f(x)dx\Rightarrow \int_0^\infty f(x)dx \text{ converges as A converges.}$$
Since $f(x)$ is going to $0$, then at some point $f^2(x)\leq f(x)$, thus $\int_1^\infty f^2(x)dx \leq \int_1^\infty f(x)dx$ from which follows that if $A$ converges, so does $S$.

 

[fresh_42]

Spoiler: Question 5

The solid of revolution about the $x$-axis:
$$S=\pi\int_1^\infty f^2(x)dx$$
The surface area $S$:
$$A=2\pi\int_1^\infty f(x)\sqrt{1+(f'(x))^2}dx$$
For $A$ to converge, $\lim_{x\to\infty} f(x)$ needs to be $0$ (if the limit is finite but non-zero, the limit of $f'(x)$ would be $0$, but the limit of $f(x)\sqrt{1+(f'(x))^2}$ would have the limit of $f(x)$ and the integral will diverge). It follows that the limit of $f'(x)=0$ as $x\to\infty$ (since $f(x)$ is becoming constant).
We then have:
$$f(x)\sqrt{1+(f'(x))^2}dx\underset{\infty}{\sim}f(x)dx\Rightarrow \int_0^\infty f(x)dx \text{ converges as A converges.}$$
Since $f(x)$ is going to $0$, then at some point $f^2(x)\leq f(x)$, thus $\int_1^\infty f^2(x)dx \leq \int_1^\infty f(x)dx$ from which follows that if $A$ converges, so does $S$.

Yes, correct. The object is known as Gabriel's Horn, where $f(x)=1/x$.

A bit more formalized:
\begin{align*}
\lim_{t \to \infty} \sup_{x \geq t}f(x)^2-f(1) &= \limsup_{t \to \infty} \int_1^t \left( f(x)^2 \right)'\,dx\\
&\leq \int_1^\infty \left| \left( f(x)^2 \right)' \,\right|\,dx\\
&=2 \int_1^\infty f(x)|f'(x)|\,dx\\
&\leq 2 \int_1^\infty f(x)\sqrt{1+f'(x)^2}\,dx\\
&=\dfrac{A}{\pi} < \infty
\end{align*}
Hence there is a $t_0\geq 1$ such that $\sup_{x\geq t_0}f(x) < \infty$ and so is $L:=\sup_{x\geq 1}f(x) < \infty$ because $f(x)$ is continuous with values in $[0,\infty)$, i.e. bounded on $[1,\infty)$. For the volume we have
\begin{align*}
V&=\int_1^\infty f(x)\cdot \pi f(x)\, dx \\ &\leq \int_1^\infty \dfrac{L}{2}\cdot 2\pi f(x)\,dx \\ &\leq \dfrac{L}{2}\int_1^\infty 2\pi f(x) \sqrt{1+f'(x)^2}\,dx \\ &= \dfrac{L}{2}\cdot A \\ &< \infty
\end{align*}

 

[archaic]

The trick is indeed to rewrite the summands. My proof uses integrals.

Why do you need integrals? Is it wrong to say that, since they take the same value each time, we can replace all $k$s and $j$s by $n$?
$$\sum_{k,\,j=1}^\infty\dfrac{1}{kj(k+j)^2}=\sum_{n=1}^\infty\dfrac{1}{nn(n+n)^2}$$

 

[fresh_42]

Why do you need integrals? Is it wrong to say that, since they take the same value each time, we can replace all $k$s and $j$s by $n$?
$$\sum_{k,\,j=1}^\infty\dfrac{1}{kj(k+j)^2}=\sum_{n=1}^\infty\dfrac{1}{nn(n+n)^2}$$

This equation you wrote is wrong (where has $m$ gone to?), the sum has to be done across both indices, so it's a Cauchy product. We sum up all combinations: $(1,1),(1,2),(2,1),(2,2),(1,3),(3,1),(2,3),(3,2),(3,3), \ldots$ I don't know whether we need integrals, my solution uses them to get rid of the $(k+j)$ terms.

 

[archaic]

This equation you wrote is wrong (where has $m$ gone to?), the sum has to be done across both indices, so it's a Cauchy product. We sum up all combinations: $(1,1),(1,2),(2,1),(2,2),(1,3),(3,1),(2,3),(3,2),(3,3), \ldots$ I don't know whether we need integrals, my solution uses them to get rid of the $(k+j)$ terms.

Which $m$?

But from what you say I guess you meant $\sum_{k=1}^\infty\sum_{j=1}^\infty \dfrac{1}{kj(k+j)^2}$?

 

[fresh_42]

(The $m$ in your formula on the RHS.)

Yes, $\sum_{k,j=1}^\infty$ is short for $\sum_{k=1}^\infty \sum_{j=1}^\infty$.

 

[archaic]

(The $m$ in your formula on the RHS.)

Yes, $\sum_{k,j=1}^\infty$ is short for $\sum_{k=1}^\infty \sum_{j=1}^\infty$.

I was sceptic at first but maybe you are on a mobile phone? It's $n*n$ :D

 

[archaic]

@Fred Wright considered the sum as I did, which simplifies to his expression.

 

[fresh_42]

I was sceptic at first but maybe you are on a mobile phone? It's $n*n$ :D

Oh, I see. No, that's wrong. The summation involves all mixed terms, too. The shortest notation is $\sum_{k,j}$ but I added from $1$ to $\infty$ (which refers to both).

 

[kent davidge]

13. 3 trucks? since three of the gifts weight no more than 3 tons

 

[fresh_42]

13. 3 trucks? since three of the gifts weight no more than 3 tons

No.

 

[Not anonymous]

12. In a square of side length 4, there is a circle of radius 1 in each corner. In the center of the square is another circle that touches the other four. Analogously, in the three-dimensional case, in the center of a cube of edge length 4, there would be a sphere which would touch eight spheres of radius 1 placed in the corners of the cube. In which dimension does the central hypersphere become so large that it touches all sides of the hypercube?

Spoiler: Question 12. But this could be a doubt rather than an answer

Is one-dimensional case to be considered valid for this question? In the 1-D case, instead of a square, we will have a line segment of length 4 on the X-axis (or the only axis in 1-D). And instead of the circles of the 2-D case, we will have 2 pairs of points, $(X=x_1, X=x_2)$ and $(X=x_2, X=x_3)$ such that $x_2 - x_1 = 2$ and $x_3 - x_2 = 2$. And the 1-D point $(X=x_2)$ in the 1-D case is analogous to the central circle/hypersphere of higher dimensional cases. And this point happens to lie on the line segment $(X=x_1)$-to-$(X=x_3)$ on the X-axis and this line segment is the 1-D equivalent of an edge of square/hypercube in higher dimensions, thus meeting the criteria of central hypersphere touching all sides of the hypercube but admittedly it does not really meet the condition of being "large".

 

[fresh_42]

Spoiler: Question 12. But this could be a doubt rather than an answer

Is one-dimensional case to be considered valid for this question? In the 1-D case, instead of a square, we will have a line segment of length 4 on the X-axis (or the only axis in 1-D). And instead of the circles of the 2-D case, we will have 2 pairs of points, $(X=x_1, X=x_2)$ and $(X=x_2, X=x_3)$ such that $x_2 - x_1 = 2$ and $x_3 - x_2 = 2$. And the 1-D point $(X=x_2)$ in the 1-D case is analogous to the central circle/hypersphere of higher dimensional cases. And this point happens to lie on the line segment $(X=x_1)$-to-$(X=x_3)$ on the X-axis and this line segment is the 1-D equivalent of an edge of square/hypercube in higher dimensions, thus meeting the criteria of central hypersphere touching all sides of the hypercube but admittedly it does not really meet the condition of being "large".

No, the one dimensional case is a) not meant and b) different from what you wrote.

The one dimensional "square" of side length $4$ is $[0,4]$. The "circles" in the corners of radius $1$ would be $[0,2]$ and $[2,4]$, which leaves the point $\{\,2\,\}$ as the central "circle", which does not touch the boundary $\{\,0,4\,\}$ of the "cube" $[0,4]$ at all.

The trick is to calculate the radius of the inner hyperspheres. This radius is $0$ in one dimension.

 

[Not anonymous]

13. There is only one rule at Christmas at the world's richest family: The gifts have to be expensive, heavy and glamorous. So they all present statues of pure gold. It may be large figure, a tiger sculpture or an opulent candlestick. The eldest son who doesn't live at home anymore receives gifts of nine tons total, but none of which is heavier than a ton. He wants to bring home all of them, but only could rent trucks which can load three tons maximal. How many trucks are needed to at least be able to transport all gifts of gold at the same time?

Shouldn't the question be asking what is the maximum but optimal number of trucks needed rather than the minimum? The minimum must be 3 because with fewer then 3 trucks, it is not possible to transport 9 tons in one go since then the trucks will be required to carry more than 3 tons which is beyond their limit. For example, if there were a total 9 gifts each weighing 1 ton, then 3 trucks would be necessary but also sufficient as each of the 3 trucks can be loaded with 3 items each, so each truck carries 3 tons.

Spoiler: Question 13 (with the above interpretation of the question, i.e. for maximum number of trucks)

Answer: 4 trucks are always sufficient to transport all items at the same time, whatever be the number of gift items and their individual weights provided no one of them exceeds 1 ton in weight and they add up to 9 tons.

First, we show that 3 trucks are not always sufficient using a counterexample. If the gifts consisted of 4 items weighing 0.975 tons each, 3 items weighing 0.9 tons each and 3 items weighing 0.8 tons each, then we have 10 items with a total weight of 9 tons. In this case, no single truck can carry more than 3 items in one go because the minimum weight per item is 0.8 tons and loading 4 items would mean exceeding the 3 ton limit. So the 3 trucks together cannot carry more than 9 items at the same time. Since the total number of items is 10, it is proven that 3 trucks are insufficient.

To show that 4 trucks is always a sufficient number, we show a strategy that is guaranteed to accommodate all items in 4 trucks. Let the total number of items be $n$. Sort the items in the descending order of their weights and let the weights of the items in the sorted order be $w_1, w_2, ..., w_n$. Start loading the items in that order to the 1st truck, move to truck 2 if the next item (say item $i$, weight $w_i$) to be loaded cannot be taken by truck 1 as it would lead to 3 ton limit being exceeded (i.e. unused capacity of truck 1 $\leq w_i$). Continue with the same process till we reach an item which cannot be loaded to truck 2 because its weight $w_j$ exceeds the unused capacity of truck 2. Now start loading remaining items to truck 3 till no more items can be accommodated in that truck. At this point, let $f_1, f_2, f_3$ be the unused/free capacity in the 3 trucks. It must be the case that $f_j \lt 1\ \forall i \in {1, 2, 3}$ since if any $f_j \ge 1$ and yet there are remaining items that could not be accommodated in 3 trucks, then the trucks must have not have been loaded as per the above strategy. Had the strategy been followed, then we would have stopped loading to truck $j$ only if the next item's weight exceeded the unused capacity of the truck and since all items are of weight not exceeding 1 ton whereas $f_j \ge 1$, at least 1 pending item could have been loaded to truck $j$ and so the free capacity must have becomes less than 1.

It follows that the final total unused capacity of the 3 trucks with the above loading strategy, $f_{total} = f_1 + f_2 + f_3 \lt 3$. The total used capacity of the 3 trucks would therefore be $u_{total} = (3 - f_{1}) + (3 - f_{2}) + (3 - f_{3}) = 9 - f_{total} \gt 6$. Since the total weight of all gifts if 9 tons, and more than 6 tons could be accommodated in 3 trucks as per the above equations, it follows that the total weight of the remaining "unaccommodated" items must be less than 3 tons. And all these remaining items can be loaded in a 4th truck of carrying capacity 3 tons.

 

[Not anonymous]

No, the one dimensional case is a) not meant and b) different from what you wrote.

The one dimensional "square" of side length $4$ is $[0,4]$. The "circles" in the corners of radius $1$ would be $[0,2]$ and $[2,4]$, which leaves the point $\{\,2\,\}$ as the central "circle", which does not touch the boundary $\{\,0,4\,\}$ of the "cube" $[0,4]$ at all.

The trick is to calculate the radius of the inner hyperspheres. This radius is $0$ in one dimension.

Thanks for reviewing that attempted solution and clarifying. I am glad that I had at least got the concepts of 1-D "circles" and 1-D "central circle" correct in that attempt. My understanding of the central circle touching the boundary of the "square"/"cube" was that it had to touch one point on each "edge"/"face" of the "square"/"cube". As per that interpretation, the 1-D "central circle", i.e. the mid-point in my solution, does touch the boundary in the 1-D case. By boundaries, does the question mean the corner points?

"The trick is to calculate the radius of the inner hyperspheres." - I guessed the same initially but wanted to validate the 1-D case 1st

 

[fresh_42]

Shouldn't the question be asking what is the maximum but optimal number of trucks needed rather than the minimum? The minimum must be 3 because with fewer then 3 trucks, it is not possible to transport 9 tons in one go since then the trucks will be required to carry more than 3 tons which is beyond their limit. For example, if there were a total 9 gifts each weighing 1 ton, then 3 trucks would be necessary but also sufficient as each of the 3 trucks can be loaded with 3 items each, so each truck carries 3 tons.

This is hair splitting. Every number of insufficiently many trucks is a minimum as every number larger than necessarily many trucks is a maximum. The exact wording would be: The minimal number of necessary trucks, the least upper bound, the supremum of all numbers of sufficiently many trucks. The supremum is a minimum, as it is the least upper bound, and it is a maximum, as it is an upper bound of necessary trucks. Hence the new word. The greatest lower bound would called infimum. So we could likewise say, that we are looking for the infimum of all numbers of insufficiently many trucks plus one.

Answer: 4 trucks are always sufficient to transport all items at the same time, whatever be the number of gift items and their individual weights provided no one of them exceeds 1 ton in weight and they add up to 9 tons.

First, we show that 3 trucks are not always sufficient using a counterexample. If the gifts consisted of 4 items weighing 0.975 tons each, 3 items weighing 0.9 tons each and 3 items weighing 0.8 tons each, then we have 10 items with a total weight of 9 tons. In this case, no single truck can carry more than 3 items in one go because the minimum weight per item is 0.8 tons and loading 4 items would mean exceeding the 3 ton limit. So the 3 trucks together cannot carry more than 9 items at the same time. Since the total number of items is 10, it is proven that 3 trucks are insufficient.

Right. Easier would have been: $10$ gifts $900\,kg$ each.

To show that 4 trucks is always a sufficient number, we show a strategy that is guaranteed to accommodate all items in 4 trucks. Let the total number of items be $n$. Sort the items in the descending order of their weights and let the weights of the items in the sorted order be $w_1, w_2, ..., w_n$. Start loading the items in that order to the 1st truck, move to truck 2 if the next item (say item $i$, weight $w_i$) to be loaded cannot be taken by truck 1 as it would lead to 3 ton limit being exceeded (i.e. unused capacity of truck 1 $\leq w_i$). Continue with the same process till we reach an item which cannot be loaded to truck 2 because its weight $w_j$ exceeds the unused capacity of truck 2. Now start loading remaining items to truck 3 till no more items can be accommodated in that truck. At this point, let $f_1, f_2, f_3$ be the unused/free capacity in the 3 trucks. It must be the case that $f_j \lt 1\ \forall i \in {1, 2, 3}$ since if any $f_j \ge 1$ and yet there are remaining items that could not be accommodated in 3 trucks, then the trucks must have not have been loaded as per the above strategy. Had the strategy been followed, then we would have stopped loading to truck $j$ only if the next item's weight exceeded the unused capacity of the truck and since all items are of weight not exceeding 1 ton whereas $f_j \ge 1$, at least 1 pending item could have been loaded to truck $j$ and so the free capacity must have becomes less than 1.

It follows that the final total unused capacity of the 3 trucks with the above loading strategy, $f_{total} = f_1 + f_2 + f_3 \lt 3$. The total used capacity of the 3 trucks would therefore be $u_{total} = (3 - f_{1}) + (3 - f_{2}) + (3 - f_{3}) = 9 - f_{total} \gt 6$. Since the total weight of all gifts if 9 tons, and more than 6 tons could be accommodated in 3 trucks as per the above equations, it follows that the total weight of the remaining "unaccommodated" items must be less than 3 tons. And all these remaining items can be loaded in a 4th truck of carrying capacity 3 tons.

Well done, albeit it bit complicated. You should study math! Mathematicians tend to either a totally trivial solution or a complicated one!

So I invite everyone to find an easier argument, why 4 trucks are sufficient!

 

[Fred Wright]

Problem 7. I don't know how to express, in closed form, the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )+ (1-4k^2))}$$ However, for anyone that may be interested, the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}$$can be expressed in closed form as follows. I note that the double sum can be expressed as ,$$\sum_{n=0}^{\infty}\sum_{k=0}^na_{k,n-k}=\sum_{m=0}^{\infty}\sum_{k=0}^{\infty} a_{k,m}
\\ m=n-k$$where $a_{k,m}$ are terms in the expansion. On making the substitution $m=n-k$ in the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}
\\ =\sum_{m=0}^{\infty}\sum_{k=0}^{\infty} (\frac{3}{2})^{k} \frac{2(m)!(2k)!}{8^{m+k} \left [(m)!\right ]^2 \left [(k)!\right ]^2 \left (2(m+k)(1+2k \right )- (1+4k^2))}
\\ =\sum_{m=0}^{\infty} \sum_{k=0}^{\infty} (\frac{1}{2})^{m} (\frac{3}{4})^{k} \frac{(2m)!(2k)!}{4^m 4^k\left [(m)!\right ]^2 \left [(k)!\right ]^2 (2m+1)(2k+1)}
\\ =2\sqrt{\frac{2}{3}}\sum_{m=0}^{\infty} \sum_{k=0}^{\infty} (\frac{1}{\sqrt{2}})^{2m+1} (\frac{\sqrt{3}}{2})^{2k+1} \frac{(2m)!(2k)!}{4^m 4^k\left [(m)!\right ]^2 \left [(k)!\right ]^2 (2m+1)(2k+1)}
\\=2\sqrt{\frac{2}{3}} \sum_{m=0}^{\infty}(\frac{1}{\sqrt{2}})^{2m+1}\frac{(2m)!}{4^m [(m)! ]^2 (2m+1)} \sum_{k=0}^{\infty}(\frac{\sqrt{3}}{2} )^{2k+1}\frac{(2k)!}{4^k[(k)! ]^2 (2k+1)} $$I expand the function$\frac{1} {\sqrt{1-x^2}}$ in a binomial series,$$\frac{1} {\sqrt{1-x^{2}}}=\sum_{n=0}^{\infty}\begin{pmatrix}
\frac{-1}{2} \\
n
\end{pmatrix} x^{2n} =\sum_{n=0}^{\infty}\frac{(2n)!}{4^n(n!)^2}x^{2n}$$and I recall that,$$\sin^{-1}(x)=\int_0^x \frac{dt}{\sqrt{1-t^2}}
\\=\sum_{n=0}^{\infty} \int_0^x\frac{(2n)!}{4^n(n!)^2}x^{2n}dx
\\=\sum_{n=0}^{\infty} \frac{(2n)!}{4^n(n!)^2(2n+1)}x^{2n +1}$$On comparison with the double sum I deduce,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}
\\=2\sqrt{\frac{2}{3}}\sin^{-1} (\frac{1}{\sqrt{2}})\sin^{-1} (\frac{\sqrt{3}}{2})$$

 

[fresh_42]

Thanks for reviewing that attempted solution and clarifying. I am glad that I had at least got the concepts of 1-D "circles" and 1-D "central circle" correct in that attempt. My understanding of the central circle touching the boundary of the "square"/"cube" was that it had to touch one point on each "edge"/"face" of the "square"/"cube".

Yes. The symmetry of the problem however, guarantees that one point on one edge and one point on every edge (which are not vertices, hence necessarily pairwise different) are equivalent.

The central circle is defined by

In the center of the square is another circle that touches the other four.

The other four what? Since circle is the only object available in this sentence, it is allowed to refer to it without repetition. Such a sentence has always to be read as "In the center of the square is another circle that touches the other four [circles]." A specification is only necessary in case more than one object is available as a possible reference. But there are only four circles of that kind. The other object which has four somethings are the edges, which are a) no circles and b) not been mentioned in the other part of the sentence, and can thus be ruled out.

However, we don't want to study grammar here - the more as English isn't my native language. So in our context it is sufficient to simply ask!

As per that interpretation, the 1-D "central circle", i.e. the mid-point in my solution, does touch the boundary in the 1-D case. By boundaries, does the question mean the corner points?

No, and no. $\{\,2\,\}$ touches the boundaries of $[0,2]$ and $[2,4]$, hence it is the central circle. But it does not touch the boundary (edges, hyperfaces) of the square (hypercube) which are $\{\,0\,\}$ and $\{\,4\,\}$ in this trivial one dimensional case.

"The trick is to calculate the radius of the inner hyperspheres." - I guessed the same initially but wanted to validate the 1-D case 1st

This is not really a case because all objects become intervals and boundaries single points.

Do not force me to define the entire thing mathematically correct in $\mathbb{R}^n$. It is sill a high school question which can be solved with high school methods. A mathematical rigorous description would exceed the length of the argument to prove the statement! At least if I had to use high school language.

 

[fresh_42]

Problem 7. I don't know how to express, in closed form, the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )+ (1-4k^2))}$$ However, for anyone that may be interested, the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}$$can be expressed in closed form as follows. I note that the double sum can be expressed as ,$$\sum_{n=0}^{\infty}\sum_{k=0}^na_{k,n-k}=\sum_{m=0}^{\infty}\sum_{k=0}^{\infty} a_{k,m}
\\ m=n-k$$where $a_{k,m}$ are terms in the expansion. On making the substitution $m=n-k$ in the double sum,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}
\\ =\sum_{m=0}^{\infty}\sum_{k=0}^{\infty} (\frac{3}{2})^{k} \frac{2(m)!(2k)!}{8^{m+k} \left [(m)!\right ]^2 \left [(k)!\right ]^2 \left (2(m+k)(1+2k \right )- (1+4k^2))}
\\ =\sum_{m=0}^{\infty} \sum_{k=0}^{\infty} (\frac{1}{2})^{m} (\frac{3}{4})^{k} \frac{(2m)!(2k)!}{4^m 4^k\left [(m)!\right ]^2 \left [(k)!\right ]^2 (2m+1)(2k+1)}
\\ =2\sqrt{\frac{2}{3}}\sum_{m=0}^{\infty} \sum_{k=0}^{\infty} (\frac{1}{\sqrt{2}})^{2m+1} (\frac{\sqrt{3}}{2})^{2k+1} \frac{(2m)!(2k)!}{4^m 4^k\left [(m)!\right ]^2 \left [(k)!\right ]^2 (2m+1)(2k+1)}
\\=2\sqrt{\frac{2}{3}} \sum_{m=0}^{\infty}(\frac{1}{\sqrt{2}})^{2m+1}\frac{(2m)!}{4^m [(m)! ]^2 (2m+1)} \sum_{k=0}^{\infty}(\frac{\sqrt{3}}{2} )^{2k+1}\frac{(2k)!}{4^k[(k)! ]^2 (2k+1)} $$I expand the function$\frac{1} {\sqrt{1-x^2}}$ in a binomial series,$$\frac{1} {\sqrt{1-x^{2}}}=\sum_{n=0}^{\infty}\begin{pmatrix}
\frac{-1}{2} \\
n
\end{pmatrix} x^{2n} =\sum_{n=0}^{\infty}\frac{(2n)!}{4^n(n!)^2}x^{2n}$$and I recall that,$$\sin^{-1}(x)=\int_0^x \frac{dt}{\sqrt{1-t^2}}
\\=\sum_{n=0}^{\infty} \int_0^x\frac{(2n)!}{4^n(n!)^2}x^{2n}dx
\\=\sum_{n=0}^{\infty} \frac{(2n)!}{4^n(n!)^2(2n+1)}x^{2n +1}$$On comparison with the double sum I deduce,$$\sum_{n=0}^{\infty}\sum_{k=0}^n (\frac{3}{2})^k \frac{(2n-2k)!(2k)!}{8^n \left [(n-k)!\right ]^2 \left [(k)!\right ]^2 \left (2n(1+2k \right )- (1+4k^2))}
\\=2\sqrt{\frac{2}{3}}\sin^{-1} (\frac{1}{\sqrt{2}})\sin^{-1} (\frac{\sqrt{3}}{2})$$

Very good! Which number is that?

 

[Fred Wright]

@fresh_42 Problem 7

 

[fresh_42]

@fresh_42 Problem 7

No, I'm asking for the actual result. Which number is your expression?

 

[Fred Wright]

No, I'm asking for the actual result. Which number is your expression?

Silly me. $\sin^{-1}(\frac{1}{\sqrt{2}})=\frac{\pi}{4}$ and $\sin^{-1}(\frac{\sqrt{3}}{2})=\cos^{-1}(\frac{1}{2})=\frac{\pi}{3}$ so I get $\frac{1}{6} \sqrt{\frac{2}{3}} \pi$.
Thank you for posting interesting and difficult problems and commenting on their solutions. It reflects the breadth of your knowledge and patience, and I admire you for that.

 

[fresh_42]

Silly me. $\sin^{-1}(\frac{1}{\sqrt{2}})=\frac{\pi}{4}$ and $\sin^{-1}(\frac{\sqrt{3}}{2})=\cos^{-1}(\frac{1}{2})=\frac{\pi}{3}$ so I get $\frac{1}{6} \sqrt{\frac{2}{3}} \pi$.
Thank you for posting interesting and difficult problems and commenting on their solutions. It reflects the breadth of your knowledge and patience, and I admire you for that.

Not silly, but not quite right (forgotten the square ):
$$
2\sqrt{\frac{2}{3}}\sin^{-1} \left(\frac{1}{\sqrt{2}}\right)\sin^{-1} \left(\frac{\sqrt{3}}{2}\right)=2\sqrt{\frac{2}{3}}\dfrac{\pi}{4}\dfrac{\pi}{3}= \dfrac{\pi^2}{6}\sqrt{\dfrac{2}{3}}=\dfrac{1}{3\sqrt{6}}\,\pi^2
$$

 

[Fred Wright]

Not silly, but not quite right (forgotten the square):
$$
2\sqrt{\frac{2}{3}}\sin^{-1} \left(\frac{1}{\sqrt{2}}\right)\sin^{-1} \left(\frac{\sqrt{3}}{2}\right)=2\sqrt{\frac{2}{3}}\dfrac{\pi}{4}\dfrac{\pi}{3}= \dfrac{\pi^2}{6}\sqrt{\dfrac{2}{3}}=\dfrac{1}{3\sqrt{6}}\,\pi^2
$$

I guess my not being able to do 5th grade arithmetic is not silly but rather indicative of onset dementia.