Nonsensical representation of gravity

[Simon Bridge]

This would be fairly standard:

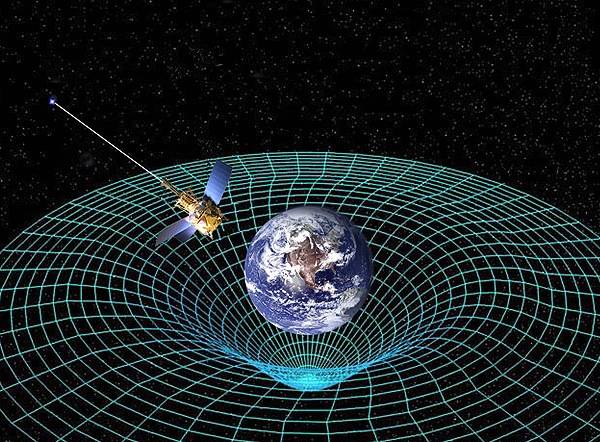
... to improve on it, for educational purposes, I'd want to remove the graphics of the Earth, the spacecraft , and the stars; add axis and label them.

I'd want to make explicit what the lines represent.
Perhaps the height of the sheet depicted represents the curvature of space-time at different 2D space coordinates on a cartesian plane passing close to the position of the Earth? Or a single sheet of the geodesic near the Earth (whatever that means?

The article in question is not clear what it means but is clear that it is entirely a fanciful illustration.

If we want to turn a 2D picture like this into a 3D one, we'd layer many of these sheets - stack them. The picture would look like the a planet making a bulge in a grid.

Would this be better?
It creates the impression of gravity as a pushing rather than a pulling effect though doesn't it? See... you can't win.

 

[Jimmy Snyder]

I doubt that Simon's image is one that #Thomas# would prefer. However, I find it has a very nice feature. You can see the curvature change polarity at the equator. This is correct.

 

[zoobyshoe]

I doubt that Simon's image is one that #Thomas# would prefer. However, I find it has a very nice feature. You can see the curvature change polarity at the equator. This is correct.

Are you talking about the bulge 'upward' right before the plunge 'downward'?

 

[Jimmy Snyder]

Are you talking about the bulge 'upward' right before the plunge 'downward'?

Actually, I've changed my mind about this too. The image is actually quite misleading. It seems to take two geodesic lines, one headed north of the earth, the other south, and cuts them in half at their intersection point to create the impression that they are a single geodesic.

The curvature of spacetime is a 4-dimensional affair. That is why geodesics are ellipses or hyperbolae depending on the speed of the smaller object. The grid lines in Simon Bridge's image consist of circles, which would be suitable for planets, and radial lines which would be suitable for light. This mixture of different types of geodesics in a single image is probably not helpful. What's worse is that the center of the Earth should be at the focal point (center) of the circles, and it is not.

 

[Simon Bridge]

... assuming it's intended to represent the geodesic.

I was quite curious about the bulge before the plunge - if that was just for dramatic effect. Most of the others look like they are just representations of gravitational potential samples across the surface of a plane that does not cross the surface of the Earth. The potential does come from the curvature so they are not lying exactly, but it is not the same as the geodesic either. The lines, therefore, are just a mesh-plot in polar coordinates.

 

[Jimmy Snyder]

In my opinion, the best image would be a set of two dimensional images. Each image would have vertical and horizontal geodesic lines bulged out at the center where the Earth is. Geodesic lines always are planar and the plane intersects the center of the earth, so no information would be imparted by a third dimension. Spherical symmetry would take care of that anyway. There should be several such images with greater and lesser degrees of curvature indicating the effect at different speeds of the smaller object.

 

[Containment]

I actually agree with this I remember the first time I saw an image of what the gravitational field of the Earth would look like and thought to myself "wow as a race are we really this bad at physics?" That was before I realized that the people that make most of the physics models probably have little to no understanding of what's actually happening or they just don't want to spend the time to make the models look good. Like in the case of school books where you might first run into these the company's most likely came up with the artwork. Also I happened to get my hands on a 9th graders science textbook a few years back when I was "babysitting" and the pictures in it are many times better the ones I had back in the good old days.

 

[Darkduke]

I think they use that representation becaue try as they might no one can come up with a better one.
I would like to undersand gravity better to but if the top theoretical physicists can't rap there heads round it what chance do i have lol
They say its a 2D representation of a 3D image if that's the case the 3D image would just be a ball with everything moving to the center but then why would a object circle the mass in the middle surley it would just head directly to the center. Unless it had another force that initiated the circular motion before hand.
If I am making no sense please feel free to tell me.

 

[Simon Bridge]

That's why the mass-geodesics are ellipses.

The problem is not so much modelling it so the top guys can understand it - but coming up with a picture that let's everyone else grasp some of the fundamentals ... to do that you have to sacrifice something.

The mass on a sheet metaphor is supposed to exploit peoples intuitive ideas about gravity to help them understand how something "curving" can lead to attraction and curved paths and so on.

So it is an analogy.
Two weights close together will roll together for example - not the same way that it happens with gravity but they come together. Scoot a small pellet across the sheet and it gets deflected by the bigger masses - line it up right and you can get it to go right around the bigger mass. And so on - it can be quite seductive.

However - you put a grid on the relaxed sheet and then put masses on it - the grid-lines bend towards the mass... no so helpful. However, what works better at the higher level risks losing the regular folk.

Like I said before, I don't think the pics we regularly see are supposed to be geodesics.
More something like this:

This is like those QM energy-level diagrams that also show the wavefunction using the level as an axis. The vertical dimension of each sheet is the gravitational potential at the horizontal coordinate on a plane that passes near a mass. The spheroid in the center represents the mass, not to scale, and I have sampled 4 parallel planes.

Flipping the direction of +V for the upper two sheets is for artistic license :), stops the top plots from obscuring the "planet", and reinforces the impression of the mass bending the space around itself.

I could probably do better by making the sheets slightly transparent.
It's a nasty fudge anyway but I think you can see what I mean.

 

[#Thomas#]

Now I see what's been bothering you people. You want the field lines to represent the field of gravity but not the path that an imaginary object would take while passing through the field...

So if i assume correctly, if an object would follow the red plain in the image posted above, it would be attracted by gravity towards the planet as it hits the "lump"?

I can see the difference now!
http://www.usefulbusiness.co.uk/storage/post-images/BigIdea.jpg?__SQUARESPACE_CACHEVERSION=1303031431987

 

[Jimmy Snyder]

Now I see what's been bothering you people. You want the field lines to represent the field of gravity but not the path that an imaginary object would take while passing through the field...

That is the exact opposite of what I have been saying. The path that an imaginary object would take while passing through the field is called a geodesic. I want the lines to represent geodesics.

 

[Simon Bridge]

#Thomas#: sort of - Jimmy would prefer the representation to show the geodesics, I'm saying that whatever any of us would prefer or expect from these pictures, they are not supposed to represent geodesics: not directly anyhow.

The red sheet represents the gravitational potential sampled at discrete x-y positions on a plane parallel to the x-y plane (through the center of the mass at z=+2.5 units. The magnitude of the potential is proportional to the displayed displacement of the sheet from z=2.5. The sign of the potential is always negative: so it doesn't matter which way up I draw the sheets.

Aside: the spheroid representing the mass is not drawn to scale.

I am hoping to illustrate that this is the sort of thing that is commonly depicted artistically - if not exactly what the artist has in mind.

The purpose is to illustrate a very narrow set of core ideas about gravity.

And you are right - an imaginary object hitting the bump will experience an attractive force towards the mass. Just not what Jimmy's going on about.

I'm trying to figure out how to graph the geodesics. For a mass, the exact paths would depend on the speed so this kind of 3-space sampling wouldn't be useful. Plotting the light-paths may be illustrative ... or maybe graphing the overall curvature at different points? Jimmy - help?

I've also been unsuccessful at getting octave to draw semi-transparent mesh or surface plots like MATLAB can. Anyone know how (or if) you use surface properties in octave?
In fact, I don't even know how to plot a set of ordered triples in octave.

 

[zoobyshoe]

That is the exact opposite of what I have been saying. The path that an imaginary object would take while passing through the field is called a geodesic. I want the lines to represent geodesics.

Wouldn't this just be the trajectory of a body in motion?

http://www.sciencephoto.com/media/363714/enlarge

 

[Simon Bridge]

Those would be the Newtonian trajectories.
Now do it for GR in a way that illustrates to the layman the way masses curve space-time :)

 

[zoobyshoe]

Those would be the Newtonian trajectories.

That's what I'm saying/asking: I've read it asserted that objects take the Newtonian trajectories because they are following the geodesic created by "curved" space-time. I'm thinking/asking if the representation of a specific geodesic (or a sampling of many geodesics) would be informative since it would be nothing but a Newtonian trajectory.

Now do it for GR in a way that illustrates to the layman the way masses curve space-time :)

Yes, this is the challenge.

 

[Simon Bridge]

Almost: the Newtonian trajectory is the projection of the 4D geodesic onto 3D space.

Also see the pic in Wikipedia's Spacetime article:

Two-dimensional analogy of spacetime distortion.
Matter changes the geometry of spacetime, this (curved) geometry being interpreted as gravity.
White lines do not represent the curvature of space but instead represent the coordinate
system imposed on the curved spacetime, which would be rectilinear in a flat spacetime.
... this would be a careful description of what the rubber-sheet models are trying to represent.

And yep - to come up with a representation of space-time curvature in 3D space that preserves important aspects of the GR treatment while being accessible to the layman or to resign ourselves to an endless repetition of the same old flawed artistic renderings and deal with the confusions that are so generated ... that is the question.

I tell you: Shakespear had it easy!

 

[Jimmy Snyder]

I'm trying to figure out how to graph the geodesics. For a mass, the exact paths would depend on the speed so this kind of 3-space sampling wouldn't be useful. Plotting the light-paths may be illustrative ... or maybe graphing the overall curvature at different points? Jimmy - help?

I think a grid of geodesics is best. The reason I say that is that geodesics are the straight lines in curved space and hence show the curvature of space. There is a formula for the geodesics, I don't know what it is though. With such a formula, you should be able to map them easily. The curvature of space is 4 dimensional curvature. For this reason, you would need to have several images for various speeds. You might consider making a movie with the first frame being the geodesics for low speed and the last frame being for light speed. thus using time as the 4th dimension. A novel idea, but I'm not sure it wouldn't be misleading.

Assume that the mass of the body that is traveling along the geodesic is small. The mass of the gravitating body also has an effect on the geodesics. In the case of a black hole, some of the geodesics probably have no counterparts in less exotic fields. You might consider making a movie of light geodesics starting with our sun and gradually increasing density up to a black hole. This might be a better idea than the movie I suggested above.

 

[Simon Bridge]

Let me know when you find an equation in a form I can use.
If I use those for a very small test mass won't that just give me the light-lines?

I'm also going to need a better renderer - it seems that octave now uses an OpenGL renderer and devs have yet to implement an alpha-mapping for it. Something I'm going to need for meshes with holes in them.

(may be possible with patches)
But we seem to have moved away from the original question and into how to do different representations - constructive but I think it may be for another thread.
I posted by big graphic because it seemed unfair to be telling people to come up with their own without doing it myself.

I suspect the original question has been answered.

 

[#Thomas#]

That is the exact opposite of what I have been saying. The path that an imaginary object would take while passing through the field is called a geodesic. I want the lines to represent geodesics.

It would seem we lack consences here. Although I respect your preferences in this matter, there must be way to represent an image to the enthusiast without causing too much confusion as it did in me, but on the other hand this could only be me, becuase in my language words such a "geodesics" can translate into multiple meanings which could all apply to this topic... i need to loose my habbit of allways using the most commonly used translation and consider all alternatives.

 

[Jimmy Snyder]

If I use those for a very small test mass won't that just give me the light-lines?

No, the geodesics do not depend on the test mass as long as that mass is small. It only depends on the speed. However, no test mass can travel at light speed.

To illustrate this dependency, remember that when Eddington photographed the eclipse in 1919, he found that starlight had been deflected 1.73 seconds of arc from their non-deflected positions. This indicates a very slight curvature for the geodesics of light (travelling, of course, at light speed) grazing the surface of the sun. However, the Earth itself also travels (at much less speed) in a geodesic 93 million miles from the surface of the sun and the curvature is so great as to bend the path into an ellipse. Also, consider the so-called asteroid belt where millions of fragments with a wide range of masses are all traveling basically along the same geodesic. That is because there is not such a wide range of speeds among the asteroids in the belt. We know that their range of speeds is small because if not then they would be colliding like crazy and showering the Earth with dinosaur killers on a regular basis.

I do have a pair of equations for geodesics, but I don't completely understand it. It comes from the book "The Einstein Theory of Relativity" by Lieber and Lieber. It also give the Newtonian equations for reference. Note that they give the geodesic in terms of polar coordinates r and $\phi$. This is compatible with what I have said before, namely, that you can draw a strictly two dimensional image. Spherical symmetry will take care of the rest. Also notice that the mass of the gravitating body, m, is used, but not the test mass.

Newton:
$$\frac{d^2u}{d\phi^2} + u = \frac{m}{h^2}$$
$$r^2\frac{d\phi}{dt} = h$$

Einstein:
$$\frac{d^2u}{d\phi^2} + u = \frac{m}{h^2} + 3mu^2$$
$$r^2\frac{d\phi}{ds} = h$$

Where h is a constant of integration, r is the radius, $\phi$ is the angle of rotation, and u = 1/r. The authors point out that there is an extra term $3mu^2$ in the first Einstein equation and that differentiation is with respect to proper time s for Einstein, but with repect to time t for Newton.

 

[#Thomas#]

No, the geodesics do not depend on the test mass as long as that mass is small. It only depends on the speed. However, no test mass can travel at light speed.

Actually recent experiments suggest that neutrinos can, in fact, move faster than light! Which was, in my humple oppinion, and adequate kick in the butt for those complacent CERN scientists... but this isn't what this topic is about...

Here, have a look at this:
http://www.nasa.gov/mpg/146898main_viz_shiftingall_21.320x240.mpg

Surely NASA would satisfy your strict standards!

 

[Simon Bridge]

Actually recent experiments suggest that neutrinos can, in fact, move faster than light!

Actually, recent experiments suggest that we need to look much harder at how neutrino experiments are done. But you are correct, there are other threads for that. In this thread, we are discussing traditional representations of Einstein space-time. After all, we could try for a representation using string theory too but that would also be off topic.

Surely NASA would satisfy your strict standards!

You mean the guys who crash spacecraft ? They also use misleading images as part of PR. (Hmmm ... can't seem to play the mpg.)

in my language words such a "geodesics" can translate into multiple meanings which could all apply to this topic

... same in English: context is everything. "Geodesic" is a term of the art[1] in general relativity where we have to describe paths in 4 dimensions. There is a lot of this in physics - "work" has several meanings too.

When people put up these pics they usually make some glib comment about showing the "shape of space time" or similar without actually defining their terms. Whenever you see this, you are not expected to take it seriously.

NASA, for example, produce a range of documents with a wide range of rigour. You will see the rubber-sheet pics where they are talking to Joe Public, a mass of equations when they are talking to scientists, and brightly colored drawings with baby-talk and smiley-faces for talking to Congressmen.

------------------------------------------
[1] i.e. jargon - this is a useful phrase I got off a lawyer.

 

[Simon Bridge]

Here's another, related, artistic impression that has misleading features. This time about the shape of the Universe.
https://www.physicsforums.com/showthread.php?t=298279
... shoud help illustrate what these sorts of drawings are supposed to be doing.

 

[#Thomas#]

Actually
You mean the guys who crash spacecraft ? They also use misleading images as part of PR. (Hmmm ... can't seem to play the mpg.)

Actually this isn't some artist's expression, this is crunch time of a supercomputer calculating how gravity would put 2 black holes together and release gravity wawes.