Nuclear Macroscopic cross-section

[badvot]

Homework Statement

Calculate the macroscopic cross section using volume fractions

Relevant Equations

Macroscopic nuclear cross section

This question is in the book " Introduction to nuclear engineering by Lamarsh" Chapter3:

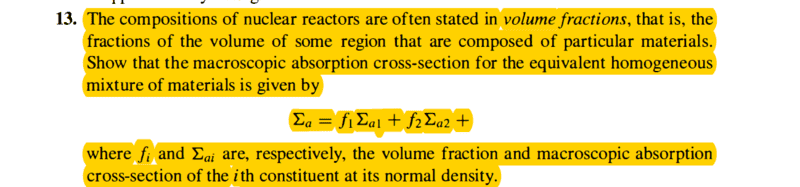
I think it's pretty basic but I couldn't find the proper way to prove it, and now I even suspect that it's not a correct question.
My attempt solution:

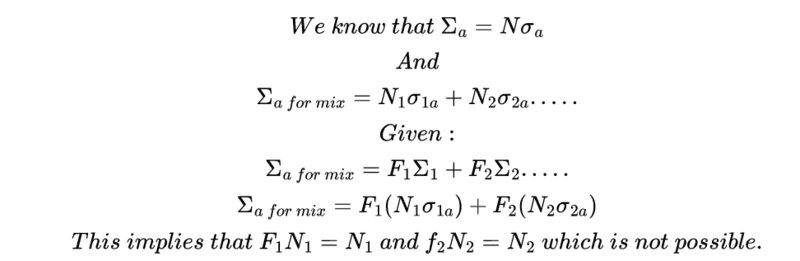
I would very much appreciate your help. Thanks in advance.

 

[TSny]

You need to distinguish between the number density $N_i^{(mix)}$ of constituent $i$ in the mixture and the number density $N_i^{(norm)}$ of constituent $i$ at its normal density.

How is $N_i^{(mix)}$ related to $N_i^{(norm)}$ and $f_i$?

You are asked to show, $$\Sigma_a^{(mix)} = f_1 \Sigma_{a1}^{(norm)} + f_2 \Sigma_{a2}^{(norm)} + ...$$

 

[badvot]

Thank you so much. I think I get it now, and here is my understanding:
If we assume two species (x,y)
$$\Sigma_{mix}= \frac{No\ of\ X\ atoms}{Volume\ of\ X} \times \frac{Volume\ of\ X}{Volume\ of\ X+Y} + \frac{No\ of\ Y\ atoms}{Volume\ of\ Y} \times \frac{Volume\ of\ Y}{Volume\ of\ X+Y}$$

 

[TSny]

Thank you so much. I think I get it now, and here is my understanding:
If we assume two species (x,y)
$$\Sigma_{mix}= \frac{No\ of\ X\ atoms}{Volume\ of\ X} \times \frac{Volume\ of\ X}{Volume\ of\ X+Y} + \frac{No\ of\ Y\ atoms}{Volume\ of\ Y} \times \frac{Volume\ of\ Y}{Volume\ of\ X+Y}$$

The left side of your equation, $\Sigma_{mix}$, is the macroscopic absorption cross-section of the mixture. This has the dimension of inverse length. However, the right side of your equation has the dimension of inverse volume.

Also, you would expect $\Sigma_{mix}$ to depend on the microscopic absorption cross-sections $\sigma_{a1}$ and $\sigma_{a2}$ of the two species. However, these do not appear on the right side.

 

[badvot]

Oh, I guess I was so enthusiastic to write the answer that I forgot to include the microscopic cross-section for each species
$$\Sigma_{mix}= \frac{No\ of\ X\ atoms}{Volume\ of\ X} \times
\frac{Volume\ of\ X}{Volume\ of\ X+Y} \times \sigma_{X} + \frac{No\ of\ Y\ atoms}{Volume\ of\ Y} \times \frac{Volume\ of\ Y}{Volume\ of\ X+Y} \times \sigma_{Y}$$
The volume fractions are those below, while the rest of the terms $\sigma \times \frac{No\ of\ atoms}{Volume}$ equal the $\Sigma_{a}^{(norm)}$ for each species
$$\frac{Volume\ of\ X}{Volume\ of\ X+Y} =F_{X}$$
$$\frac{Volume\ of\ Y}{Volume\ of\ X+Y} =F_{Y}$$
The units check out
$$cm^{-1}= \frac{\#}{cm^{3}} \times \frac{cm^{3}}{cm^{3}} \times cm^{2} + \frac{\#}{cm^{3}} \times \frac{cm^{3}}{cm^{3}} \times cm^{2} $$

 

[TSny]

OK. That looks good.