Outer measure of an open interval ....

[Math Amateur]

I am reading Sheldon Axler's book: Measure, Integration & Real Analysis ... and I am focused on Chapter 2: Measures ...

I need help with proving that the outer measure of an open interval, \mid (a, b) \mid = b - a

Axler's definitions of length and outer measure are as follows:

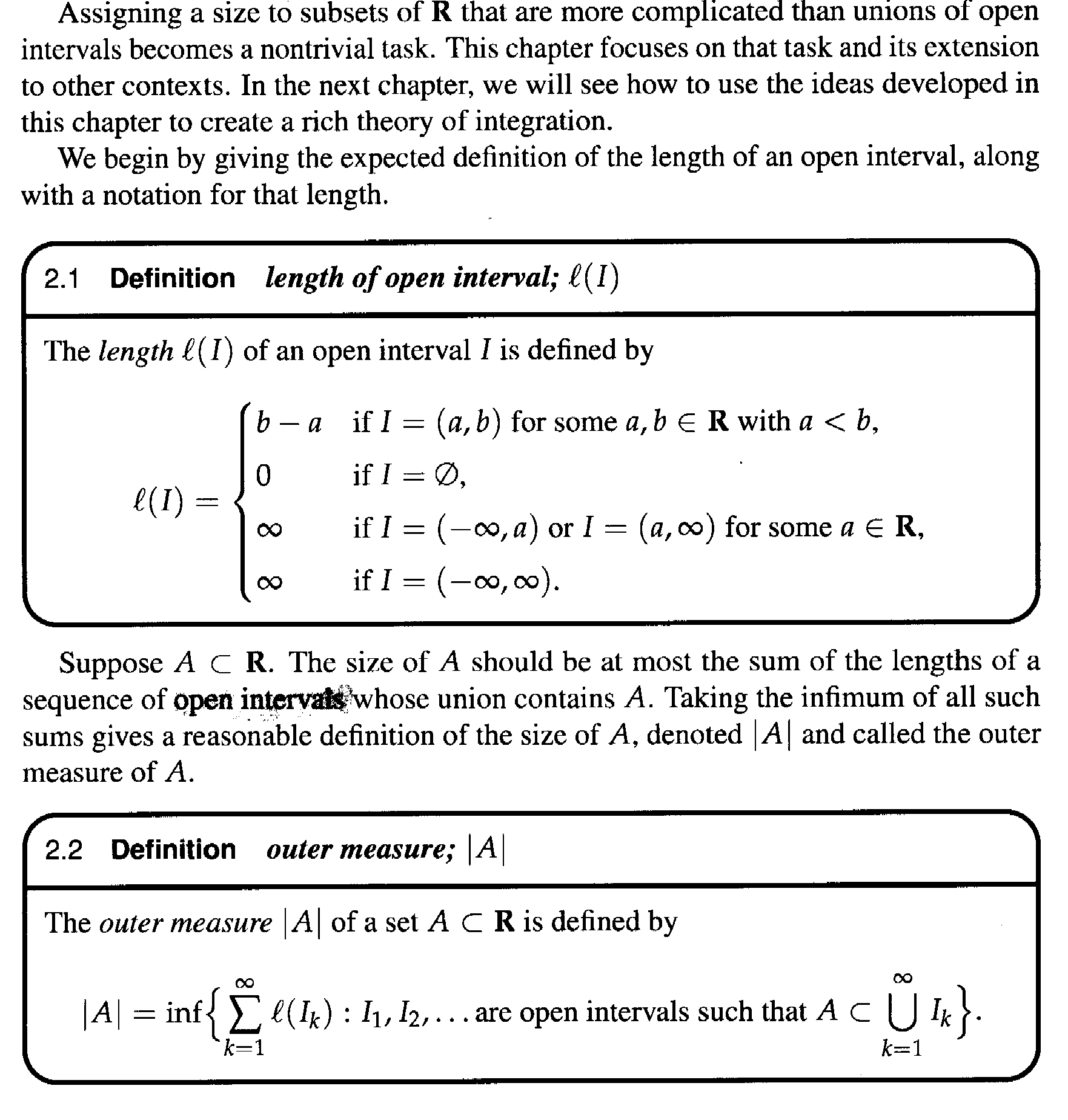
Can someone demonstrate rigorously that $$ \mid (a, b) \mid = b - a$$ ...

I know it seems intuitively obvious but how would you express a convincing and rigorous proof of the above result ...
Help will be much appreciated ... ...

Peter

 

[GJA]

Hi Peter,

I agree with you here. For something that seems so intuitively clear, the proof isn't a one-liner using only the definition. My suggestion would be to try and use the result you asked about in another post, namely that $\vert[a,b]\vert = b-a$. Also think about approximating $(a,b)$ using closed intervals contained in $(a,b)$. These two ideas together are enough to formulate a short argument proving $\vert(a,b)\vert = b-a.$ I'll leave it at this for now, but feel free to let me know if you have additional questions.

 

[Math Amateur]

Thanks for the help GJA ...

BUT ... still struggling with constructing a proof ...

Can you help further ...

Peter

 

[GJA]

Certainly. Let's approximate $(a,b)$ via the closed intervals $[a+1/2n, b-1/2n].$ We then have $b - a - 1/n = \vert[a+1/2n, b - 1/2n]\vert\leq \vert (a,b)\vert$ for all $n$ (large enough), because $[a+1/2n, b-1/2n]\subset (a,b)$ for all $n$ (large enough). Since this holds for all $n$ (large enough), it follows that $b-a\leq \vert(a,b)\vert.$ Can you think of an argument that would show $\vert(a,b)\vert \leq b-a$? Hint: $(a,b)$ itself is an open interval that contains $(a,b)$. Think about this and the definition of outer measure.

Edit: I needed to add the qualifier "large enough" because $[a+1/2n, b-1/2n]$ may not be contained in $(a,b)$ for all $n$. However, since $a<b$, there is $N$ such that $[a+1/2n, b-1/2n]\subset (a,b)$ for all $n\geq N$; i.e., for all $n$ "large enough."

 

[Math Amateur]

Certainly. Let's approximate $(a,b)$ via the closed intervals $[a+1/2n, b-1/2n].$ We then have $b - a - 1/n = \vert[a+1/2n, b - 1/2n]\vert\leq \vert (a,b)\vert$ for all $n$ (large enough), because $[a+1/2n, b-1/2n]\subset (a,b)$ for all $n$ (large enough). Since this holds for all $n$ (large enough), it follows that $b-a\leq \vert(a,b)\vert.$ Can you think of an argument that would show $\vert(a,b)\vert \leq b-a$? Hint: $(a,b)$ itself is an open interval that contains $(a,b)$. Think about this and the definition of outer measure.

Edit: I needed to add the qualifier "large enough" because $[a+1/2n, b-1/2n]$ may not be contained in $(a,b)$ for all $n$. However, since $a<b$, there is $N$ such that $[a+1/2n, b-1/2n]\subset (a,b)$ for all $n\geq N$; i.e., for all $n$ "large enough."

Thanks for the help GJA ...

To show that $\vert(a,b)\vert \leq b-a$ ... we proceed as follows ...

We have I_1, I_2, ... = $(a,b), \emptyset, \emptyset,$ ... ... is a covering of $(a,b)$ ... ...

Therefore $(a,b)$ is a lower bound for $\sum_{n=1}^{\infty} I_n = b - a$ ... ...

... so ... $\vert(a,b)\vert \leq b-a$ ...Is that correct?

Peter

 

[GJA]

A few things to note. First, we wouldn't say $(a,b)$ is a lower bound because it's an interval, not a number. Next, we can only say something is a lower bound for a set when it's a value below all the numbers in the set. For example $3$ is a lower bound for the set $(5,10)$ but $9$ is not. Since you chose a specific covering of $(a,b)$ and not an arbitrary one, you cannot conclude that the value you came up with is a lower bound for $\{\sum_{k}l(I_{k})\, :\,\ldots\}$, because you don't know that the covering you chose produces a value that is smaller than all the values in the set. What you have shown is that $b-a$ belongs to the set of values $\{\sum_{k}l(I_{k})\,:\,\ldots\}$ and, therefore, $\vert[a,b]\vert\leq b-a.$

 

[HOI]

A few things to note. First, we wouldn't say $(a,b)$ is a lower bound because it's an interval, not a number. Next, we can only say something is a lower bound for a set when it's a value below all the numbers in the set.

Below or equal to every number in the set.[/quote]

For example $3$ is a lower bound for the set $(5,10)$[//quote]
and so is 5.

but $9$ is not. Since you chose a specific covering of $(a,b)$ and not an arbitrary one, you cannot conclude that the value you came up with is a lower bound for $\{\sum_{k}l(I_{k})\, :\,\ldots\}$, because you don't know that the covering you chose produces a value that is smaller than all the values in the set. What you have shown is that $b-a$ belongs to the set of values $\{\sum_{k}l(I_{k})\,:\,\ldots\}$ and, therefore, $\vert[a,b]\vert\leq b-a.$

 

[Math Amateur]

A few things to note. First, we wouldn't say $(a,b)$ is a lower bound because it's an interval, not a number. Next, we can only say something is a lower bound for a set when it's a value below all the numbers in the set. For example $3$ is a lower bound for the set $(5,10)$ but $9$ is not. Since you chose a specific covering of $(a,b)$ and not an arbitrary one, you cannot conclude that the value you came up with is a lower bound for $\{\sum_{k}l(I_{k})\, :\,\ldots\}$, because you don't know that the covering you chose produces a value that is smaller than all the values in the set. What you have shown is that $b-a$ belongs to the set of values $\{\sum_{k}l(I_{k})\,:\,\ldots\}$ and, therefore, $\vert[a,b]\vert\leq b-a.$

Thanks GJA ...

Sorry ... I have confused things with a typo ... I wrote $(a,b)$ when I meant $\mid (a, b) \mid$ ... ...

So ... my post should have read as follows:

To show that $\vert(a,b)\vert \leq b-a$ ... we proceed as follows ...

We have $I_1, I_2, ... = (a,b), \emptyset, \emptyset,$ ... ... is a covering of $(a,b)$ ... ...

Therefore $\vert(a,b)\vert$ is a lower bound for $\sum_{n=1}^{\infty} I_n = b - a$ ... ...

... so ... $\vert(a,b)\vert \leq b-a$ ...
A word about the claim that " ... Therefore $\vert(a,b)\vert$ is a lower bound for $\sum_{n=1}^{\infty} I_n = b - a$ ... ..."

... $\vert(a,b)\vert$ is a lower bound for the set of all sums of the form $\sum_{n=1}^{\infty} I_n$ ... (indeed, it is the greatest lower bound ...) ... so it is less than the particular sum mentioned ...

Is the above correct ... apart from referring to a lower bound of a number rather than a set of numbers ...

Peter