- #71
Moose100
- 116
- 0
OK I am posting the pics of my notes. This text isn't helping me get this across this is for the first problem 16.32
At "part 3"i did use a polynomial calculator. Where one of the roots is x=-0.032. I wanted to make sure that you saw I was heeding your advice.
Also the values in the mass action ratio reflect me multiplying the equilibrium values by 2 to reflect a doubling in pressure that comes from moving in the the piston halfway.

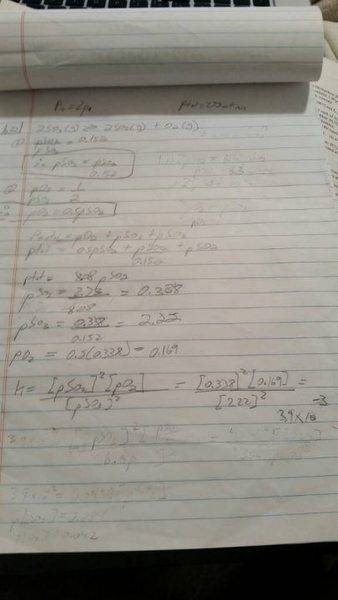
![20150920_174543[1].jpg 20150920_174543[1].jpg](https://www.physicsforums.com/attachments/20150920_174543-1-jpg.89011/)
At "part 3"i did use a polynomial calculator. Where one of the roots is x=-0.032. I wanted to make sure that you saw I was heeding your advice.
Also the values in the mass action ratio reflect me multiplying the equilibrium values by 2 to reflect a doubling in pressure that comes from moving in the the piston halfway.
Last edited: