- #71
The Tortoise-Man
- 95
- 5
Baluncore said:Your irrational line breaks make it hard to understand your reasoning.
If the only losses were the series resistance of the inductor, or the dielectric losses of the capacitor, then the wikipedia simplicity would be correct.
But in the real world there are other networks in parallel with L and C that lower the Q. There is no advantage in having an isolated tuned circuit if it does not have energy inputs and energy outputs.
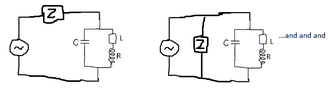
Ok, maybe I understand your point and the reason for my confusion now (correct
me if I'm wrong):
Seemingly we talked past each other on the point what the considered "tuned circuit" should be.
So I guess that you implicitely considered the antenna impedance + the coupling capacitor
as part of it, while I (wrongly?) assumed that when you talked about "tuned circuit" you literally
only mean this LC-thing. In pictures:
Well, if you indeed meant by "tuned circuit" the blue encircled thing, then clearly it's Q depends on the coupling capacitor (I conjecture that you meant exactly this thing because as you said the Q of it should depend on antenna losses, while the Q of the green encircled thing has - as wiki formula says - no dependence on the antenna components & coupling capacitor. Did understood your point now correctly?