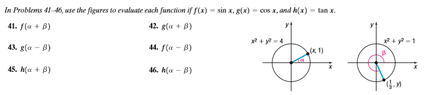Solve $\sin(\alpha + \beta)$: Answer #41

[karush]

View attachment 7029

trying to do #41

\begin{align*}\displaystyle
\sin(\alpha + \beta)
&=\sin\alpha \cos \beta
+\cos \alpha \sin \beta\\
&=
\frac{1}{4}\cdot\frac{3}{1}
+\frac{\sqrt{15}}{4}\cdot \frac{\sqrt{10}}{3}
\end{align*}

ok the book answer to this was

$$f(\alpha + \beta)=\frac{1-2\sqrt{6}}{6}$$

but I couldn't derive this

 

[MarkFL]

From the graph on the left, we have:

$$2\sin(\alpha)=1\implies \sin(\alpha)=\frac{1}{2}$$-

Hence:

$$\cos(\alpha)=\sqrt{1-\left(\frac{1}{2}\right)^2}=\frac{\sqrt{3}}{2}$$

And from the graph on the right:

$$\cos(\beta)=\frac{1}{3}$$

Hence (observing the terminal side of the angle is in the 4th quadrant):

$$\sin(\beta)=-\sqrt{1-\left(\frac{1}{3}\right)^2}=-\frac{2\sqrt{2}}{3}$$

And so we find:

$$f(\alpha+\beta)=\sin(\alpha+\beta)=\sin(\alpha)\cos(\beta)+\cos(\alpha)\sin(\beta)=\frac{1}{2}\cdot\frac{1}{3}-\frac{\sqrt{3}}{2}\cdot\frac{2\sqrt{2}}{3}=\frac{1-2\sqrt{6}}{6}$$

 

[karush]

ok I had r=4 not r=2

(Headbang)ok if I can sneak another one in here

$$\displaystyle\sin\left({2\theta}\right)= \frac{1}{3}$$
$$3\sin\left({2\theta}\right) = 1$$

then ?

 

[MarkFL]

ok I had r=4 not r=2

(Headbang)ok if I can sneak another one in here

$$\displaystyle\sin\left({2\theta}\right)= \frac{1}{3}$$
$$3\sin\left({2\theta}\right) = 1$$

then ?

I'm assuming you are to solve for $\theta$...in which case I would observe that there are solutions in quadrant I and quadrant II, and they are symmetrical about $$\alpha=\frac{\pi}{2}$$, and we must keep the periodicity of the sine function in mind, hence:

$$2\theta=\left(\frac{\pi}{2}\pm\arccos\left(\frac{1}{3}\right)\right)+2k\pi$$ where $k\in\mathbb{Z}$

And so we have:

$$\theta=\frac{1}{2}\left(\frac{\pi}{2}\pm\arccos\left(\frac{1}{3}\right)\right)+k\pi=\frac{\pi}{4}(4k+1)\pm\frac{1}{2}\arccos\left(\frac{1}{3}\right)$$

 

[karush]

why $\arccos\left(\frac{1}{3}\right)$ ?

 

[MarkFL]

why $\arccos\left(\frac{1}{3}\right)$ ?

If we have:

$$\sin(\alpha)=\frac{1}{3}$$

But, we want the angle between the terminal side of $\alpha$ and $$\frac{\pi}{2}$$...that is we want the complementary angle to $\alpha$. :)