- #1
chwala
Gold Member
- 2,745
- 387
- Homework Statement
- This is apast paper question. Allow me to post it as it is.
- Relevant Equations
- Probability
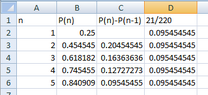
Okay, i was able to solve it by trial and error, i am seeking for a more concrete approach. Can combination work here? or a more solid approach using sequences? or probability itself?
My trial and error,
##P_{green} = \dfrac{9}{12}×\dfrac{8}{11} ×\dfrac{7}{10}×\dfrac{6}{9}×\dfrac{3}{8} = \dfrac{21}{220}##
##n=5##.
and why 2 marks? or there is a shorter method.