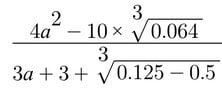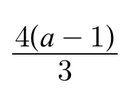STS
- 5
- 0
I don't understand this.
a is not suppose to be -1; this is the only rule in the equation
The answer is the second picture, I just don't know the steps that lead to that answer.
View attachment 8926
a is not suppose to be -1; this is the only rule in the equation
The answer is the second picture, I just don't know the steps that lead to that answer.
View attachment 8926