brotherbobby
- 750
- 169
- Homework Statement
- A 10 km long straight road connects two towns A and B. Two cyclists simultaneously start one from town A and the other from town B. On reaching the opposite town, a cyclist immediately returns to his starting town whereas the other cyclist takes some rest and then returns to his starting town. Both of them can ride at speed 20 km/hr in absence of wind but during their whole journey uniform wind from town 𝐴 to 𝐵 increases the speed of the cyclist going into the wind by the same amount as it decreases the speed of the cyclist going against the wind. Both the cyclists meet twice, first at 2 km and then 6 km away from one of the towns. For what period does a cyclist rest?
- Relevant Equations
- 1. In uniform motion with speed ##v##, the displacement after a time is ##\Delta t## is ##\Delta x=v\Delta t##.
2. If frame ##S'## moves with velocity ##+v## along the ##+xx'## axis in normal configuration, then the velocity of an object in frame ##S## is ##u=u'+v##, where ##u'## is its velocity in frame ##S'##.
Attempt : [I could do the problem but get different answers from that of the text and several other places on the internet where the problem's been solved. I haven't seen their solution(s) yet].
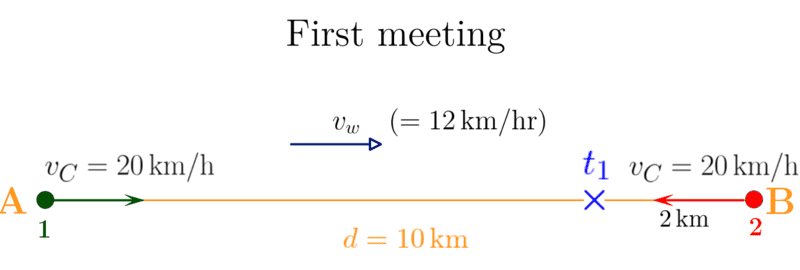
Meeting 1 : I start by drawing an image of the problem for the first meeting the position of which is shown by the blue cross mark (##\color{blue}{\boldsymbol{\times}}##). This meeting should happen 2 km from B because (cyclist) 1 should travel a greater distance (8 km) in the same time as 2 (2 km) due to wind. If this meeting happens at a time ##t_1##, then
##\small{t_1=\dfrac{8}{20+v_w}=\dfrac{2}{20-v_w}\Rightarrow v_w = 12\,\text{km/h}}\quad{\Large{\color{green}\checkmark}}##. This answer matches with the text and I have put it in the diagram.
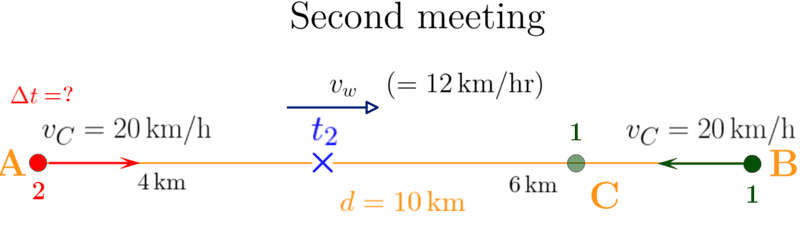
Meeting 2 : Here 2 has come to A and 1 has gone to B. When they start again, they meet at a point 6 km from B or 4 km from A shown by the blue cross mark (##\color{blue}{\boldsymbol{\times}}##). If neither cyclist had waited, they'd meet again at the same place as before, 2 km from B. But because they meet earlier than that, the cyclist from A (cyclist 2) must have lost ground and therefore it must have been him who rested at A (##\color{red}{\large{\boldsymbol\times}}##) for a time ##\Delta t## before starting his journey back to B.
This answer is wrong, as per the text.
During this time, 1 must have covered a distance ##20\Delta t## and reached a point shown as C in the diagram. Hence, when 2 is supposed to start from A, their distance of separation ##\Delta x_2=10-20\Delta t##. But 2 had to travel 4 km, hence the time of the meet ##\small{\Delta t_2=\dfrac{4}{20+12}=\dfrac{1}{8}\,\text{hr}}##. But this is also the time that 1 got to travel from B's side : ##\small{\dfrac{1}{8}=\dfrac{10-20\Delta t-4}{8}\Rightarrow 6-20\Delta t=1\Rightarrow 20\Delta t=5\Rightarrow \boxed{\Delta t =\dfrac{1}{4}\,\text{hr}=15\,\text{mins}}}\quad\color{red}{\large{\boldsymbol\times}}##.
Correct answers : ##\text{The cyclist 1 stops at B for a time of 18.75 mins}##.
Request : Where do you think am I going wrong? Thanks for the time and the trouble.
Meeting 1 : I start by drawing an image of the problem for the first meeting the position of which is shown by the blue cross mark (##\color{blue}{\boldsymbol{\times}}##). This meeting should happen 2 km from B because (cyclist) 1 should travel a greater distance (8 km) in the same time as 2 (2 km) due to wind. If this meeting happens at a time ##t_1##, then
##\small{t_1=\dfrac{8}{20+v_w}=\dfrac{2}{20-v_w}\Rightarrow v_w = 12\,\text{km/h}}\quad{\Large{\color{green}\checkmark}}##. This answer matches with the text and I have put it in the diagram.
Meeting 2 : Here 2 has come to A and 1 has gone to B. When they start again, they meet at a point 6 km from B or 4 km from A shown by the blue cross mark (##\color{blue}{\boldsymbol{\times}}##). If neither cyclist had waited, they'd meet again at the same place as before, 2 km from B. But because they meet earlier than that, the cyclist from A (cyclist 2) must have lost ground and therefore it must have been him who rested at A (##\color{red}{\large{\boldsymbol\times}}##) for a time ##\Delta t## before starting his journey back to B.
This answer is wrong, as per the text.
During this time, 1 must have covered a distance ##20\Delta t## and reached a point shown as C in the diagram. Hence, when 2 is supposed to start from A, their distance of separation ##\Delta x_2=10-20\Delta t##. But 2 had to travel 4 km, hence the time of the meet ##\small{\Delta t_2=\dfrac{4}{20+12}=\dfrac{1}{8}\,\text{hr}}##. But this is also the time that 1 got to travel from B's side : ##\small{\dfrac{1}{8}=\dfrac{10-20\Delta t-4}{8}\Rightarrow 6-20\Delta t=1\Rightarrow 20\Delta t=5\Rightarrow \boxed{\Delta t =\dfrac{1}{4}\,\text{hr}=15\,\text{mins}}}\quad\color{red}{\large{\boldsymbol\times}}##.
Correct answers : ##\text{The cyclist 1 stops at B for a time of 18.75 mins}##.
Request : Where do you think am I going wrong? Thanks for the time and the trouble.
Last edited: