bolzano95
- 89
- 7
- Homework Statement
- What is the force of a wall on a revolvable rod?
- Relevant Equations
- II. Newton Law, Free-Body Diagram
I have a dilemma.
If I look at the diagram and say the sum of the forces in x and y direction has to be zero, then I will simply conclude that the force of a wall on a revolvable rod is the blue N as drawn.
But what if the force is actually the green N? To me, it makes more sense because if imagine no tension and no mass M, then the force of a wall N would be drawn as below (green N).
So how can I argue that the orange component of N does exist? If it exists...
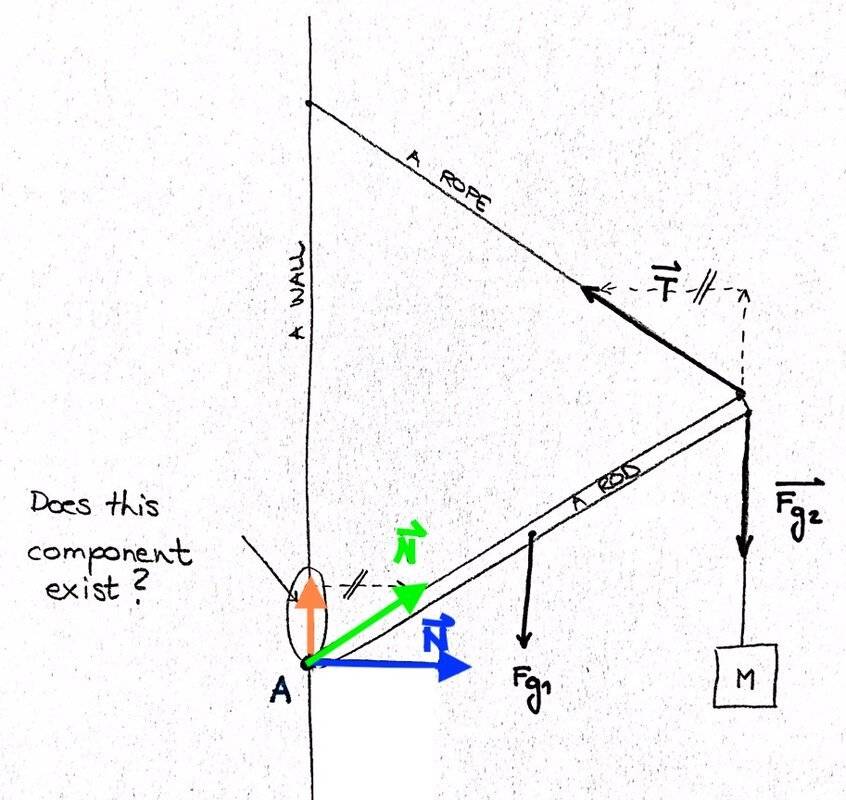
If I look at the diagram and say the sum of the forces in x and y direction has to be zero, then I will simply conclude that the force of a wall on a revolvable rod is the blue N as drawn.
But what if the force is actually the green N? To me, it makes more sense because if imagine no tension and no mass M, then the force of a wall N would be drawn as below (green N).
So how can I argue that the orange component of N does exist? If it exists...