rudransh verma
Gold Member
- 1,067
- 96
- Homework Statement
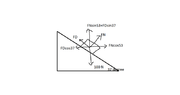
- A block of 100N weight is slowly slid up on a smooth incline of inclination 37 degrees by a person. Calculate the work done by the person in moving the block through a distance of 2m, if the driving force is a) parallel to the incline and b) in the horizontal direction.
- Relevant Equations
- ##W=F.d##
##W=mgh=100(\sin 37)2=-120J## Right answer!
But the question is asking work done by the person. So again I wrote two eqns
##F_N\sin 53+F_D\sin 37-100=10.2a_y##
##F_N\cos 53-F_D\cos 37=-10.2a_x##
I just need ##a_x## and ##a_y## to solve.
But the question is asking work done by the person. So again I wrote two eqns
##F_N\sin 53+F_D\sin 37-100=10.2a_y##
##F_N\cos 53-F_D\cos 37=-10.2a_x##
I just need ##a_x## and ##a_y## to solve.
Attachments
Last edited: