- #1
JDoolin
Gold Member
- 723
- 9
Terrell Revisited: "The Invisibility of the Lorentz Contraction"
I recently posted this response to a general question of how Special Relativity worked.
G H Wells Jr pointed out that
and
Terrell's Argument
Both of these are important issues to take into account. However, they do not change the fact that "a meter stick in motion past the observer in such a way that it is moving parallel to its length, and is momentarily seen by its observer at its point of closest approach, will surely be seen as contracted."
This last quote is directly from an article by James Terrell, in in the 1959 Physical Review "Invisibility of Lorentz Contraction" , but he argues the opposite point: He claims that "the conformality of aberration ensures that, at least over small solid angles, each [co-located observer, regardless of relative motion] will see precisely what the other sees. No Lorentz contractions will be visible, and all objects will appear normal."
Is the Lorentz contraction "invisible" as Terrell claims, or has James Terrell made a mistake which has gone unnoticed for decades?
I'll take some time to analyze Terrell's argument (or lack thereof), and check whether my own methods (analyzing intersections of world-lines and light-cones) agree with his, (transformations of angles via an aberration equation) and if they don't agree, see if I can figure out why.
Terrell finds an aberration equation from the Lorentz transformations, then uses the aberration equation to conclude that the Lorentz contraction effect "vanishes," but I find it suspicious, when by using the Lorentz transformations directly, I find that the Lorentz contraction is quite visible. and when using diagrams from the aberration equation, I still find that the Lorentz contraction is visible.
In fact, even using the aberration equation, it is plain that the objects do not "all appear normal," as Terrell claimed. They definitely have different lengths depending on their relative position, and the relative speed of the observer.
Method Using the Aberration:
I found a nice diagram of the aberration equation here: http://www.mathpages.com/rr/s2-05/2-05.htm which helps me make my point without a lot of math.
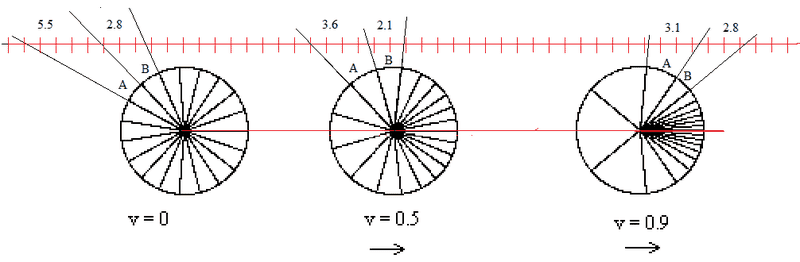
It appears to me, that even using the aberration equations, the Lorentz Contraction is visible, and for certain, it cannot be said that "all objects will appear normal" as Terrell claims.
In the attached diagram you can see that the length of the ruler swept out by angle A is approximately 5.5 units when in the original frame, but it is contracted to 3.6 units when the observer is going 50% of the speed of light, and to 3.1 units when the observer is going 90% of the speed of light.
The length of ruler swept out by angle B is 2.8 units, but contracted to 2.1 units when the observer is going 50% of the speed of light. However, when you go 90% of the speed of light, yes, there is ONE angle where the apparent length of the ruler is equal to the original length.
But Terrell's statements seem to indicate that he believes the "objects will appear normal" regardless of the angle viewed, which is simply not true.
It appears that this error has indeed stood up to the test of time, because there is no hint in the Wikipedia article on terrell rotation that anyone has ever bothered to correct him. http://en.wikipedia.org/wiki/Penrose-Terrell_rotation.
(My Method)
Consider a ruler lying in the y=1 plane and the z=0 plane. Consider marks on the ruler at points (-2.0, -1.9, -1.8, -1.7, ... 0, 0.1, 0.2, 0.3, 0.4, 0.5, 0.6). (You can imagine the ruler going on forever if you prefer.
Assume your position is x=0,y=0,z=0, and the time is now t=0. (This experiment will take a long time to discuss, but essentially takes zero time to perform.) Assume also that the ruler is aligned with its zero mark at x=0 (with you).
Now, you are observing several "events" on the ruler. Namely, light bounced off or emitted from the ruler sometime in the past, and you are now seeing those events which happened in the past. You can calculate when those events happened by the formula:
[tex]t=-\frac{\sqrt{x^2+y^2}}{c}[/tex]
Now we consider another observer passing through the same location and time (0,0,0,0) but traveling at a speed of 0.8c. The two of you share past light-cones, so all of the events that you are observing, the other observer is observing at the same instant.
However, to find out where he is seeing these events, we must perform a lorentz transformation on each of them.
[tex]\begin{align*} t' &= \gamma t - \beta \gamma x\\ x'&=-\beta \gamma t + \gamma x \\ \end{align*}[/tex]
When this is done, in particular, the ruler marks (-1.5, -1.4, -1.3, -1.2, -1.1, -1.0) are mapped to new positions:
-0.0963, -0.0394, 0.0202, 0.0827, 0.1488, 0.2190
We are particularly interested in the markers -1.4 and -1.2, which now appear at positions -.0394 and .0827. The uncontracted length of the ruler is [tex](-1.2) - (-1.4) = 0.2[/tex], while the apparent length is [tex].0827 - (-.0934)=.1221[/tex] The length contraction factor is [tex].1221/.2=.6105[/tex]
Which is roughly* the same as that which is expected by the lorentz contraction factor [tex]\sqrt{1-0.8^2}= 0.6[/tex]
*If you wanted more fine detail, you should make more marks on the ruler around x=-1.33
I am attaching a couple of xls files, so you can see how I calculated things. File 1: https://www.physicsforums.com/attachment.php?attachmentid=37895&d=1312984992
File 2: https://www.physicsforums.com/attachment.php?attachmentid=37896&d=1312984992
Finally, (In direct conflict with Terrell's conclusiion) the region where to look, so that the effect of lorentz contraction is exactly what would be expected (a meter stick in motion past the observer in such a way that it is moving parallel to its length, and is momentarily seen by the observer at its point of closest approach" WILL BE SEEN AS CONTRACTED:
I recently posted this response to a general question of how Special Relativity worked.
JDoolin said:I'd say you are missing the vital third issue commonly known as the "relativity of simultaneity"
Imagine that you have an open-ceiling circular room ,filled with smoke (to reveal where a flash of light is), and walled with mirrors (to reflect the flash of light), and there is a bright flash of light that emits from the center, passes through the smoke in an expanding circle, bounces off walls (simultaneously), and arrives again simultaneously at the center.
From the point of view of someone hovering directly above the room, it appears that the light hits every part of the mirror simultaneously. However, to someone traveling past at 30% of the speed of light, it should appear as in the animation below.

There are three main differences from the hovering viewpoint and the .3c viewpoint:
(1) The light takes longer to make its outbound and return trip. (time dilation)
(2) The room no longer appears to be circular but slightly oval. (length contraction)
(3) The lignt no longer reaches all parts of the outer circle simultaneously, but instead hits the back end first. (relativity of simultaneity.)
G H Wells Jr pointed out that
ghwellsjr said:You must have some magic smoke that doesn't attenuate the primary signal significantly but still provides a secondary reflection scattered in all directions but still bright enough to be detected far, far away.
and
ghwellsjr said:Isn't it obvious that eventually the angles for viewing each cycle will distort the image so that it doesn't match your animation? Once you see that, you can also see that there is distortion within each cycle right down to the very first one. That's all I'm trying to point out.
Terrell's Argument
Both of these are important issues to take into account. However, they do not change the fact that "a meter stick in motion past the observer in such a way that it is moving parallel to its length, and is momentarily seen by its observer at its point of closest approach, will surely be seen as contracted."
This last quote is directly from an article by James Terrell, in in the 1959 Physical Review "Invisibility of Lorentz Contraction" , but he argues the opposite point: He claims that "the conformality of aberration ensures that, at least over small solid angles, each [co-located observer, regardless of relative motion] will see precisely what the other sees. No Lorentz contractions will be visible, and all objects will appear normal."
Is the Lorentz contraction "invisible" as Terrell claims, or has James Terrell made a mistake which has gone unnoticed for decades?
I'll take some time to analyze Terrell's argument (or lack thereof), and check whether my own methods (analyzing intersections of world-lines and light-cones) agree with his, (transformations of angles via an aberration equation) and if they don't agree, see if I can figure out why.
Terrell finds an aberration equation from the Lorentz transformations, then uses the aberration equation to conclude that the Lorentz contraction effect "vanishes," but I find it suspicious, when by using the Lorentz transformations directly, I find that the Lorentz contraction is quite visible. and when using diagrams from the aberration equation, I still find that the Lorentz contraction is visible.
In fact, even using the aberration equation, it is plain that the objects do not "all appear normal," as Terrell claimed. They definitely have different lengths depending on their relative position, and the relative speed of the observer.
Method Using the Aberration:
I found a nice diagram of the aberration equation here: http://www.mathpages.com/rr/s2-05/2-05.htm which helps me make my point without a lot of math.
It appears to me, that even using the aberration equations, the Lorentz Contraction is visible, and for certain, it cannot be said that "all objects will appear normal" as Terrell claims.
In the attached diagram you can see that the length of the ruler swept out by angle A is approximately 5.5 units when in the original frame, but it is contracted to 3.6 units when the observer is going 50% of the speed of light, and to 3.1 units when the observer is going 90% of the speed of light.
The length of ruler swept out by angle B is 2.8 units, but contracted to 2.1 units when the observer is going 50% of the speed of light. However, when you go 90% of the speed of light, yes, there is ONE angle where the apparent length of the ruler is equal to the original length.
But Terrell's statements seem to indicate that he believes the "objects will appear normal" regardless of the angle viewed, which is simply not true.
It appears that this error has indeed stood up to the test of time, because there is no hint in the Wikipedia article on terrell rotation that anyone has ever bothered to correct him. http://en.wikipedia.org/wiki/Penrose-Terrell_rotation.
(My Method)
JDoolin said:The main axiom I'm using here is that the current apparent position of an object according to an observer, is the positional component (in the observer's current rest frame) of the intersection of the objects world-line (or curve) with the [surface of] the observer's current past light cone.
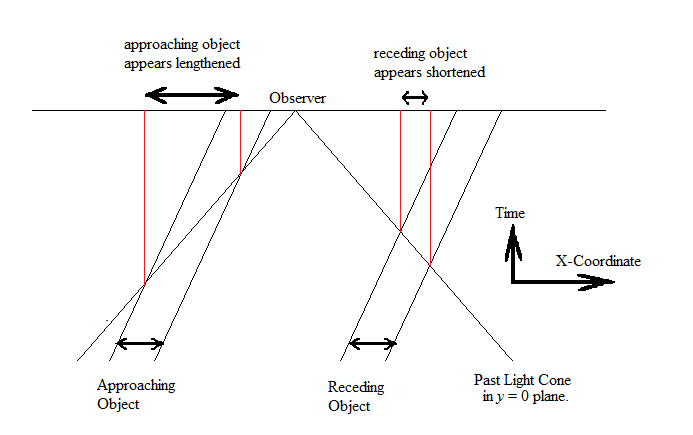
So the attached diagrams show first, a one-dimensional object passing in the y=0 plane. In this plane, the distortion is always present.
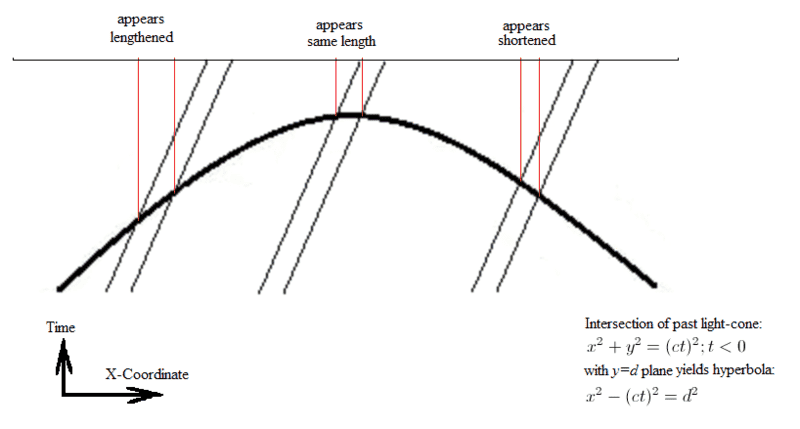
In the second diagram, a one-dimensional object is passing in the y=d plane. In the y=d plane, there is a small region where there is no significant distortion between the "actual" length-contracted shape, and the "apparent" shape.
JDoolin said:Maybe I should clarify that I am talking about the SURFACE of the light cone. I think the point you're missing is that the surface of the past light-cone with its point at (0,0,0,0) is the locus of events which can be detected at the event (0,0,0,0). This fact remains the same, regardless of the observer's reference frame.
I hope you can understand that the observer can see the locus of events on the surface of his or her past light-cone. The tip of the light-cone is the event where the information arrives. It is not a remote event, but THE local event. Naturally, it is also the one event which does not move when you perform a lorentz transformation. All of the other events move, but any event which is in the surface of the past light-cone stays in the surface of the past light-cone.
(If you have any doubt on this, think, how could it be otherwise? How could light that is arriving at an event (0,0,0,0) in one reference frame be NOT arriving at the event (0,0,0,0); the same exact event, in another reference frame? Also you can check the before and after transformation coordinates in my spreadsheet files, to check that indeed t' = -sqrt(x'^2+y'^2).)
Consider a ruler lying in the y=1 plane and the z=0 plane. Consider marks on the ruler at points (-2.0, -1.9, -1.8, -1.7, ... 0, 0.1, 0.2, 0.3, 0.4, 0.5, 0.6). (You can imagine the ruler going on forever if you prefer.
Assume your position is x=0,y=0,z=0, and the time is now t=0. (This experiment will take a long time to discuss, but essentially takes zero time to perform.) Assume also that the ruler is aligned with its zero mark at x=0 (with you).
Now, you are observing several "events" on the ruler. Namely, light bounced off or emitted from the ruler sometime in the past, and you are now seeing those events which happened in the past. You can calculate when those events happened by the formula:
[tex]t=-\frac{\sqrt{x^2+y^2}}{c}[/tex]
Now we consider another observer passing through the same location and time (0,0,0,0) but traveling at a speed of 0.8c. The two of you share past light-cones, so all of the events that you are observing, the other observer is observing at the same instant.
However, to find out where he is seeing these events, we must perform a lorentz transformation on each of them.
[tex]\begin{align*} t' &= \gamma t - \beta \gamma x\\ x'&=-\beta \gamma t + \gamma x \\ \end{align*}[/tex]
When this is done, in particular, the ruler marks (-1.5, -1.4, -1.3, -1.2, -1.1, -1.0) are mapped to new positions:
-0.0963, -0.0394, 0.0202, 0.0827, 0.1488, 0.2190
We are particularly interested in the markers -1.4 and -1.2, which now appear at positions -.0394 and .0827. The uncontracted length of the ruler is [tex](-1.2) - (-1.4) = 0.2[/tex], while the apparent length is [tex].0827 - (-.0934)=.1221[/tex] The length contraction factor is [tex].1221/.2=.6105[/tex]
Which is roughly* the same as that which is expected by the lorentz contraction factor [tex]\sqrt{1-0.8^2}= 0.6[/tex]
*If you wanted more fine detail, you should make more marks on the ruler around x=-1.33
I am attaching a couple of xls files, so you can see how I calculated things. File 1: https://www.physicsforums.com/attachment.php?attachmentid=37895&d=1312984992
File 2: https://www.physicsforums.com/attachment.php?attachmentid=37896&d=1312984992
Finally, (In direct conflict with Terrell's conclusiion) the region where to look, so that the effect of lorentz contraction is exactly what would be expected (a meter stick in motion past the observer in such a way that it is moving parallel to its length, and is momentarily seen by the observer at its point of closest approach" WILL BE SEEN AS CONTRACTED:
JDoolin said:the effect [of speed-of-light delay-times] will at a minimum at a certain angle, but would never competely go away.
You can get it as exact as you want by using events around the point t'=-1, x'=0
[tex]
\begin{pmatrix}
c t'\\ x'
\end{pmatrix}
= \Lambda ^{-1}
\begin{pmatrix}
c t\\ x
\end{pmatrix}
[/tex]
[tex]
=
\begin{pmatrix}
\gamma & \beta \gamma \\
\beta \gamma & \gamma
\end{pmatrix}
\begin{pmatrix}
-1\\ 0 \end{pmatrix} = \begin{pmatrix} -\gamma\\ -\beta \gamma
\end{pmatrix}
[/tex]
Since in this case,
[tex]\begin{matrix} \beta = 0.8 \\ \gamma = \frac{1}{\sqrt{1-.8^2}}=\frac{5}{3}\\ \beta \gamma = \frac{4}{3} \end{matrix}[/tex]
You can use points on the ruler right around x=-4/3, labeled, for instance 1.333 and -1.334, (and the corresponding t values, which you can easily calculate, using [itex]- (ct)^2 = x^2 + y^2[/itex]
) .