Harmonic Definition and 1000 Threads
-
G
I Dispersion: Why are Sine-like Functions Fundamental?
Hi. Light travelling in dispersive media is normally treated by being broken up into its harmonic constituents by Fourier analysis and those then travel at frequency-dependent, but constant speed. However, from a mathematical point of view, there should be infinitely many other bases of the...- greypilgrim
- Thread
- Dispersion Fourier Harmonic
- Replies: 6
- Forum: Optics
-
Energy of particle in simple harmonic motion
Using the above equations for potential and kinetic energy, I figured that potential energy would be twice kinetic energy where ##kx^2=2mv^2##. Solving this equation for v (where ##k=mw^2##), we get ##v=wx/sqrt 2## Counting one complete cycle of the function on a graphing calculator, I found...- Brian_D
- Thread
- Harmonic Particle
- Replies: 13
- Forum: Introductory Physics Homework Help
-
K
Rotating simple harmonic oscillator
If I understand the problem correctly, I need to find the angular frequency of the mass's oscillations about the radius R, which, I think, should be the length of the spring when the mass is merely rotating with angular speed ω (and not oscillating along the radial direction). I was able to find...- kekpillangok
- Thread
- Harmonic Rotating
- Replies: 11
- Forum: Introductory Physics Homework Help
-

Harmonic potential exercise with perturbation theory
Hello there, I'm training with some exercises in view of the July test, so I will post frequently in the hope that someone can help me, since the teacher is often busy and there are no solutions to the exercises. A particle of mass m in one dimension is subject to the potential: ##V(x) =...- keyzan
- Thread
- Harmonic Perturbation Potential
- Replies: 2
- Forum: Advanced Physics Homework Help
-
N
Damped harmonic oscillation
- NTesla
- Thread
- Damped Harmonic Oscillation
- Replies: 64
- Forum: Introductory Physics Homework Help
-
E
Harmonics, Interferences, Autocorrelations, FFT
Hi I have some questions about the following: 1. It is an FFT spectrum analysis output from the Sigview software. First. The large peak at 60Hz is AC interference on air (maybe capacitive coupling) as the amplifier is completely battery operated. How come there are no 120 Hz, 180Hz, 240Hz...- Ephant
- Thread
- Fft Harmonic Interference
- Replies: 7
- Forum: Electrical Engineering
-

Continuity Equation for a Dimensionless Harmonic Oscillator
I've tried to solve this problem (Zettili, Exercise 3.5) four times at this point. I believe my equation for the wave function at a later time ##t## is correct. The problem is my continuity equation is not satisfied; it does not equal zero. It's close but I'm off by a factor of ##m## and...- flyusx
- Thread
- Continuity Harmonic Oscillator
- Replies: 16
- Forum: Advanced Physics Homework Help
-

Where Did I Go Wrong in Proving Harmonic Motion in an Adiabatic Process?
TL;DR Summary: Problem said that the ball moves in a harmonic motion and asked to prove it. The process is adiabatic Problem said that the ball moves in a harmonic motion and asked to prove it. The process is adiabatic. I did the development, but at certain point I'm having a problem. The...- pedrovisk
- Thread
- Adiabatic Harmonic Motion
- Replies: 6
- Forum: Advanced Physics Homework Help
-

B I'm trying to find a general formula for a harmonic(ish) series
Im looking for a general formula for the partial sum of a series where the nth term is in the form of a_n = 1/(c+kn), where c and k are arbitrary constants. it "looks" like a harmonic series but not in the form I'm capable of figuring out. help.- al4n
- Thread
- Formula General Harmonic
- Replies: 8
- Forum: General Math
-

Solve Disk & Coil Spring Motion: Get Amplitude Help
I was able to solve part 1 but I am not not getting how to find new amplitude of the motion . Please help me- Lil123
- Thread
- Harmonic Motion Physics
- Replies: 7
- Forum: Introductory Physics Homework Help
-

Solve First Year Harmonic Motion Problem: Cylinder Rolling
Thank you guys for taking the time to read this - I'm decently struggling with first year and need some tips on how to properly conceptualize problems and learn what the right approach is on certain problems. Have a wonderful day, again thank you for checking this post out!- Xiothus
- Thread
- Cylinder First year Fundamentals Harmonic Harmonic motion Motion Need guidance Rolling Spring Surface Year
- Replies: 5
- Forum: Introductory Physics Homework Help
-

I Translating the harmonic oscillator
Let's say I know the position space wavefunctions of the 1d harmonic oscillator ##\psi_n(x)## corresponding to the state ##| n \rangle## are known. I want to write ##\psi_m(x + a)##, for fixed ##m = 1,2,...##, in terms of all of the ##\psi_n(x)##. I know \begin{align*} \psi_n(x+a) = \langle x |...- ergospherical
- Thread
- Harmonic Harmonic oscillator Oscillator
- Replies: 2
- Forum: Quantum Physics
-
P
I Thoughts about coupled harmonic oscillator system
Same instruction was given while finding value of 'g' by a bar pendulum. In the former case,does the spring obeys hooke's law while it forms a coupled harmonic oscillator system?Does the bar pendulum somehow breaks the simple harmonic motion(such that we can't apply the law for sumple harmonic...- phymath7
- Thread
- Coupled Coupled oscillations Harmonic Harmonic oscillator Hooke's law Oscillator Simple hamonic motion System Thoughts
- Replies: 1
- Forum: Classical Physics
-

B How is the harmonic mean affected by additional data points?
We have a collection of 8 discrete data points. They are: 10, 20, 30, 20, 30, 40, 30, 40 In increasing order: 10, 20*2, 30*3, 40*2 The harmonic mean of this data series is 22.86 I read on Wikipedia that the harmonic mean is skewed towards the smaller values i.e. smaller values will affect the...- Feynstein100
- Thread
- Data Harmonic Mean Points
- Replies: 4
- Forum: General Math
-

Superposition of two one-dimensional harmonic waves
##\mathbf {Homework ~Statement:}## Consider the superposition of two one-dimensional harmonic waves $$s_1(x,t)=3.5 cm \cdot cos(27.5s^{-1} \cdot t - 5.65m^{-1} \cdot x)$$ $$s_2(x,t)=3.5 cm \cdot cos(27.5s^{-1} \cdot t - 5.5m^{-1} \cdot x)$$ ##\mathbf {a)}## Calculate the wavelength ##\lambda##...- orangephysik
- Thread
- Harmonic Superposition Waves
- Replies: 10
- Forum: Introductory Physics Homework Help
-

Damped harmonic oscillation of a swingboat
Hi, so of course Φ0 = 15° and after solving after solving Φ(t=5*T = 5/f) I found γ = 0.012 I need help with b). If I do 2° = 15° * exp(-0.012t)*cos(2πf*t), I'm not able to find t so I did something else by assuming that the amplitude decreases at a constant rate: After 5*T = 5*1/f = 18.52 s...- orangephysik
- Thread
- Damped Harmonic Harmonic oscillation Oscillation
- Replies: 6
- Forum: Introductory Physics Homework Help
-
V
How to prove that motion is periodic but not simple harmonic?
TL;DR Summary: Prove that a sum of trigonometric ratios is periodic but not not simple harmonic. We need to prove that ##x = sin{\omega t} + sin{2\omega t} + sin{4\omega t}## where ##x## is the displacement from the equilibrium position at time ##t##. I can see that each term is a SHM, but...- vcsharp2003
- Thread
- Harmonic Motion Periodic Periodic motion Simple harmonic motion
- Replies: 51
- Forum: Introductory Physics Homework Help
-
Z
Solving for Simple Harmonic Motion: A Picture Problem
Here is a picture of the problem It is not clear to me how to really prove that the equation for ##\theta(t)## is simple harmonic motion, and what the period of this motion is.- zenterix
- Thread
- Electric dipole Harmonic Harmonic motion Motion Picture Simple harmonic motion Torque
- Replies: 1
- Forum: Introductory Physics Homework Help
-

Bungee jump | simple harmonic motion
As you all know, a bungee jump is where a person is tied to a cord and the person jumps off and bounces up again. The natural length of a cord is 75 metres. Then when a person is attached onto the cord, the length becomes 83 metres when the person is at rest. I am sure that the person is not...- DarkEnergy890
- Thread
- Bungee Bungee jump Harmonic Harmonic motion Jump Motion Simple harmonic motion
- Replies: 5
- Forum: Introductory Physics Homework Help
-
J
Modification to the simple harmonic oscillator
I was assuming there could be something via perturbation theory? I am unsure.- jamesonWHIS
- Thread
- Harmonic Harmonic oscillator Oscillator Simple harmonic oscillator
- Replies: 2
- Forum: Advanced Physics Homework Help
-

Study of harmonic motion of a liquid in a V shaped tube
A V-shaped tube with a cross-section A contains a perfect liquid with mass density and length L plus and the angles between the horizontal plane and the tube arms as shown in the attached figure. We displace the liquid from its equilibrium position with a distance and without any initial...- sumatoken
- Thread
- Harmonic Harmonic motion Lagrange Liquid Motion Physics Study Tube Vibration
- Replies: 2
- Forum: Introductory Physics Homework Help
-
G
I Can the Inverse Tan Function Justify Phase Lag in a Driven Harmonic Oscillator?
This is an equation I found for the delta phase lag of a driven oscillator. W is the driving angular frequency and Wo is the natural angular frequency of the driven system. Of course this is just a small part of the solution to the differential equation. Now ... 1) when W is much smaller than Wo... -
P
X^4 perturbative energy eigenvalues for harmonic oscillator
The book(Schaum) says the above is the solution but after two hours of tedious checking and rechecking I get 2n^2 in place or the 3n^2. Am I missing something or is this just a typo?- PBTR3
- Thread
- Eigenvalues Energy Harmonic Harmonic oscillator Oscillator
- Replies: 6
- Forum: Advanced Physics Homework Help
-
S
I How to interpret complex solutions to simple harmonic oscillator?
Consider the equation of motion for a simple harmonic oscillator: ##m\ddot {x}(t)=-kx(t).## The solutions are ##x(t)=Ae^{i\omega t}+Be^{-i\omega t},## where ##\omega=\sqrt{\frac{k}{m}}##, and constants ##A## and ##B##. Physically, what does it mean for a solution to be complex? Is it only the... -
A
I The speed of a waves on a string in Simple harmonic motion
The speed of a wave in simple harmonic motion on a string is $$v= \sqrt{\frac{F}{\mu}}$$ where v= the horizontal velocity of the wave on a string. Is the F the horizontal force or the resultant force (combination of Fy and Fx)? -

B Is simple harmonic motion also a pure translatory motion?
Is simple harmonic motion also a pure translatory motion?"A rigid body moves in pure translation if each particle of the body undergoes the same displacement as every other particle in any given time interval" [Halliday and Resnick, Physics].If not,then how does shm deviate from this definition> -

B Harmonic oscillator and simple pendulum time period
Hi, I have been thinking about pendulums a bit and discovered that a HO(harmonic Oscillator) will take the same time to complete one period T no matter which amplitude A/length l it has, if stiffness k and mass m are the same. But moving on to a simple pendulum suddenly the time period for one...- Misha87
- Thread
- Harmonic Harmonic oscillator Oscillator Pendulum Period Simple harmonic oscillator Simple pendulum Time Time period
- Replies: 36
- Forum: Classical Physics
-

I Doubt on Morse potential and harmonic oscillator
I have a little doubt about Morse potential used for vibration levels of diatomic molecules. With regard to the image below, if the diatomic molecule is in the vibrational ground state, when the oscillation reaches the maximum amplitude for that state the velocity of the molecule must be zero so...- Salmone
- Thread
- Doubt Harmonic Harmonic oscillator Oscillator Potential
- Replies: 2
- Forum: Quantum Physics
-
M
I Constructive interference of harmonic electromagnetic waves
Hello ! I have a doubt as to how is this case, if it occurs, of the constructive interference of two harmonic electromagnetic waves but of different wavelengths or frequencies between them. That is, if between the two electromagnetic waves a new and unique electromagnetic wave is created and... -

Question on Intro QM pertaining to Harmonic Oscillator
Hi. I have attached a neatly done solution to the above question. I request someone to please check my solution and help me rectify any possible mistakes that I may have made.- warhammer
- Thread
- Harmonic Harmonic oscillator Intro Oscillator Qm
- Replies: 2
- Forum: Introductory Physics Homework Help
-
W
Transformer modelling for harmonic studies
Why transformer core is modeled as resistance even though it is frequency dependent.With increasing frequency current rises. Doesn't it seem capacitive reactance behaviour? Which decrease with increasing omega?- waqasakbar323
- Thread
- Harmonic Modelling Studies Transformer
- Replies: 7
- Forum: Electrical Engineering
-
K
Proving that a "composition" is harmonic
I am learning some complex analysis as it is a prerequisite for the masters program that I was accepted into and I didn't take it yet during my bachelors. I am using some lecture notes in Slovene and I have run into a problem that has proven troublesome for me : If ##g: D \rightarrow \mathbb{C}...- kmitza
- Thread
- Complex analysis Composition Harmonic
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
W
Modelling of tranformer and MV cable for harmonic studies
For my research project, modelling is needed for dyn5 630 kVA transformer and 20 kV cable NA2XS(F)2Y to do harmonic analysis. For that i need leakage reactance, and resistance of copper losses for transformer. And following parameters for 20 kV cable are also needed. Positive, negative and...- waqasakbar323
- Thread
- Cable Harmonic Modelling Studies
- Replies: 11
- Forum: Electrical Engineering
-

I Particle on a cylinder with harmonic oscillator along z-axis
I need to know if I have solved the following problem well: A spin-less particle of mass m is confined to move on the surface of a cylinder of infinite height with a harmonic potential on the z-axis and Hamiltonian ##H=\frac{p_z^2}{2m}+\frac{L_z^2}{2mR^2}+\frac{1}{2}m\omega^2z^2## and I need to...- Salmone
- Thread
- Cylinder Harmonic Harmonic oscillator Oscillator Particle Quantum mechanics
- Replies: 2
- Forum: Quantum Physics
-

Quantum Harmonic Oscillator with Additional Potential
Hello there, I am trying to solve the above and I'm thinking that the solutions will be Hermite polynomials multiplied by a decaying exponential, much like the standard harmonic oscillator problem. The new Hamiltonian would be like so: $$H = - \frac \hbar {2m} \frac {d^2}{dx^2}\psi + \frac...- Mr_Allod
- Thread
- Harmonic Harmonic oscillator Oscillator Potential Quantu physics Quantum Quantum harmonic oscillator
- Replies: 1
- Forum: Advanced Physics Homework Help
-

B Why is a simple pendulum not a perfect simple harmonic oscillator?
Khan Academy claims that a simple pendulum not a perfect simple harmonic oscillator. Why is it so?- Huzaifa
- Thread
- Harmonic Harmonic oscillator Oscillator Pendulum Simple harmonic oscillator Simple pendulum
- Replies: 4
- Forum: Classical Physics
-

Finding Spring Constant When Given Amplitude, Time, and Mass
I averaged the masses and times (which i took the time given and divided by 10 because in the problem it says you measure the time it takes to complete 10 oscillations) then plugged them directly into the T=(2(pi)((m/k)^1/2) and got the wrong answer. This is really confusing me because I don't...- physicsneedslabs
- Thread
- Amplitude Constant Harmonic Mass Motion Spring Spring constant Time
- Replies: 9
- Forum: Introductory Physics Homework Help
-
L
An object oscillating in simple harmonic motion
Since it passes through the origin every ##3.6s## the period is ##T=3.6s## hence ##\omega=\frac{2\pi}{\omega}=\frac{2\pi}{3.6}\frac{rad}{s}## thus ##A=\frac{v_{max}}{\omega}=\frac{1.2}{\frac{2\pi}{3.6}}m\simeq 0.69m## and ##a_{max}=\omega^2 A=(\frac{2\pi}{T})^2 A=(\frac{2\pi}{3.6})^2 \cdot...- lorenz0
- Thread
- Harmonic Harmonic motion Motion Oscillating Simple harmonic motion
- Replies: 6
- Forum: Introductory Physics Homework Help
-
J
Discretizing a 1D quantum harmonic oscillator, finding eigenvalues
##x## can be discretized as ##x \rightarrow x_k ## such that ##x_{k + 1} = x_k + dx## with a positive integer ##k##. Throughout we may assume that ##dx## is finite, albeit tiny. By applying the Taylor expansion of the wavefunction ##\psi_n(x_{k+1})## and ##\psi_n(x_{k-1})##, we can quickly...- JTFreitas
- Thread
- 1d Eigenvalues Harmonic Harmonic oscillator Oscillator Quantum Quantum harmonic oscillator
- Replies: 5
- Forum: Advanced Physics Homework Help
-

Comp Sci Harmonic Amplitudes - 3rd & 101st
I have attempted the question using the large formula here with figures from the waveform with n as 3 & 101: however I think that the large formula is for the total waveform encompassing all harmonics as the 3rd and 101st harmonic amplitudes are very similar 10.9662222 *10^-6V for the 3rd...- Jason-Li
- Thread
- Amplitudes Electrical Harmonic Harmonics Physics
- Replies: 13
- Forum: Engineering and Comp Sci Homework Help
-

Square Wave Magnetic Pulses Using Harmonic Addition
- Narayanan KR
- Thread
- Addition Electromagetic field Harmonic Harmonic analysis Harmonic frequency Magnetic Square Square wave Wave
- Replies: 24
- Forum: Electrical Engineering
-

B Arithmetic progression, Geometric progression and Harmonic progression
How do I build functions by using Arithmetic Sequence, Geometric Sequence, Harmonic Sequence? Is it possible to create all the possible function by using these sequences? Thanks!- pairofstrings
- Thread
- Arithmetic Arithmetic progression Functions Geometric Harmonic
- Replies: 5
- Forum: General Math
-

MHB Understanding the Harmonic Function Problem
Please help me I am struggle with this question Thank you in advance- jaychay
- Thread
- Function Harmonic
- Replies: 2
- Forum: Topology and Analysis
-

MHB How to Solve a Harmonic Function Problem Involving Partial Derivatives?
Can you please help me how to do it ? I am really struggle with this question. Thank you in advance- jaychay
- Thread
- Function Harmonic
- Replies: 3
- Forum: Topology and Analysis
-
R
Weakly interacting Bosons in a 3D harmonic oscillator
1. Since N is large, ignore the kinetic energy term. ##[-\mu + V(r) + U|\Psi (r)|^2]\Psi (r) = 0## 2. Solve for the density ##|\Psi (r)|^2## ##|\Psi (r)|^2 = \frac{\mu - V(r)}{U}## 3. Integrate density times volume to get number of bosons ##\int|\Psi (r)|^2 d\tau = \int \frac{\mu -...- rmiller70015
- Thread
- 3d Bosons Harmonic Harmonic oscillator Oscillator
- Replies: 4
- Forum: Advanced Physics Homework Help
-
When Does the Amplitude Drop to 1/5 in Damped Harmonic Motion?
Hi ! Problem : y = 5 e^-0.25t sin (0.5.t) (m, s). Determine the deviation at a time when the amplitude has dropped to 1/5 of the original value. I tried with A=A0 e^-bt=5 e^-0.25t - Do i need to determine the time here or recreate the deviation equation when A decreased ? I don't understand...- meher4real
- Thread
- Damped Damped harmonic motion Harmonic Harmonic motion Motion
- Replies: 11
- Forum: Introductory Physics Homework Help
-

Superposition of two simple harmonic motion
Hey! I am stuck in this problem, i don't know how to sum this ecuations. I remember that its possible because the direction is the same So, i try to sum like this: cos (t+5325) + 1.5 cos (t+5325) =1.5 cos (t+5325) I don't know if i fine. I thanks your help, please ;)- Krokodrile
- Thread
- Harmonic Harmonic motion Motion Simple harmonic motion Superposition
- Replies: 4
- Forum: Introductory Physics Homework Help
-

Find Ground State Energy of 3D Harmonic Oscillator
Summary:: I am trying to find the exact ground state energy of the hamiltonian.kindly help me with this- koustav
- Thread
- 3d Harmonic Harmonic oscillator Oscillator
- Replies: 4
- Forum: Advanced Physics Homework Help
-

Is Your Calculation of Simple Harmonic Motion Accurate?
The first ecuation values i am 99% that is correct. But, in the second and three problem i don't know if my results are ok. The problem number 2 i comprobate with the teacher that te aceleration its correct, so, with this i calculate the velocity. I use like example the second problem for try...- Krokodrile
- Thread
- Harmonic Harmonic motion Motion Movement Simple harmonic motion
- Replies: 5
- Forum: Introductory Physics Homework Help
-
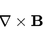
Density of states of one three-dimensional classical harmonic oscillator
ia- anaisabel
- Thread
- Classical Density Density of states Harmonic Harmonic oscillator Oscillator States Statisical mechanics
- Replies: 11
- Forum: Advanced Physics Homework Help