Oscillator Definition and 1000 Threads

Oscillation is the repetitive variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. The term vibration is precisely used to describe mechanical oscillation. Familiar examples of oscillation include a swinging pendulum and alternating current.
Oscillations occur not only in mechanical systems but also in dynamic systems in virtually every area of science: for example the beating of the human heart (for circulation), business cycles in economics, predator–prey population cycles in ecology, geothermal geysers in geology, vibration of strings in guitar and other string instruments, periodic firing of nerve cells in the brain, and the periodic swelling of Cepheid variable stars in astronomy.

View More On Wikipedia.org
Recent contents Top users
  1. DaveE

    I Is the Earth-Sun system an oscillator? What is an oscillator?

    I thinking of high Q oscillator examples* and pondered planetary orbits. The oscillators I am familiar with all shift energy either from place to place or different forms (mass-spring, L-C, E-B, etc.). But a planet in orbit (let's say circular) has potential and kinetic energy that never really...
  2. J

    B Why does my LC circuit not oscillate its energy between Electric & Magnetic fields?

    My inductor has 8 turns, 4 cm diameter and 4 cm length. The capacitor I use is a 1 microfarad polyester capacitor. When the copper wire inductor is connected to DC voltage the compass needle is deflected by 90 degrees and the multimeter detects 1 A or 1000 mA of current. When DC is switched...
  3. B

    Textbook 'The Physics of Waves': Linearity of Forced Oscillator

    Reference textbook “The Physics of Waves” in MIT website: https://ocw.mit.edu/courses/8-03sc-...es-fall-2016/resources/mit8_03scf16_textbook/ Chapter 2 - Problem 2.2 [Page 51] (see attached file) Question: In the content of Page 43 (see attached file), it also states that the amplitude of...
  4. M

    Coupled oscillator question

    The problem and solution is, However, I am confused how they get ##\vec a = (1, 2)## (I convert from column vector to coordinate form of vector). I got ##\vec a = (a_1, a_2) = (a_1, 2a_1) = a_1(1, 2)## however, why did they eliminate the constant ##a_1##? Thanks for any help!
  5. flyusx

    Continuity Equation for a Dimensionless Harmonic Oscillator

    I've tried to solve this problem (Zettili, Exercise 3.5) four times at this point. I believe my equation for the wave function at a later time ##t## is correct. The problem is my continuity equation is not satisfied; it does not equal zero. It's close but I'm off by a factor of ##m## and...
  6. A

    B Amplifier or oscillator for a vibration generator

    I purchased this vibration generator to construct a Chladni plate. I was hoping to use it with my existing oscilloscope (which appears to be very old). It seems the oscilloscope is not giving me a signal strong enough to drive the generator. Any suggestions for a different amplifier or power...
  7. N

    Oscillation with friction - Analytical mechanics

    Hi, I had those exercises and want to know if they're correct. Also, feedback/tips would be great from you, professionals. $$A$$ 1. Let's consider the oscillator with a friction parameter... \begin{equation} m \ddot{x}+\alpha \dot{x}=-\kappa x \end{equation} but with \begin{equation}...
  8. DifferentialGalois

    Oscillator Differentials: What's a physical meaning of complex part of the solution for coordinate change of the anharmonic oscillator?

    I don't understand what the question means, and the answer is provided here: https://physics.stackexchange.com/a/35821/222321 Could someone provide a comprehensive one-by-one explanation.
  9. TheAmatuerHobbyist

    Electrical Electromagnetic confinement and Oscillators

    I am wondering if it is possible to use two electromagnets oscillating at about 1 ghz to suspend an amount of ferrofluid in an acrylic chamber. As I understand it, 1 ghz should be sufficient enough to get the ferrofluid away from each magnet, as after a quick google search, magnetic fields move...
  10. MatinSAR

    Analyse motion of an oscillator: x(t)=0.2cos(12*pi*t)

    Hello. I have tried to solve it using x-t Graph. We know that period of this function is ##T=\frac {1}{6}s##. Then I've used ##x(t)=0## to find the times in which the oscillator is at ##x=0##: ##t=\frac {k}{12} + \frac {1}{24}## for ## k \in Z.## Now I can draw x-t graph. We should check time...
  11. kornelthefirst

    What Is the Next Step in Simplifying the Van der Pol Oscillator Equation?

    First i looked at the case of ## \epsilon = 0## and came to the conclusion, that this oscillator has a circular limit cycle in a phase space trajectory, when plotted with the axes x and ##\dot{x}##. I have found that ##x_0^p (t) = a_1 \cos(t)## which implies that all other Fourier- coefficients...
  12. ergospherical

    I Translating the harmonic oscillator

    Let's say I know the position space wavefunctions of the 1d harmonic oscillator ##\psi_n(x)## corresponding to the state ##| n \rangle## are known. I want to write ##\psi_m(x + a)##, for fixed ##m = 1,2,...##, in terms of all of the ##\psi_n(x)##. I know \begin{align*} \psi_n(x+a) = \langle x |...
  13. P

    I Thoughts about coupled harmonic oscillator system

    Same instruction was given while finding value of 'g' by a bar pendulum. In the former case,does the spring obeys hooke's law while it forms a coupled harmonic oscillator system?Does the bar pendulum somehow breaks the simple harmonic motion(such that we can't apply the law for sumple harmonic...
  14. Z

    What is the oscillator model in a generalized Snyder scheme？

    What is the oscillator model in a generalized Snyder scheme？How to derive the formula？
  15. M

    Why is φ Assumed to Be 90 in the Undamped Forced Oscillator Solution?

    For this problem, The solution is, However, can someone please explain how this is showing equation 15.35 as a solution of equation 15.34? I though both sides should be equal without assuming that ##\phi = 90## Also why are they allowed to assume ##\phi = 90##? Many thanks!
  16. J

    Modification to the simple harmonic oscillator

    I was assuming there could be something via perturbation theory? I am unsure.
  17. G

    I Can the Inverse Tan Function Justify Phase Lag in a Driven Harmonic Oscillator?

    This is an equation I found for the delta phase lag of a driven oscillator. W is the driving angular frequency and Wo is the natural angular frequency of the driven system. Of course this is just a small part of the solution to the differential equation. Now ... 1) when W is much smaller than Wo...
  18. P

    X^4 perturbative energy eigenvalues for harmonic oscillator

    The book(Schaum) says the above is the solution but after two hours of tedious checking and rechecking I get 2n^2 in place or the 3n^2. Am I missing something or is this just a typo?
  19. S

    I How to interpret complex solutions to simple harmonic oscillator?

    Consider the equation of motion for a simple harmonic oscillator: ##m\ddot {x}(t)=-kx(t).## The solutions are ##x(t)=Ae^{i\omega t}+Be^{-i\omega t},## where ##\omega=\sqrt{\frac{k}{m}}##, and constants ##A## and ##B##. Physically, what does it mean for a solution to be complex? Is it only the...
  20. Misha87

    B Harmonic oscillator and simple pendulum time period

    Hi, I have been thinking about pendulums a bit and discovered that a HO(harmonic Oscillator) will take the same time to complete one period T no matter which amplitude A/length l it has, if stiffness k and mass m are the same. But moving on to a simple pendulum suddenly the time period for one...
  21. Salmone

    I Doubt on Morse potential and harmonic oscillator

    I have a little doubt about Morse potential used for vibration levels of diatomic molecules. With regard to the image below, if the diatomic molecule is in the vibrational ground state, when the oscillation reaches the maximum amplitude for that state the velocity of the molecule must be zero so...
  22. Twigg

    Cost/Benefits of an RF Cavity vs Quartz Oscillator

    I just encountered an RF cavity in the wild for the first time. It was used as the frequency reference in the Agilent 8640B RF signal generator, which I believe dates back to the 70's. Were quartz oscillators not an option back then? Or were they worse in stability back then? I'm curious about...
  23. warhammer

    Question on Intro QM pertaining to Harmonic Oscillator

    Hi. I have attached a neatly done solution to the above question. I request someone to please check my solution and help me rectify any possible mistakes that I may have made.
  24. Rezex124

    I Damped oscillator with changing mass

    Hello, So about two weeks ago in class we looked at RLC circuits in our E&M course, and short story short... we compared the exchange of energy between the Capacitor and the Inductor (both ideal) to simple harmonic motion. Once the capacitor and inductor are not ideal anymore, we said it's...
  25. Salmone

    I Particle on a cylinder with harmonic oscillator along z-axis

    I need to know if I have solved the following problem well: A spin-less particle of mass m is confined to move on the surface of a cylinder of infinite height with a harmonic potential on the z-axis and Hamiltonian ##H=\frac{p_z^2}{2m}+\frac{L_z^2}{2mR^2}+\frac{1}{2}m\omega^2z^2## and I need to...
  26. Mr_Allod

    Position expectation value of 2D harmonic oscillator in magnetic field

    Hello there, for the above problem the wavefunctions can be shown to be: $$\psi_{n,l}=\left[ \frac {b}{2\pi l_b^2} \frac{n!}{2^l(n+l)!}\right]^{\frac12} \exp{(-il\theta - \frac {r^2\sqrt{b}}{4l_b^2})} \left( \frac {r\sqrt{b}}{l_b}\right)^lL_n^l(\frac {r^2b}{4l_b^2})$$ Here ##b = \sqrt{1 +...
  27. Mr_Allod

    Quantum Harmonic Oscillator with Additional Potential

    Hello there, I am trying to solve the above and I'm thinking that the solutions will be Hermite polynomials multiplied by a decaying exponential, much like the standard harmonic oscillator problem. The new Hamiltonian would be like so: $$H = - \frac \hbar {2m} \frac {d^2}{dx^2}\psi + \frac...
  28. D

    Need help with spring mass oscillator and its period

    I thought I would multiply b to the whole equation of T, but I have no idea how to formulate into the type of solution it wants
  29. VVS2000

    I Block sliding down an incline plane

  30. Huzaifa

    B Why is a simple pendulum not a perfect simple harmonic oscillator?

    Khan Academy claims that a simple pendulum not a perfect simple harmonic oscillator. Why is it so?
  31. J

    Discretizing a 1D quantum harmonic oscillator, finding eigenvalues

    ##x## can be discretized as ##x \rightarrow x_k ## such that ##x_{k + 1} = x_k + dx## with a positive integer ##k##. Throughout we may assume that ##dx## is finite, albeit tiny. By applying the Taylor expansion of the wavefunction ##\psi_n(x_{k+1})## and ##\psi_n(x_{k-1})##, we can quickly...
  32. R

    Why Are My Coupled Oscillator Eigenvalues Incorrect?

    Hi, I have to find the eigenvalues and eigenvectors for a system of 3 masses and 4 springs. At the end I don't get the right eigenvalues, but honestly I don't know why. Everything seems fine for me. I spent the day to look where is my error, but I really don't know. ##m_a = m_b = m_c## I got...
  33. R

    Driven oscillator amplitude steady state X(t) = ##Asin(\omega t + \delta)##

    I found ## \frac{\gamma}{2} = 7##, ##\gamma = 14## ##\omega_0^2 = \omega_d^2 + \frac{\gamma^2}{4} = 25## ##\omega_0 = \omega = 25##, thus ##\delta = \frac{\pi}{2}## ##A = \frac{\frac{F_0}{m}}{\sqrt((\omega_0^2 - \omega^2)+ \gamma^2\omega^2)} = 0.04## Thus, ##X(t) = 0.04sin(25t + \frac{\pi}{3} -...
  34. vel

    Energy loss of damped oscillator

    yeah, I don't even know how to start
  35. A

    Inductor Efficiency via LC Oscillator

    I want to create an LC circuit with varying inductors and compare those inductors for efficiency. Would it be accurate to suggest measuring the area under the curve of the first cycle of the resonant frequency would determine which of the inductors are most efficient? If the area is greater...
  36. C

    When to have only voltage gain in an oscillator

    What I already know In general, power gain is desirable for an oscillator in order to make up for the losses and then feedback that gain (amplified signal) into the oscillator for it to keep oscillating. Voltage gain is not generally used for oscillators. What I want to know Since power gain is...
  37. C

    Purely passive RC Phase Shift Oscillator?

    It is possible to build a purely passive RC phase shift oscillator with 2 separate (in the future) RC stages like this? Where there would be 2 RC networks that each provide 180 degrees of phase shift. Of course, there would be a buffer in between the 2 RC networks so that they behave as 2...
  38. hilbert2

    A Anharmonic oscillators with closed-form solutions

    There are some articles from the 1980s where the authors discuss 1D quantum oscillators where ##V(x)## has higher than quadratic terms in it but an exact solution can still be found. One example is in this link: https://iopscience.iop.org/article/10.1088/0305-4470/14/9/001 Has anyone tried to...
  39. C

    RC Phase Shift Oscillator not Oscillating

    Hello everyone. I am having some trouble with an RC phase shift oscillator that I built as a hobby project. I am completely stuck on this and I just cannot figure it out. My oscillator is not oscillating. Here is the circuit that I am trying to get to work. Taken from...
  40. R

    Weakly interacting Bosons in a 3D harmonic oscillator

    1. Since N is large, ignore the kinetic energy term. ##[-\mu + V(r) + U|\Psi (r)|^2]\Psi (r) = 0## 2. Solve for the density ##|\Psi (r)|^2## ##|\Psi (r)|^2 = \frac{\mu - V(r)}{U}## 3. Integrate density times volume to get number of bosons ##\int|\Psi (r)|^2 d\tau = \int \frac{\mu -...
  41. M

    Nonlinear spring made from many different linear springs in series?

    Can I create a nonlinear spring, for a nonlinear oscillator, by putting many linear springs in series and parallel?
  42. koustav

    Find Ground State Energy of 3D Harmonic Oscillator

    Summary:: I am trying to find the exact ground state energy of the hamiltonian.kindly help me with this
  43. H

    Is My Thinking Reasonable: A's Impact on Oscillator Motion

    I just want to check if my thinking is reasonable. Since B,C and D are all dependent or fixed by the oscillator, A is the only factor to affect the amplitude of the motion at resonance or even throughout the entire process?
  44. B

    How to solve a differential equation for a mass-spring oscillator?

    There is an mass-spring oscillator made of a spring with stiffness k and a block of mass m. The block is affected by a friction given by the equation: $$F_f = -k_f N tanh(\frac{v}{v_c})$$ ##k_f## - friction coefficient N - normal force ##v_c## - velocity tolerance. At the time ##t=0s##...
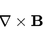
  45. anaisabel

    Density of states of one three-dimensional classical harmonic oscillator

    ia
  46. K

    I Stimulated emission in harmonic oscillator

    Hello! Is stimulated emission possible for a harmonic oscillator (HO) i.e. you send a quanta of light at the right energy, and you end up with 2 quantas and the HO one energy level lower (as you would have in a 2 level system, like an atom)?
  47. S

    I How to solve 2nd order TDSE for a Gaussian-kicked harmonic oscillator?

    Consider the gaussian kick potential, ##\hat{V}(t) = \hat{x} \exp{(\frac{-t^2}{2 \tau^2})}## where ##\hat{x} = a+a^\dagger## in terms of creation and annihilation operators. Then we define the potential in the interaction picture, ##\hat{V}_I(t) = e^{i\hat{H}t}\hat{V}(t)e^{-i\hat{H}t}## I...
  48. T

    I Question on Harmonic Oscillator Series Derivation

    Good afternoon all, On page 51 of David Griffith's 'Introduction to Quantum Mechanics', 2nd ed., there's a discussion involving the alternate method to getting at the energy levels of the harmonic oscillator. I'm filling in all the steps between the equations on my own, and I have a question...
  49. D

    How Does Superposition Affect Measurements in a 1-D Harmonic Oscillator?

    Consider a one-dimensional harmonic oscillator. ##\psi_0(x)## and ##\psi_1(x)## are the normalized ground state and the first excited states. \begin{equation} \psi_0(x)=\Big(\frac{m\omega}{\pi\hbar}\Big)^{\frac{1}{4}}e^{\frac{-m\omega}{2\hbar}x^2} \end{equation} \begin{equation}...
  50. Hamiltonian

    I Solving and manipulating the damped oscillator differential equation

    the differential equation that describes a damped Harmonic oscillator is: $$\ddot x + 2\gamma \dot x + {\omega}^2x = 0$$ where ##\gamma## and ##\omega## are constants. we can solve this homogeneous linear differential equation by guessing ##x(t) = Ae^{\alpha t}## from which we get the condition...
Back
Top